Prisms

A prism is an optical component that serves one of two major functions: it disperses light, or it modifies the direction (and sometimes polarization) of light (1). In some cases, a prism has more than one function. Prisms are usually transparent to the region of the electromagnetic spectrum being observed. Most people are familiar with transparent prisms that are used in the visible region of the spectrum.

David W. Ball

Dispersing Prisms

As early as the 13th century, six-sided crystals of natural quartz were used to generate rainbows (2). The six sides complicated matters, however, making it difficult for experimenters to figure out what was going on. The prevailing view at the time was that the prism was adding color to the white light, which was the "pure" form of light. By the middle of the 16th century, high-quality triangular prisms were available (coincident with the rise of the Venetian glass trade), but scientists at the time still thought that the glass added color to white light.

A prism disperses light (that is, it spatially separates light by wavelength) if the light goes from one medium (say, the air) into another medium (say, a glass prism) at an angle other than 90°. The classic triangular prism has three nonparallel surfaces, so even if light passes through one surface at a 90° angle, it will pass through a second surface at a non-normal angle.

Between 1666 and 1672, Isaac Newton performed his seminal experiments on white light with prisms. He was able to demonstrate conclusively that white light was in fact a composite of all colors, and that the prism itself only separated the colors. Newton was taking advantage of the fact that a prism disperses light; that is, it separates a range of wavelengths into its component wavelengths. A prism disperses as a result of two issues. First is Snell's law (3), which relates the angle of incidence θ_i to the angle of refraction θ_r for light impinging on a surface at some angle to the index of refraction of the two media involved:

n_i·sinθ_i = n_r·sinθ_r

where n_i and n_r are the indices of refraction of the media for the incoming light and the refracted light. The index of refraction is simply the ratio of the velocity of light in a vacuum c and the velocity of light in a given material v_j:

n_j = c / v_j

As such, the slower light is when passing through a nonvacuum, the higher its index of refraction.

The second issue is the fact that the index of refraction of the prism material is not constant; rather, it varies with wavelength. As such, the amount of refraction is different for every wavelength of light, and so a prism acts to spatially separate the different wavelengths (that is, colors) of light. If the light going into the prism is a narrow image, the separation will be visible not far from the prism, before the light has gotten too dim (Figure 1).

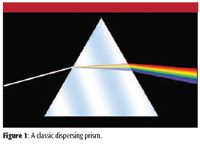
Figure 1

Note in Figure 1 that the higher-energy (blue) light is refracted more than the lower-energy (red) light, implying that the index of refraction for blue light is higher than the index of refraction of red light — such is the general trend for most transparent materials. This property is referred to as dispersion, and a dispersion curve is a plot of the refractive index versus wavelength (Figure 2). If dispersion did not exist, prisms would not separate the different wavelengths of light. (On the other hand, because of dispersion, certain optical components like lenses will separate differing wavelengths of light and direct the light rays in slightly different directions. This effect is usually undesirable and is called chromatic aberration.)

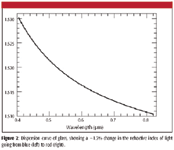
Figure 2

Prisms can be composed of a variety of materials. Various forms of glass, lead crystal, and quartz (natural and artificial) are used in the visible region. Well-cut diamonds sparkle in the light because of a prism effect. Inorganic salts, like sodium chloride, can be used to make prisms for the infrared region of the spectrum.

Reflecting Prisms

According to Snell's law, when light is trying to pass from a medium of higher refractive index to a medium of lower refractive index, at some critical angle, θ_c the angle of refraction becomes 90° with respect to the line perpendicular to the surface. For any two materials, the critical angle is given by the expression

θ_c =arcsin n₂/n₁ , n₂<n₁

If the incoming light approaches the interface at any angle greater than θ_c, it does not pass through the interface; rather, it is reflected. This phenomenon is called total internal reflection, and the surface is acting as a mirror essentially.

Some prisms have the primary job of reflecting light using total internal reflection. In many — but not all — cases, the light is also dispersed. For example, in the Abbe prism (Figure 3), a 30-60-90 right triangle takes advantage of total internal reflection. The Abbe prism has the characteristic that a certain wavelength of light comes out 60° relative to the incoming light beam, and by rotating the prism, the wavelength that comes out at 60° can be changed without changing the spatial position of the selected wavelength. As such, the Abbe prism is referred to as a constant deviation prism. A similar prism is the Perrin–Broca prism, a four-sided prism having angles of 90°, 75°, 135°, and 60° that directs a certain wavelength of light 90° from the incoming path, again rotating the prism to select the particular wavelength but not moving the position of the outgoing beam. The Perrin–Broca prism also relies on both dispersion and total internal reflection.

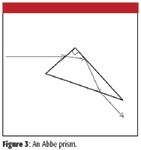
Figure 3

A Dove prism (Figure 4) is a piece of a 45-45-90 right triangle prism with the right angle cut off parallel to the hypotenuse. Because the two angled faces are at opposite angles, any refraction introduced at the first interface will be canceled by refraction at the second interface, so when used properly, a Dove prism does not introduce any net refraction, nor any net beam deflection (that is, the direction of the outgoing light is the same as that of the incoming light). There is one total internal reflection, however. Also, the image is inverted: it flips top to bottom but not left and right, as shown in Figure 4. An interesting feature of the Dove prism is that as it is rotated along its long axis, the outgoing image rotates at twice the angular rate as the prism. This makes a Dove prism very useful in, among other applications, altering the direction of polarization of linearly polarized light.

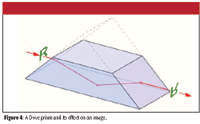
Figure 4

A Porro prism takes advantage of two internal reflections to reverse the direction of incoming light (Figure 5). The image inverts top and bottom but does not exchange left and right.

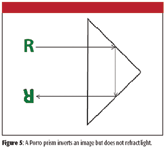
Figure 5

A rhomboid prism (Figure 6) changes the position of the incoming light but not the direction or orientation of the image. It, too, depends upon internal reflection.

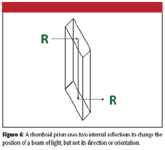
Figure 6

Some prisms change the direction of light, but do so because they have silvered faces and not because of internal reflection. A rather common example of this is the pentaprism, shown in Figure 7, along with a similar prism called a roof pentaprism. The pentaprism simply changes the direction of the incoming light by 90°, but in doing so, it also reverses the image laterally (that is, left to right). The roof pentaprism has two sloped sides on one of the reflecting surfaces, which serve to reverse left and right again so that the image comes out in the same orientation as it went in. Roof pentaprisms are common in single-lens reflex cameras as part of the camera viewfinder; because of the prism, the image you see in the eyepiece of the camera is the same as what you see when you look at the scene without the camera.

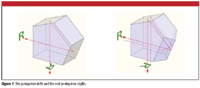
Figure 7

All of the prism examples so far have been single pieces, although some prisms like the Abbe prism can be constructed by cementing multiple triangular prisms together. Some prisms are always constructed out of multiple pieces, glued together with a substance like Canada balsam, a turpentine-like substance that has a refractive index close to that of glass. Although it can be made out of a single piece, the Porro–Abbe prism (Figure 8) usually is made by cementing four right-angle prisms or two double right-angle prisms together. The Abbe–Koenig prism (Figure 9) also is made by cementing two smaller prisms together. Its overall function is similar to the Porro–Abbe prism, but the final light path is the same as the incoming light path, whereas the Porro–Abbe prism moves the path of the light.

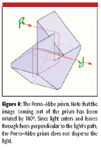
Figure 8

Polarization Effects

Some solid, transparent crystals are birefringent — they have two different indices of refraction depending on the crystal axis that the light is passing through. One common form of birefringent material is calcite, a crystalline form of calcium carbonate. For visible light, the two indices of refraction of calcite are about 1.4 and 1.6. If light were to pass through a sample of calcite, it would be split into two separate beams. One of the beams of light would look as if the light passed straight through the crystal. This beam is composed of what are called the ordinary rays, or o-rays, of light. The other, displaced beam of light is composed of the extraordinary rays, or e-rays, of light. Each of these rays is now composed of linearly polarized light, with the two polarizations perpendicular to each other.

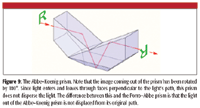
Figure 9

The Nicol prism (Figure 10) takes advantage of this phenomenon. Formed by two calcite prisms having a 68° angle, as the o-rays and e-rays diverge, the o-rays internally reflect at the inner boundary and are directed away. The e-rays emerge, linearly polarized and slightly shifted in path. A Wollaston prism (Figure 11) is similar in effect but does not involve internal reflection of the o-rays.

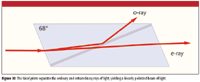
Figure 10

A Glan–Taylor prism (Figure 12) is a common polarizing prism that separates the e-ray from the incoming beam. It is composed of two right triangles of calcite or other birefringent material. The optical axis of the birefringent material is collinear with the polarization of the e-ray, and the two prisms are separated by a small space. In this prism, only the extraordinary ray is transmitted, while all of the ordinary ray and only some of the extraordinary ray is internally reflected. A Glan–Foucault prism is similar, except that in this case, the optical axis of the calcite is perpendicular to that of the Glan–Taylor prism. The extraordinary ray coming out of this prism has a polarization 90° from that of the Glan–Taylor prism. Finally, the Glan–Thomson prism is the same as a Glan–Foucault prism, except that the two right-angle prisms are glued together. This prism works the same way as the Glan–Foucault prism, but it has a lower limit of light input due to the potential for damage of the glue by the incoming light.

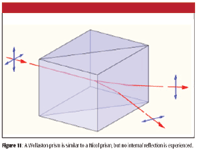
Figure 11

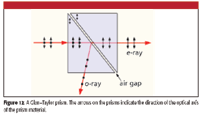
Figure 12

David W. Ball is a professor of chemistry at Cleveland State University in Ohio. With the appearance of this column, he is also a published poet. Many of his "Baseline" columns have been reprinted in book form by SPIE Press as The Basics of Spectroscopy, available through the SPIE Web Bookstore at www.spie.org. His most recent book, Field Guide to Spectroscopy (published in May 2006), is available from SPIE Press. He can be reached at d.ball@csuohio.edu; his website is academic.csuohio.edu/ball.

References

(1) E. Hecht, Optics, 4th ed. (Addison-Wesley, New York, 2002).

(2) J. Gage, Color and Meaning: Art, Science, and Symbolism (University of California Press, Berkeley, California, 1999).

(3) D.W. Ball, Spectroscopy 13(2), 18–19 (1998).

Fan Mail

Paul R. Loconto, PhD, a laboratory scientist specialist with the Michigan Department of Community Health (Lansing, Michigan), sent the following email:

Dr. Ball,

Thanks for your four-part series on introductory quantum mechanics. I even reviewed matrix algebra to verify that the multiplication of the 2 x 2 matrices as you showed is not commutative.

Paul