Article
Special Issues
Spectroscopy Supplements
The Application of Computational Chemistry to Problems in Mass Spectrometry
Author(s):
Quantum chemistry is capable of calculating a wide range of electronic and thermodynamic properties of interest to a chemist or physicist. Calculations can be used both to predict the results of future experiments and to aid in the interpretation of existing results. This paper will demonstrate some examples where quantum chemistry can aid in the development of mass spectrometric methods. Gas-phase electron affinities (EAs) have been difficult to determine experimentally, so the literature values are often not reliable. Computational methods using quantum chemistry have allowed the compilation of a self-consistent database for the EAs of polynuclear aromatic compounds. Likewise, proton affinities (PAs) and ionization potentials (IPs) have been calculated and compared favorably with experimental results for these molecules.
Quantum chemistry is capable of calculating a wide range of electronic and thermodynamic properties of interest to a chemist or physicist. Calculations can be used both to predict the results of future experiments and to aid in the interpretation of existing results. This article demonstrates some examples where quantum chemistry can aid in the development of mass spectrometric methods. Gas-phase electron affinities have been difficult to determine experimentally, so the literature values are often not reliable. Computational methods using quantum chemistry have allowed the compilation of a self-consistent database for the electron affinities of polycyclic aromatic compounds. Likewise, proton affinities and ionization potentials have been calculated and compared favorably with experimental results for these molecules.
The Schrödinger equation (equation 1) provides a complete description of almost any problem in chemistry.

This eigenvector problem (with its Hamiltonian, H, operating on a wave function description of the molecule, Ψ) can generate the spatial distribution and energy levels, E, of a molecule’s electrons from which chemical properties arise. This equation arose from the formulation of quantum mechanics in the 1920s. Thereafter, much of the real power of this new field of theoretical chemistry lay dormant for nearly 40 years. The problem with its implementation was in the extreme complexity in solving the Schrödinger equation for an accurate solution. The simplest mathematical expression of the equation requires one function for each electron; however, the behavior of each electron and atomic nucleus affects all others. In all, the calculations for a simple molecule could involve more than half a million terms, each containing a six-dimensional integral. These can be solved only through an iterative process involving hundreds or thousands of mathematical cycles. Theorists working on these problems made assumptions or simplifications that were so extreme that the computed results often did not agree with the experimental results. Experimentalists, therefore, grew to have little faith in these methods for a while.
With the advent of new methods and supercomputers, the situation has improved dramatically. For example, one of the most successful applications of molecular quantum mechanics has been the reproduction and prediction of molecular conformation. Even with the use of low levels of theory (that is, using many approximations) for moderately large molecules, bond lengths have been computed to within better than ±0.01 Å accuracy and bond angles to ±3°. Using larger basis sets (that is, the internally stored standard set of coefficients and exponents that define the orbitals), even the accuracy of crystallography can be challenged. Computational chemistry has finally escaped the boundaries of purely theoretical chemistry. Chemists can now study chemical phenomena by performing computationally intense calculations on computers rather than by examining reactions and compounds experimentally. This is especially attractive when the actual laboratory experiments are time-consuming, costly, dangerous, or difficult. Computational chemistry can now provide chemists with ready access to modern theoretical tools capable of determining molecular structures, molecular spectra, and energetics, and of elucidating reaction pathways and chemical reaction products.
The state of the art in computational chemistry has advanced sufficiently that the calculations derived from the electronic configurations of the molecules of interest can approach the accuracy of data produced by experimental methods (1,2). The calculations are usually performed on supercomputers with software programs such as Gaussian (3). For certain problems, therefore, it may be advantageous to perform quantum calculations instead of the actual laboratory experiments. Thus, computational chemistry can serve as a vital adjunct to experimental chemistry.
This approach has been effective in several fields (for example, physical chemistry, organic chemistry, and pharmacology) but has not always been used extensively in the field of mass spectrometry (MS). We present here some examples where computational chemistry provides added insight into applications of ion chemistry monitored by MS.
Various MS techniques (1,4,5) have been developed for the measurement of gas-phase basicities of hydrocarbons. These gas-phase data permit the determination of the stabilities of a large number of fundamentally interesting carbocations and a detailed analysis of the factors affecting their stabilities (1,4,5). As both gas-phase experimental techniques and theoretical methods have been developed to permit accurate determination of the energetics of gas-phase ionic processes, careful comparisons of experimental and theoretical results provide important insights into the current state of theoretical methodology and into the interpretation of experimental data. Using methodology found to be accurate and efficient for smaller hydrocarbon species, we calculated gas-phase basicities, proton affinities, and heats of formation of a series of small and moderately large polycyclic aromatic hydrocarbons (PAHs) and also larger hydrocarbons and ions of interest. The approximate ±1.5 kcal/mol accuracy now possible in such calculated energies provides the exciting prospect for using calculations to fill in data inaccessible experimentally or clarifying cases where the experimental results are in question.
The prediction of electron affinities of PAHs was undertaken to test methodology for computationally challenging cases of open-shell negative ions and to compare with experimental electron affinities. The calculation of both proton affinities and electron affinities could lead to information that help define the inherent positive- and negative-ion sensitivities in such molecules. On the basis of some preliminary experimental work in this area, it appeared there was some correlation between positive- and negative-ion sensitivities and proton affinities and electron affinities, respectively (6). By developing a theoretical basis for the prediction of proton affinities and electron affinities in cases where experimental data are available, one could lay a foundation that would permit calculations with environmentally important molecules that have not been studied experimentally. This approach will permit a more intelligent interpretation of analytical data obtained by current methodology and the design of new analytical procedures that could improve the quality of the data gathered in the future.
Technical Approach
Chemical ionization (CI)-MS (7) has been used extensively as a supplementary method to electron ionization (EI)-MS. Using a protonating agent (for example, CH5+ from methane) at relatively high pressure in an ion source promotes ion-molecule reactions that can result in the formation of abundant protonated molecules, MH+, of the target analyte. The following reaction (equation 2) defines the gas-phase basicity and the proton affinity:
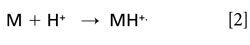
The gas-phase basicity is the negative of the free energy change (ΔG°) of this reaction, while the proton affinity is the negative of the enthalpy change (ΔH°) of this reaction. In “normal” positive-ion MS, the ionization potential is relevant in the formation of radical cations in reaction (equation 3):
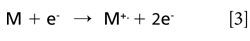
In addition, radical anions can be formed in the ion source by different mechanisms. The mechanism of interest for the proposed study is resonant electron capture (8), which relies on a reagent gas (for example, methane) to provide a buffer to thermalize the electrons in the ion source. The main ion produced under these conditions is the M-. ion. The electron affinity is defined by reaction (equation 4):
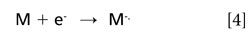
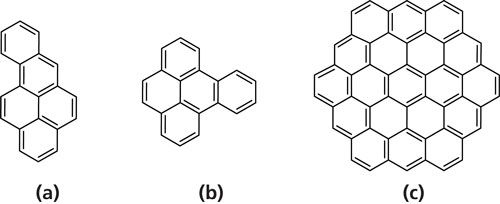
The electron affinity is the negative of the energy change for this reaction. In the course of applying CI-MS to environmental and hazardous waste analysis (6), extreme differences have been observed in the sensitivities of the same compound under the positive-ion mode and the negative-ion mode, and between selected compounds of the PAH class under the negative-ion mode. Since the PAHs are a large class of pollutants, we were interested in using electron affinities as a guide for CI-MS experiments. Further work in other laboratories confirmed these findings (9–12). For example, benzo[a]pyrene (Figure 1a) is 400 times more sensitive in the negative-ion mode than under positive-ion CI. Furthermore, benzo[e]pyrene (Figure 1b) an isomer of benzo[a]pyrene, is only half as sensitive in the negative-ion mode as in positive-ion mode (9). These sensitivity results can be related to the magnitudes of the electron affinities. In fact, Buchanan and Olerich predicted some electron affinities using molecular orbital calculations and correlated with negative-ion sensitivities of PAHs (12).
Figure 1: Structures of the isomers, (a) benzo[a]pyrene and (b) benzo[e]pyrene, and of (c) circumcoronene (C54H18).
Theoretical calculations were performed on the molecules of interest with the Gaussian suite of programs (3). We have carried out these calculations starting out at the semi-empirical level using the Austin Model 1 (AM1), then going to the Hartree-Fock (HF) levels, density functional theory (DFT) with the B3LYP hybrid functional, Møller-Plesset perturbation theory (MP), and coupled-cluster theory. The plan is generally to start with the lowest levels of theory to generate approximate structures in the least amount of time. The lower levels of theory do not have any electron correlation, which is necessary for reliable energies. Frequency calculations at the DFT level are used to give infrared (IR) frequencies of the molecule that indicate whether the structure is a minimum structure or a saddle point and are also used to determine zero-point and thermal energy terms and entropies. Higher levels of theory are applied to achieve the most accurate energies. The various theoretical methods are paired with basis set selections, which approximate the true wave function for the molecule shown in equation 1. In the case of proton affinity calculations, proton affinities are derived from the energy difference between the neutral molecule and the protonated molecule, and between the neutral molecule and the radical ions for ionization potentials and electron affinities. These “electronic” energies are corrected with thermodynamic terms from scaled frequencies (13). These calculated energies are then used to correlate with known experimental values for proton affinities and electron affinities and with analytical sensitivities in negative- and positive-ion mode CI-MS detection of these hydrocarbons in environmental samples. Such studies may lead to improved analytical methodology for specific detection of certain PAHs and for the prediction of analytical sensitivities for unknown new molecules.
Proton affinities calculated from the energies of the neutral PAHs and the closed-shell carbocations formed by protonation present no serious computational problems, but are complicated by the fact that one must ensure that the site of protonation chosen is the most favorable one thermodynamically. Therefore, calculations were required for carbocations at all the different sites of protonation to be sure that the lowest energy isomer was chosen. Calculating electron affinities has other problems as well. Calculating the energy of the odd-electron open-shell radical anion species is complicated by problems with spin contamination from quartet and higher spin states (14). The use of spin annihilation by Schlegel’s projection method (15), as implemented with PMP2 calculations in the Gaussian program, can lead to some improvement in spin contamination in the calculation of electron affinities. We found that the use of the unrestricted UB3LYP method gave the least spin contamination, and is the method we have chosen.
Results and Discussion
Gas-phase basicities and proton affinities were calculated for 45 PAHs. All of these 45 PAHs had experimental gas-phase basicities and proton affinities associated with them. An additional 12 PAHs without experimental gas-phase basicities and proton affinities were calculated to predict their gas-phase basicities and proton affinities (13). One of the purposes of this study was to see how well the calculated gas-phase basicities correlate with good experimental values. Table I shows statistical results for calculated gas-phase basicities of 40 PAHs correlated with known experimental gas-phase basicities. Table I presents the statistics from three levels of theory. The absolute differences between theory and experiment were sometimes large, 11–16 kcal/mol for the HF level of theory and 4–8 kcal/mol for the B3LYP level. The MP2 level, on the other hand, gave absolute gas-phase basicity values closer to experimental (an average of 194.1 kcal/mol for the MP2 theory compared to 193.9 kcal/mol for the experimental gas-phase basicities) (13). The best least-squares fit to experimental gas-phase basicities, however, was found with the B3LYP/6-311G(d,p) level of theory.
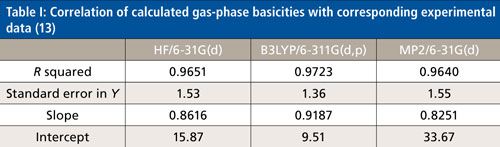
The theory has proved sufficiently successful in correlating with the experimental gas-phase basicities that the computed gas-phase basicities have errors of about the same magnitude as anticipated errors in the experimental values. This suggests gas-phase basicities of PAHs that were outliers in this statistical analysis might need to be reevaluated experimentally.
Calculations determining electron affinities were performed on 45 PAHs, of which 38 had experimental electron affinities available. There have been various ways to determine electron affinities experimentally (16–23). Not all of these methods are equally reliable, so that various experimentally determined electron affinities often differ from one another, in some cases by large amounts, and from computed values. We have also used solution-phase reduction potentials together with estimated solvation energies to independently estimate electron affinity values to serve as a double check on the reliability of experimental electron affinity values. The electron affinities can be related to the reduction potentials in solution by including solvation energies of the neutral PAHs and their radical anions in a thermodynamic cycle (14). The solvation energies of the radical anions can be approximated by the generalized Born electrostatic solvation model (14,24). For the theoretical results, it was determined that the B3LYP/6-31+G(d,p) and the B3LYP/6-311G(d,p) levels of theory showed the smallest standard errors from linear regressions between the theoretical and experimental free energies (14). The standard errors were 0.07 eV (1.6 kcal/mol), with similar errors in the electron affinities for electron affinities from the analysis of reduction potential data. These two approaches have been very effective in compiling a set of electron affinities that we regard as accurate for many PAHs and singling out other electron affinity values as suspect. In fact, we find that nearly half of the electron affinity values in the literature for PAHs are considered unreliable to varying degrees from this analysis.
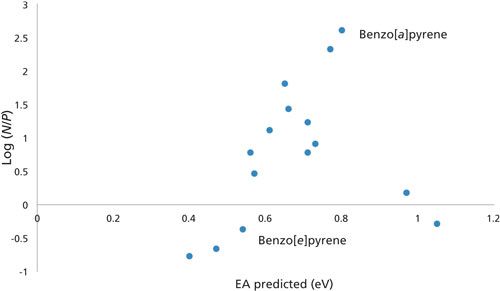
With a reliable set of electron affinities for the PAHs, a good database for comparing sensitivities under negative-ion chemical ionization was presented. In Figure 2 the log of the ratio of the negative-ion to positive-ion sensitivities (N/P) for a series of PAHs (6,9,25) versus electron affinity gives a reasonable correlation. A rough trend is seen with increasing negative-ion sensitivity with increasing electron affinity. Figure 2 shows the data points for benzo[a]pyrene and benzo[e]pyrene isomers. These two PAH isomers differ drastically in negative-ion sensitivity in a way that is consistent with their electron affinities. The N/P for benzo[a]pyrene is 400, while for benzo[e]pyrene it is 0.5. There appears to be a threshold for enhanced sensitivity in the negative-ion mode at an electron affinity of approximately 0.5–0.6 eV. Naphthacene and perylene are outliers to this trend because they both have high electron affinities, but show no enhancement for the negative ion mode.
Figure 2: Semilog plot of the negative to positive ion sensitivity versus predicted electron affinity (EA) for a series of PAHs.
Calculations at levels that are reliably accurate become more challenging with very large molecular structures. We have recently tested these limits for larger PAHs (with the number of carbons from 24 to 54) at the same theoretical levels used for the smaller PAHs (with the number of carbons below 24) discussed so far. These calculations are accessible with the use of high-speed supercomputers and continue to give reliable proton affinity and electron affinity values. In addition, we calculated ionization potentials at these levels of theory which compared favorably with experimental ionization potentials from photoelectron spectroscopy (26). Circumcoronene, shown in Figure 1, is an example of one of these large PAHs. While its high symmetry (D6h) speeds up calculations, Jahn-Teller distortions make the radical cation and radical anion structures more challenging because of their reduced symmetry and open-shell electronic structure, but not impossibly difficult. Looking at comparisons with experimental ionization potentials provides a useful test on the reliability of the calculated results for open-shell systems that became a large issue for electron affinity calculations discussed above. The results for ionization potentials are particularly good test cases because, unlike experimental electron affinities, they are not subject to very significant problems with experimental inaccuracies. Interpretation of photoelectron spectroscopy results are relatively straightforward. What we observe is a level of agreement (circa 1.5 kcal/mol) between experiment and theory for ionization potentials comparable to what we observe for those experimental values we regarded as reliable for proton affinities and electron affinities.
Conclusions
Computational chemistry has been shown to prove helpful in understanding experimental results associated with MS techniques and can be used to determine quantities not available experimentally. The usefulness of computational chemistry has expanded substantially in recent decades with the advent of new quantum mechanical methods and the widespread use of supercomputers. Today, even desktop computers are able to generate useful data using some of the computational chemistry programs that are available.
The application of computational chemistry to the evaluation of experimental determinations of proton affinities and electron affinities has helped to assess the reliability of experimental results. This, in turn, aids the setup of chemical ionization experiments in both the positive-ion and negative-ion modes and helps to explain why some compounds show great enhancement in the negative ion mode and some compounds do not (for example, the benzopyrenes).
In addition to the PAH results discussed above, there are other applications of theory to MS that we have investigated. For example, the unusual ion C10H2+ from the ion-trap mass spectrum of pyrene was subjected to theoretical calculations that showed that the preferred structure was linear and not some ring structure (27). This was in agreement with the experimental results (28). Also, the proton affinity of diborane, B2H6, which is difficult to determine experimentally, was determined by high-level calculations (2).
Acknowledgments
We are pleased to acknowledge support from the Center for Scientific Computing at the CNSI and MRL: NSF MRSEC (DMR-1121053 and NSF CNS-0960316) and the High Performance Computing center of the United States Environmental Protection Agency.
Disclaimer
The views expressed in this article are those of the authors and do not necessarily represent the views or policies of the United States Environmental Protection Agency (US EPA).
References
- D.H. Aue, in Encyclopedia of Computational Chemistry, P.V.R. Schleyer, N.L. Allinger, T. Clark, J. Gasteiger, P.A. Kollman, H.F. Schaefer III, and P.R. Schreiner, Eds. (John Wiley & Sons, Chichester, UK, 1998), pp. 210–220.
- L.D. Betowski and M. Enlow, J. Molec. Struct. (Theochem) 638, 189–195 (2003).
- M.J. Frisch et al., Gaussian 09 (Gaussian, Inc., Wallingford, Connecticut, 2009).
- D.H. Aue and M.T. Bowers, in Gas-Phase Ion Chemistry, volume 2, M.T. Bowers, Ed. (Academic Press, New York, 1979).
- D.H. Aue, in Interdisciplinary Reviews: Computational Molecular Science (Wiley, New York, 2011), pp. 487–508.
- L.D. Betowski, H.M. Webb, and A.D. Sauter, Biomed. Mass Spectrosc. 10, 369–376 (1982).
- F. Hatch and B. Munson, Anal. Chem. 49, 731–733 (1977).
- J.G. Dillard, Chem. Rev.73, 589–643 (1973).
- S. Daishima, Y. Iida, A. Shibata, and F. Kanda, Org. Mass Spectrom. 27, 571–577 (1992).
- G.K-C. Low, G.E. Batley, R.O. Lidgard, and A.M. Duffield, Biomed. Environ. Mass Spectrom. 13, 95–104 (1986).
- L.R. Hilpert, G.D. Byrd, and C.R. Vogt, Anal. Chem. 56(11), 1842–1846 (1984).
- M.V. Buchanan and G. Olerich, Org. Mass Spectrom. 19, 486–489 (1984).
- D.H. Aue, M. Guidoni, and L.D. Betowski, Int. J. Mass Spectrom. 201, 283–295 (2000).
- L.D. Betowski, M. Enlow, L. Riddick, and D.H. Aue, J. Phys. Chem. A110, 12927–12946 (2006).
- H.B. Schlegel, J. Phys. Chem. A92, 3075–3078 (1988).
- National Institute of Standards and Technology (NIST), Standard Reference Database Number 69, March 1998 Release, “Negative Ion Energetics,” data compiled by J.E. Bartmess. (http://webbook.nist.gov/chemistry/).
- R.S. Ruoff, K.M. Kadish, P. Boulas, and E.C.M. Chen, J. Phys. Chem. 99, 8843–8850 (1995).
- E.L. Chaney, L.G. Christophorou, P.M. Collins, and J.C. Carter, J. Chem. Phys. 52, 4413–4417 (1970).
- E.P. Grimsrud, S. Chowdhury, and P. Kebarle, J. Chem. Phys.83, 3983–3989 (1985).
- K.M. Broadus and S.R. Kass, J. Am. Chem. Soc.122, 10697–10703 (2000).
- J.W. Deault, G. Chen, and R.G. Cooks, J. Am.Soc. Mass Spectrom. 9, 1141–1145 (1998).
- S.W. Staley and J.T. Strnad, J. Phys. Chem.98, 116–121 (1994).
- R. Gygax, H.L. McPeters, and J.I. Brauman, J. Am. Chem. Soc.101, 2567–2570 (1979).
- C.J. Cramer and D. Truhlar, Chem. Rev. 99, 2161–2200 (1999).
- M. Oehme, Anal. Chem. 55, 2290–2295 (1983).
- D.H. Aue and L.D. Betowski, unpublished work.
- L.D. Betowski, W. Winnik, A.B. Marcus, and S.M. Pyle, Int. J. Mass Spectrom. and Ion Proc.173, 27–39 (1998).
- S. Lee, N. Gotts, G. Von Helden, and M.T. Bowers, J. Phys. Chem. A.101, 2096–2102 (1997).
Don Betowski is retired from the United States Environmental Protection Agency (US EPA) in Las Vegas, Nevada. Donald H. Aue is with the Department of Chemistry and Biochemistry at the University of California, Santa Barbara. Direct correspondence to: betowski_don@hotmail.com

Newsletter
Get essential updates on the latest spectroscopy technologies, regulatory standards, and best practices—subscribe today to Spectroscopy.



