Article
Spectroscopy
Spectroscopy
The Contribution of Raman Microscopy to the Characterization of Nanomaterials
Raman has a unique capability to characterize nanoscale materials that are between crystalline and amorphous.

Raman is usually thought of as a tool for studying molecular and crystalline structure; in this context it also can differentiate the amorphous phase from any number of crystalline phases. However, it is also known that Raman spectra of a crystalline phase can be acquired under conditions where X-ray crystallography indicates that the sample is amorphous. With the development of nanophase materials, Raman is exhibiting a unique capability for characterizing materials that are between crystalline and amorphous.
I heard recently that the concept of nanoscience lies with Richard Feynman, one of the boy geniuses of the Manhattan Project. If you do not know who he was, you can do a Google search on Feynman's name that will bring you to a web site (feynmanonline.com) with this interesting summary of who he was: "This web site is dedicated to Richard P. Feynman (1918–1988), scientist, teacher, raconteur, and musician. He assisted in the development of the atomic bomb, expanded the understanding of quantum electrodynamics, translated Mayan hieroglyphics, and cut to the heart of the Challenger disaster. But beyond all of that, Richard Feynman was a unique and multifaceted individual." To get to the point, Feynman delivered a lecture on December 29, 1959, entitled "There's Plenty of Room at the Bottom" (1), which is cited as the origin of nanoscience. Several points are of interest. First, the transcript of the lecture has no term with "nano" in front of it, even though he describes things on the nanoscale level. Second, he describes many technologies that were far from development in 1959, but have since appeared. For example, he described nanolithography for storing all the information accumulated by man in something small enough to carry around, and miniaturizing computers, using germanium as the active substrate! But for us, what may be most interesting is a comment that he makes in the section entitled "Better Electron Microscopes"; he talks about using a better electron microscope that would enable one to visualize where the atoms are for chemical analysis. Maybe such a microscope has come close to realization, but we also should note that spectroscopy has been developed for indirect chemical analysis and is being done during reactions, which would be difficult to do in an electron microscope.
The goal of this column is to discuss the use of spectroscopy in studying nanomaterials, in particular, how Raman spectroscopy is being used to study nanomaterials. However, we are not going to include a discussion of microcrystalline and disordered carbon, carbon nanotubes, or graphene because we have dealt with them in earlier columns, and they actually are special cases.
Phonon Confinement
The essential point to understanding Raman spectra of solid-phase nanomaterials is to recognize the effect of the crystallite size on the optical phonons that we detect. That means reviewing a bit of solid state physics. The reader is directed to the August 2007 issue of the Journal of Raman Spectroscopy, which was devoted to review papers on nanomaterials. In particular, the article by Arora and colleagues (2) discusses the role of phonon confinement in solids. Because this concept is key to understanding how Raman can contribute to the analysis of nanomaterials, we will attempt to summarize the theory here.
In a crystal of essentially infinite dimensions, there is translational symmetry that is reflected in the conservation of momentum selection rule. The phonons in such crystals are described by both their energy (ω) and wavevector (q). The wavevector describes how the phonon propagates in the crystal and is consequently a vector quantity — it has direction and magnitude. The wavevector can vary between 0 and π/2ai, where ai is the lattice constant along the ith axis. In a Raman scattering event the laser light enters a crystal along an axis, for instance the Z direction (for example, a (001) face) and the scattered light can be collected in the back-scattering geometry (-Z); to conserve momentum, for this geometry the scattering wavevector is kl - ks = kl - (-kl) = 2kl = qph where kl is the wavevector of the laser photon in the crystal, ks is the wavevector of the scattered light in the crystal, and qph describes the phonon scattering wavevector. The wavevectors in crystals of all visible photons are so close to zero in the reciprocal lattice that it is said that first order Raman scattering always occurs at the "Brillouin zone center" which means q = 0. It is this approximation that breaks down in nanocrystals.
All states of a crystal (electronic and vibrational or phonons) are described in terms of energy and momentum. Electronic and phonon states cannot assume arbitrary energies and momenta in crystals. These states have to fit the properties of the crystal. The relationship between energy and momentum, called dispersion, is described in Figure 1, which shows the dispersion curves for the acoustic and optical phonon modes in a crystal with two atoms in the unit cell such as silicon, germanium, or diamond. If the crystal is small, the phonons are not free to drift over large distances. There will be scattering at the crystal boundaries which will change the phonon wavevector, effectively introducing uncertainty to its value.
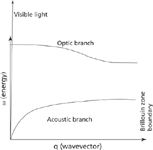
Figure 1
The uncertainty in the phonon momentum means that scattering occurs not only at Δk = q = 0, but for some values of q greater than 0; the uncertainty in q is estimated to be equal to π/d, where d is the size of the grain. Clearly the smaller the value of d, the more the contributions from farther along the dispersion curve. In an early publication dealing with Raman scattering from such small crystals (3), a Gaussian confinement model was developed.
For isolated nanocrystals, the phonon cannot extend beyond the crystal boundary so its wavefunction is to be multiplied by a Gaussian confinement function, W(r), which is sometimes expressed as
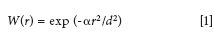
where d is the dimension of a "spherical crystal" and α describes how rapidly the phonon amplitude decays close to the surface. The scattering intensity is predicted to follow
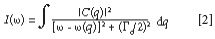
where C(q) is a q-dependent weighting function that depends on the confinement model and Γo is the natural width of the zone-center optical phonon. This equation for I(ω) is compared to the measured spectrum to account for the shift and asymmetric broadening of the peaks. Usually, the dispersion of the optical phonon slopes downward around the center of the Brillouin zone (Figure 1), so the observed Raman signal becomes asymmetric with a tail on the low energy side. In cases where the phonon dispersion curve goes up before it goes down, the asymmetric tail will appear on the high frequency side; this has been observed in TiO2 (4).
It is sometimes the case that more broadening and asymmetry are recorded than can be accounted for by the model. In this case, it is possible to invoke defect-induced scattering that shorten the lifetime and add to the broadening (Lorentzian function). In summary, the asymmetry can be attributed to a number of factors such as particle size distribution and irregularity in the particle shape (Gaussian function), as well as confinement. Arora and colleagues (2) surveyed the Raman scattering in many materials. One of the more interesting examples is porous silicon, which is of technological interest because of its efficient photo- and electroluminescence, despite the fact that the parent silicon is an indirect gap material (which means that it will only luminesce weakly as a phonon is created or absorbed during the electronic transition to take up the difference in wavevector of the electron in the conduction band and the hole in the valence band). In fact, it has been shown that the strong photoluminescence caused by a radiative recombination of carriers across the indirect gap in porous silicon is mediated by the confined phonons (5).
Applications
Since the development of chemical vapor deposition (CVD) methods to synthesize diamond films in the 1980s, Raman spectroscopy has been seen as a valuable tool for characterizing these materials. The size of the diamond crystals, the presence of twinning, and the amount and character of the nondiamond component has been correlated with conditions of deposition. While it was presumed that there could be nanocrystalline diamond present, its Raman spectrum was only identified in the year 2000 with the use of 244-nm excitation (6); the requirement for UV excitation is based on the need to reduce the relative contributions from the nondiamond species, and to detect the Raman signal in the absence of luminescence (which would occur at longer wavelengths). (In fact, the 244-nm-excited spectrum of CVD diamond was recorded in 1995, and the dependence of the peak position and width as a function of deposition conditions was noted, but not identified as evidence for nanodiamond [7].) If the Raman spectra of CVD diamond films are recorded with visible excitation wavelengths, one finds that a broad band at 1150 cm-1 often occurs, and it has often been attributed to nanodiamond. However, the band always occurs with a second band at 1450 cm-1, and the excitation-wavelength dependence of the vibrational energies indicated that it could be more accurately assigned to resonance-enhanced transpolyacetylene segments at grain boundaries (8).
Raman scattering in nanoporous semiconductors also has been reviewed in the 2007 issue of the Journal of Raman Spectroscopy (9). In addition to a discussion of porous silicon and germanium, this review article considers scattering by polar semiconductors in which surface phonons with energy between that of the TO and LO phonons of the bulk material appear. Because the surface phonons couple to the free-carrier plasmons, carrier depletion can be studied.
In their review article, Gouadec and Colomban (10) consider the role of interactions between nanophases in crystal growth and in composite structure and properties. In composites, one needs to be concerned with what happens at grain boundaries as well as in the grains themselves. When the composite is made of nanomaterials, the concentration of the boundaries relative to the bulk is quite high. Reactions at the grain boundaries include lattice reconstruction, passivation or corrosion, and contamination. In addition, large thermal-chemical gradients during processing result in further changes including the production of new phases. An interesting observation that the authors cite is the conversion of gas phase–deposited TiO2 particles from rutile to the anatase structure at 5 nm diameter because of differences in surface energy (11).
Gouadec and Colomban consider the morphology (following crystallization and amorphization processes) of covalently bonded materials, especially inorganic and organic polymers. They point out that the long range order is directly reflected in the lattice and librational modes that occur in the low frequency range of the spectrum (<100 cm-1) and Raman bands of molecular motions broaden in the amorphous phase in an analogous fashion to what is observed in X-ray diffraction (XRD). Thus the vibrations of the molecular motion are sensitive to short range order and the librational and lattice modes are sensitive to long range order required for crystallinity. They point out that it is possible to estimate the relative amounts of crystalline vs. amorphous phase by band-fitting the low frequency part of the spectrum to a Rayleigh background, an amorphous mode, and a crystalline lattice mode. As an example they reproduce the polarized spectra of a polyamide 6.6 fiber. The polarization behavior is observed for both the amorphous and the crystalline components. From these measurements it was possible to understand that the mechanical fatigue of the fiber follows from the transformation of the amorphous phase (12).
At this point, I want to diverge a bit. Since 1990, when Raman instruments were built on a single monochromator platform used in conjunction with some type of Rayleigh filter, the low frequency cutoff has been about 100 cm-1. Until recently, if one wanted to measure spectral features much lower than 100 cm-1, the only recourse was to use a triple spectrograph, which is a more complicated, more expensive instrument with less throughput. Within the past year or two Bragg filters have become available that allow measurement of Raman bands down to 10 cm-1 on specially designed single grating spectrographs (13).
Gouadec and Colomban also described the vibrational motion in nanoparticles. The elastic sphere model employed molecular dynamics simulations to calculate the vibrational density of states (14). The results of these calculations were the description of spheroidal modes (involving radial displacements) and torsional modes (involving tangential displacements with no volume change). Calculations show that the frequency is proportional to the particle diameter, and this finding has been confirmed in some systems.
Gouadec and Colomban also considered in what size domains the two models (phonon confinement vs. elastic sphere models) were most appropriate. They estimated that bulk properties should be adequate for crystals larger than ~50 nm. Between ~5 and 10 nm the elastic sphere model should be used. The phonon confinement model will be valid for crystals in the ~15–50 nm size range. Between ~10 and 15 nm, the elastic sphere and phonon confinement models overlap. Note that when these particles are embedded in a matrix there are additional possibilities for internal stress and other physical phenomena that can affect the spectra.
Ceramics and glass-ceramics are a class of composite materials whose properties can potentially be followed by analyzing their Raman spectra in the framework of nanomaterials. Transformations between various crystalline and amorphous phases during processing through the mesoporous and gel phases can be followed. Because the spectra of both tetrahedral and octahedral species in amorphous metal oxide structures have been assigned, one can infer details of the connectivity and distortions of these glasses as a function of sample history (that is, processing).
One of the more important types of composite is the reinforcement of ceramics with fibers because the final product is refractory, with low density and high damage tolerance. The goal is to incorporate fibers in the amorphous phase and prevent the onset of crystallization and grain growth. The shear stress between a fiber and its matrix can be studied by following the Raman shift as a function of position of the fiber relative to its exposure at the end of the part. (This is only possible when the matrix is optically transparent).
The behavior of glasses was mentioned in some of the examples above. In fact, the study of glass is a field in its own right, and by its very nature it can be aided by the concepts of nanomaterials. The goal of the analysis of glasses is to determine where in the phase space of composition good glasses with low quench rates will form. Experiments are confirming theoretical predictions that the sweet spots occur when there are "rigid but stress-free networks." According to Boolchand and colleagues (15), Raman scattering has been important in determining the "intermediate phases" in which "local and medium range molecular structures . . . form isostatically rigid networks . . . that do not age."
Summary
In this column installment, I tried to indicate how Raman spectroscopy has been contributing to the characterization of nanomaterials that are of intellectual and technological interest. To understand the concepts used to study these materials, interested readers will have to get a crash course in some areas of solid-state science if they have not been exposed to these concepts. The goal in this installment is to indicate what would be of interest in using Raman spectroscopy to study these materials and to provide an entry guide to the literature.
Next Installment
Before we wrap up, I would like to welcome David Tuschel as my collaborator on this column. The feedback that I have been getting on the column is quite good, and consequently the two of us will be publishing five installments a year, instead of the three that I have been publishing until now. David's first column will appear in the March issue. I want to thank all of you that have shared your comments with me. Keep them coming, and be sure to read David's installment next month.
References
(1) http://www.feynmanonline.com/; published by CalTech's Engineering and Science, February 1960.
(2) A.K. Arora, M. Rajalakshmi, T.R. Ravindran, and V. Sivasubramanian, J. Raman Spectros. 38, 604–607 (2007).
(3) H. Richter, Z.P. Wang, and L. Ley, Solid State Commun. 39, 625 (1981).
(4) A.L. Bassi, D. Cattaneo, V. Russo, C.E. Bottani, E., Barborini, T. Mazza, P. Piseri, P. Milani, F.O. Ernst, K. Wegner, and S.E.J. Pratsinis, Appl. Phys. 98, 074305 (2005).
(5) G.W.T. Hooft, Y.A.R.R. Kessenev, G.L.J.A. Rikkin, and A.H.J. Venhuizen, Appl. Phys. Lett. 61, 2344 (1992).
(6) Z.Sun, J.R. Shi, B.K. Tay, and S.P. Lau, Diamond Relat. Mater. 9, 1979 (2000).
(7) R.W. Bormett, S.A. Asher, R.D. Witowski, W.D. Partlow, R. Lizerski, and F. Pettit, J. Appl. Phys. 77(11), 5916- 5923 (1995).
(8) A.C. Ferrari and J. Robertson, Phys. Rev. B. 63, 121405 (2001).
(9) G. Irmer, J. Raman Spectrosc. B 38, 634–646 (2007).
(10) G. Gouadec and P. Colomban, Progress in Crystal Growth and Char. of Mater. 53, 1–56 (2007).
(11) E. Barborini, I.N. Kholmanov, P. Piseri, C. Ducati, C.E. Bottani, and P. Milani, Appl. Phys. Lett. 81(16), 3052 (2002).
(12) J.M. Herrera-Ramirez, P. Colomban, and A. Bunsell, J. Raman Spectrosc. 35(12), 1063 (2004).
(13) A.Rapaport, B. Roussel, H.J. Reich, F. Adar, A. Glebov, O. Mokhun, V. Smirnov, and L. Glebov, "Very Low Frequency Stokes and Anti-Stokes Raman Spectra Accessible with a Single Multi-Channel Spectrograph and Volume Bragg Grating Optical Filters", presented at the International Conference on Raman Spectroscopy (ICORS), Boston, Massachusetts, 2010.
(14) R. Meyer, L.J. Lewis, S. Prakash, and P. Entel, Phys. Rev. B. 68, 104303 (2003)
(15) P. Boolchand, M. Jin, D.I. Novita, and S. Chakravarty, Raman Spectrosc. 38, 660–672 (2007).

Fran Adar is the Worldwide Raman Applications Manager for Horiba Jobin Yvon (Edison, New Jersey). She can be reached by e-mail at fran.adar@horiba.com.
Newsletter
Get essential updates on the latest spectroscopy technologies, regulatory standards, and best practices—subscribe today to Spectroscopy.






