Article
Spectroscopy
Spectroscopy
Modulators
Author(s):
Some types of spectroscopy work better if the intensity of the light source increases and decreases in a regular pattern. Such a varying signal is called modulated, and here, the author explores the devices that perform this function.

Some types of spectroscopy work better if the intensity of the light source increases and decreases in a regular pattern. Electronic detectors, for example, can home in on these regularly varying signals to separate them from nonsignals or stray light using a lock-in amplifier. Such a varying signal is called modulated. Rather than fluctuating the source, which can be difficult or impossible, the light from the source is interrupted mechanically or optically. A modulator is a device that interrupts the light.
Mechanical Modulators
Mechanical modulators are called choppers (see Figure 1). They physically block the light beam. The frequency of the interruption depends upon the angular velocity of the wheel and the pattern of openings; in fact, many choppers come with interchangeable wheels and/or variable speeds so the frequency of modulation can be varied. The intensity pattern of the light from the source approaches a square wave. Chopping speeds can vary to almost zero to over 50,000 Hz.
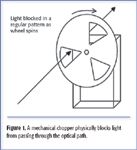
Figure 1. A mechanical chopper physically blocks light from passing through the optical path.
Designs based upon tuning forks also are available. These are called resonant choppers, and consist of a flat paddle attached to a narrow tine. Resonant choppers are designed to have a certain fixed frequency. As the tines on the fork vibrate, the space between the paddles opens and closes with fixed frequency. Because the motion is sinusoidal, the light intensity varies sinusoidally. Chopping rates of over 5 kHz are possible.
Intermittent chopping is performed with a shutter. A shutter can be as simple as a card mounted on a rotating axis, or as complex as an iris used in a camera. Unlike choppers, which are controlled electronically, a shutter can be controlled electronically or manually. Shutters also vary from other modulators in that the frequency of chopping can be deliberately variable — mechanical choppers can be designed to have a variable frequency, but because many of them depend upon a rotating mask, even the variable frequency has a repetitive pattern to it.
Electro-Optical Modulators
An electro-optical modulator is based upon the change in the refractive index of a birefringent material due to the presence of an electric field. The Pockels effect is the change in refractive index of a medium in the presence of an electric field. The change in refractive index is directly proportional to the strength of the field. As polarized light travels through the medium, it changes its polarization. If the medium is sandwiched between crossed polarizers, light will only pass if the change in birefringence is enough to make the resulting polarization the same as the outgoing polarizer; this is a Pockels cell, and is illustrated in Figure 2. By modulating an electric field placed across the medium (up to more than 100 MHz), the Pockels cell becomes a modulator. The electric field is parallel to the propagation vector of the light. Materials used for crystals include potassium dihydrogen phosphate, deuterated potassium dihydrogen phosphate, lithium niobate, and beta-barium borate.
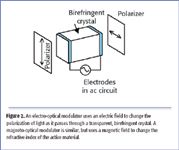
Figure 2. An electro-optical modulator uses an electric field to change the polarization of light as it passes through a transparent, birefringent crystal. A magneto-optical modulator is similar, but uses a magnetic field to change the refractive index of the active material.
A Kerr cell is similar to a Pockels cell except that it depends upon the Kerr effect, a transverse effect on the refractive index that depends upon the square of the electric field. The electrodes are mounted on the sides, not the ends, of the cell. Modulations of over 10 MHz are possible. Nitrobenzene is a common medium.
Magneto-Optical Modulators
A magneto-optical modulator uses the Faraday effect, a change in the refractive index due to the presence of a magnetic field. The magnetic field must be parallel to the propagation of the light. Many materials, including flint glass and water, show a Faraday effect. Certain kinds of garnet show very strong Faraday effects as well.
The magneto-optical modulator differs from the electro-optical modulator in that it can rotate the plane of linear polarized light by any angle, whereas electro-optical modulators typically rotate the plane of polarized light by 90°. The relationship between the rotation angle and the strength of the magnetic field is
θ = B·V· l
where θ is the angle of rotation, B is the strength of the magnetic field in tesla, l is the length of the active medium, and V is Verdet constant, the proportionality constant in the equation that is characteristic of the medium. The Verdet constant is wavelength-dependent and has units of rad/(T·m).
Acousto-Optical Modulator
When passing a soundwave of given wavelength through a medium, the pressure of the sound waves can change the refractive index of the medium. Because these variations occur at regular intervals (equal to the wavelength of the sound wave), incoming light will be diffracted in much the same way as a crystal defracts X-rays (Bragg defraction). This is an acousto-optical modulator (also called a Bragg cell), which is illustrated in Figure 3. Acousto-optical modulators can be constructed of crystalline lithium niobate, lithium tantalate, tellurium dioxide, or titanium dioxide.

Figure 3. An acousto-optical modulator uses standing sound waves to set up refractive index differences in a solid material. The regular refractive index variations can then refract light of certain wavelengths by certain angles, much like a crystal diffracts X-rays via Bragg diffraction.
David Ball is a professor of chemistry at Cleveland State University in Ohio. Many of his "Baseline" columns have been reprinted in book form by SPIE Press as The Basics of Spectroscopy, available through the SPIE Web Bookstore at www.spie.org. His new textbook, Physical Chemistry, was published in August 2002. He can be reached at the Department of Chemistry, Cleveland State University, Cleveland, OH 44115, by e-mail at d.ball@csuohio.edu, or by phone at (216) 687-2456. His personal website is academic.csuohio.edu/ball

Newsletter
Get essential updates on the latest spectroscopy technologies, regulatory standards, and best practices—subscribe today to Spectroscopy.



