Raman Applications That Are Driving a Rapidly Expanding Market

Because of the weakness of the Raman signal and the relative high intensity of the laser line, traditional Raman instruments (before 1990) were made with large double monochromators (with a single-channel detector) or large triple monochromators (with a multichannel detector). Many technological innovations starting in about 1990 made it easier to build instruments with higher sensitivity and lower utility requirements (1). Because of this, the percentage of Raman users who do analysis rather than study the light-scattering phenomenon itself has grown, as has the number of publications illustrating solutions to real-world problems. Raman is now known to be a beneficial, easy-to-use technique. While hard numbers are not readily available on the market size (especially taking into account that there were always people who "built" their own systems with monochromators not sold as Raman instruments), we can assume that 20 years ago, before the rapid evolution started, there were probably not more than 150–200 instruments sold per year. On the other hand, according to the 2006 SDI report (2), no less than 860 instruments were shipped in 2005. Their assessment is that the market is far from mature. In this article we will try to indicate why people are using Raman spectroscopy.

Fran Adar

Raman Activity

Raman spectroscopy is a light-scattering phenomenon in which a laser photon of well-defined energy (<0.5 cm^–1 in width) is scattered by a molecule or crystal. Usually this scattering is mediated by vibrational transitions. To have something vibrate, there must be at least two atoms to beat against each other. The more atoms in a molecule or crystal, the more "degrees of freedom," and therefore the greater the number of bands that will appear in the spectrum. So the first thing that we learn is that we are detecting bonded species — single atoms or elemental ions will not have a vibrational spectrum (not rocket science).

Organic Molecules

The next question is how to derive analytical information from the spectra. This is where things become interesting. As the number of atoms that vibrate gets bigger, the interpretation gets more challenging. One would like to associate a given band with a particular vibration. Those of you who use IR spectroscopy know that there are bands that are easy to identify (CH, SH, >C=C<, >C=O functional groups), but the spectrum below 1500 cm^–1 becomes quite complicated. This is because the vibrations of the various atoms are coupled together strongly. Who remembers the coupled pendulum experiment in elementary physics? Two balls of similar mass are hanging from a support. The balls are weakly coupled together with a weak spring. When you start one ball in motion, you will find that the remaining ball will slowly start to move. One mass cannot move independently of anything to which it is coupled. The same thing happens in molecules or crystals. Everything is coupled together, so if you try to imagine isolating the motion of one atom, it will start to pull on atoms of similar masses that have similar bonds (that is, force constants) that are close to it. The result is that if you imagine everything wiggling together, the wiggling will relax into "normal modes," which is a name for the natural vibrations of the system. Each vibration, which defines a natural state, is independent of other vibrations, and each one will have its own characteristics that include frequency, intensity, and polarization properties. If you change the molecule slightly, such as by adding a methyl side group to a linear hydrocarbon, the spectrum will probably change dramatically. The reason that some functional groups do have bands that can be recognized and can be easily identified is that the criteria for similar mass or force constant are not met. So, for CH and SH modes, the proton, which has a very small mass, beats against the C or S. If you calculate the reduced mass for the two groups, you will see that they are quite different, so the frequencies are quite different (2800–3100 cm^–1 for CH versus ~2500 cm^–1 for SH). The reason the CH band can have such a range is that there are often multiple protons on each carbon that couple together, and as a second order effect, the hydrogen motion is affected by what is attached to the carbon. As a rule of thumb, aliphatic CH groups appear below 3000 cm^–1, ethylenic CH groups appear near 3015 cm^–1, and aromatic CH groups appear near 3060 cm^–1. You might wonder why I do not list OH and NH groups. The answer is that when they are isolated from interactions, they have sharp bands at 3334 cm^–1 (NH₃ gas phase) and 3652 cm^–1 (H₂O, gas phase), but H-bonding has profound effects on the spectra (3). Because the H-bonding in hydroxyl is much stronger than in NH groups, these effects are potentially stronger. The stronger the H-bonding, the more the bands will be downshifted. And when the environment of the NH or OH group is not homogeneous, there can be very large effects on the widths. In liquid H₂O, the band is several hundred cm^–1 wide. But in some aluminum-containing minerals, the OH band splits and is quite sharp. Because of these effects, it often is not easy to spectrally differentiate OH from NH.

For anyone interested in using Raman spectroscopy for interpretation of spectra of organic materials, in conjunction with or without IR spectroscopy, it is strongly recommended that a few good references be acquired (4,5).

Inorganic Materials

The situation for inorganic materials is in some ways more complicated, and in some ways less complicated. For those materials that have identifiable molecular groups (carbonates, nitrates, phosphates, sulfates, silicates, and others in a form of MO_n), the number of atoms in the molecules is much more limited than that of organic materials. In these cases the frequency of the symmetric stretch of each of these groups tends to be fairly robust; it can shift somewhat with anion. In the solid form, however, there can be more than one crystal phase, which can cause shifts, crystal field splittings, and correlation field splittings when there is more than one molecular unit in the unit cell. Unfortunately, there is no textbook (of which we are aware) that covers these materials adequately, but we can point you to some literature articles that can get you started (6–8). In addition, you will need to be prepared to learn the geological nomenclature; for instance, the two forms of CaCO₃ are called calcite and aragonite. Geologists can tell you the differences in their structures.

The situation becomes even murkier when you look at inorganic materials in which there really is no molecular functional group in the unit cell. In this case, when there is more than one crystallographic phase, the spectra do not even indicate that the chemistry is identical. The most prominent example is that of carbon. There is graphite, other black carbons in various states of disorder, fullerenes (buckyballs and nanotubes, single-walled and multiwalled), and diamond. All of these structures are pure carbon, but they all have different spectra. Another class of materials with this behavior is some metal oxides. TiO₂ and ZrO₂ both occur in three crystal phases, whose spectra appear totally different. Even SiO₂ occurs in many phases, due in part to the ability of silicon to be coordinated tetrahedrally and octahedrally.

An area of particular importance is that of metal oxides. The Raman spectrum does not directly tell you the oxidation state nor the stoichiometry, but it does provide a "fingerprint" that gives unequivocal identification as a secondary standard. Consider the oxides involved in corrosion of common metals. Fe⁺³₂O₃, also known as hematite, is orange, and it has a distinctly different spectrum from Fe₃O₄, (Fe⁺³₂ Fe⁺²O₄), known as magnetite (the mineral that is magnetic and is sometimes used in a compass). Then there are two forms of iron oxy hydroxide, FeOOH; goethite and lepodocrocite have different spectra. Metallic iron is rarely used in its unalloyed form. The oxides of alloys often are composed of more than one metallic element, and the composition is also reflected in the spectra. The best source of information for these systems is the scientific literature, but some information is available in a text by Ross (9).

The Raman spectra of semiconductors also should be mentioned here. The most common ones are either formed from one element (Si, Ge, diamond) or two elements (III-V and II-VI materials). Si, Ge, and diamond have two atoms in the unit cell, which is why they are Raman active (refer to the discussion at the beginning). The lattices are cubic, so the vibrations of the group IV semiconductors associated with each of the directions in space are degenerate (that means equivalent), so one line appears in the spectrum. The III-V and II-VI semiconductors occur either in the cubic form (analogous to silicon) or the wurtzite form. Remember my comment about geological names? Wurtzite is the geologists' name for ZnS. It is a hexagonal structure closely related to the diamond–silicon structure by rotating every other set of planes in the (111) direction. If one of these semiconductors occurs in the cubic form, there are two possible bands observed in the Raman spectrum. This is a result of the fact that the two atomic types allow for polarity. When the phonon (vibration) is composed of atomic motion parallel to the phonon wavevector (scattering direction), the energy of the field separation adds to the vibrational energy, producing a longitudinal phonon with higher frequency than the transverse phonon. In case you are not confused enough, if there are free carriers, they will shield this effect and the longitudinal optical (LO) phonon (which can be identified by its polarization properties) will appear at the frequency of the transverse optical (TO) phonon. And there is more. There can be electric field effects at the surface due to something called "surface states." And by using different excitation wavelengths, you can control the depth of penetration of the light to separate these various effects. In the wurtzite lattice, there are two functional groups in the unit cell, which is hexagonal. So there are more phonons possible, some of which will also exhibit field effects.

Then there is bandgap engineering, in which different semiconductors are layered, sometimes with near atomic layer thicknesses. Sometimes layers are repeated to make synthetic crystals called superlattices. The spectra will show effects of boundaries, superlattices, fields, and confinement. Fortunately, there are some textbooks available to help with all this (10,11).

Before we go on, let me just add that the Raman effect can be mediated by transitions other than vibrations (10). These include electronic transitions (for instance, rare earth ions in crystals or "free electrons" in semiconductors, or plasmons) and magnetic transitions. But usually these are phenomena that are studied by physicists, and not of interest to most analysts.

Applications in Materials Development, Characterization, and Analysis

So now that we know what we can measure, let's see where it can be useful.

Polymers

The polymer molecule can be identified, and its "morphology" characterized. The morphology refers to the physical state of the polymer. In processing, has it been oriented or crystallized? These parameters will affect the performance of the polymer and are engineered into manufactured parts. For example, polyethylene terephthalate (PET) fibers used in tire cords must be strong (high orientation, maybe not so crystalline), and PET films used for drug delivery must be amorphous so that there is a means for the active pharmaceutical ingredient (API) to diffuse. Raman spectra enable differentiation between the amorphous and crystalline phases, and determination of the degree of orientation. Very often, metal oxides such as TiO₂ are added to control the optical properties, and these are easily detected and identified.

Pharmaceutical Tablets

Virtually all of the components of the tablets are detectable by Raman spectroscopy. Manufacturers need to know if their final products are consistent with their formulations. How well have the components been mixed? Has the active pharmaceutical ingredient (API) changed polymorph or pseudomorph (acquired or lost water) during the tablet production or during its shelf life? Even though Raman spatial resolution has been considered overkill for this application (laser spot size about 1 μm vs. particle size 20 μm or larger), new techniques are showing how high-sensitivity Raman maps can be acquired over the entire range of the tablets.

Bioclinical Research

There is a great deal of excitement in this area among researchers who understand the potential that Raman can have in characterizing biological tissue. Not only can lipids, proteins, nucleic acids, and carbohydrates be differentiated, but members of each class have different spectra. This area, however, is far from mature, offering fantastic opportunity for anyone looking to explore new ways to characterize disease processes.

Metal Oxides

The ability to record Raman spectra and maps of metal oxides covers the broadest range of scientific disciplines — corrosion, art conservation, paint pigments, and catalysis, to name a few. The end products of corrosion enable one to model the corrosion mechanism, and consequently to figure out how to prevent it. The identification of metal oxides in art pieces enables the conservator to improve their condition, preserving cultural heritage. Identification of oxides enables analysis and quality control of manufactured paints. The systematic analysis of the catalytic surface, under well-defined chemical–physical conditions, provides a detailed understanding of the catalytic mechanism for a given system; subsequent engineering of the catalytic substrate can provide billions of dollars in fuel and product savings.

Figure 1 shows a Raman image of an iron alloy that had been corroded. The sample had been embedded in epoxy and cross-sectioned. Video images showed areas of varying shades of white and grey. The white areas tended to be nonoxidized metal, whereas the grey regions reflected spectra of a mixture of hematite (Fe₂O₃) and magnetite (Fe₃O₄). The Raman image was reconstructed from the hyperspectral cube produced by mapping the sample. Pure spectra were extracted from the cube and used to model the map.

Figure 1: Micrograph of a corroded metal surface. The piece had been embedded in epoxy, cross-sectioned, and polished.

Nanomaterials

Raman spectroscopy has been found to be the method of choice for characterizing carbon nanotubes, and because of the intense enhancement, there is sensitivity to single tubes.

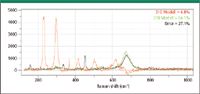
Figure 2: Model spectra used to fit the hyperspectral cube. The red spectrum represents hematite, and the green spectrum represents magnetite.

Integrated Circuits

The current state of the art in the engineering of electronic circuits on semiconductors makes use of the fact that engineering strain into the substrate can improve the electronic mobility, and therefore the overall performance. Because of this, strain is being engineered into silicon by several methods, and the strain is being measured with 0.2-μm spatial resolution by the Raman microscope. In addition, Raman has been used to identify contaminating defects on semiconductors as well as many other commercial products.

Figure 3: (a) Raman images of hematite and magnetite; (b) same as (a) but with two images overlaid.

In considering any of these applications, keep in mind that Raman spectroscopy might not be a primary measurement. That means that if, for example, you want to determine the crystalline phase of ZrO₂, the spectrum doesn't tell you the phase directly (as X-ray diffraction [XRD] would). But once someone has measured the XRD and Raman spectrum of the different phases, you know which spectrum corresponds to which phase. So you can use the Raman spectra to identify the phase under conditions where XRD would not work; that is, at higher spatial resolution.

Figure 4: Raman image and video overlaid.

Summary

Analysis of engineered and manufactured materials can now benefit from Raman spectroscopy. This follows from the instrumental evolution of the last 20 years. This note surveys what kind of information is available from Raman spectroscopy and what applications can benefit from their measurement.

Fran Adar is the Worldwide Raman Applications manager for Horiba Jobin Yvon (Edison, NJ). She can be reached by email at: fran.adar@jobinyvon.com

References

(1) F. Adar, M. Delhaye, and E. DaSilva, J. Chem. Educ. 84, 50 (2007).

(2) Global Assessment Report, 9th Edition, July 2006 (Strategic Directions International, Inc., Los Angeles).

(3) G. Herzberg, Infrared and Raman Spectro of Polyatomic Molecules (D. Van Nostrand, Co., Princeton, New Jersey, 1945).

(4) G. Socrates, Infrared and Raman Characteristic Group Frequencies, Tables and Charts (John Wiley and Sons, Ltd., Chichester, UK, 2001).

(5) D.W. Mayo, A. Miller, and R.W. Hannah, Course Notes on the Interpretation of Infrared and Raman Spectra (Wiley-Interscience, Hoboken, New Jersey, 2004).

(6) W.P. Griffith, J. Chem. Soc. A, 1372, 286–291 (1970).

(7) A. Wang, J. Han, L. Guo, J. Yu, and P. Zeng, Appl. Spectrosc. 48, 959–968 (1994).

(8) R. Frost, T. Klopogge, and J. Schmidt, Non-destructive Identification of Minerals by Raman Microscopy, 2003, www.sci.qut.edu.au/minerals/ijvs%20Raman%20paper/IJVS%20Raman%20paper.htm

(9) S.D. Ross, Inorganic Infrared and Raman Spectra (McGraw-Hill, London, 1972).

(10) R. Hayes and R. Loudon, Scattering of Light by Crystals (Dover Publications, 2004) (a reprint of the 1978 edition published by Wiley).

(11) T. Ruf, Phonon Raman-Scattering in Semiconductors, Quantum Wells and Superlattices: Basic Results and Applications, Series #142 (Springer-Verlag Berlin and Heidelberg GmbH & Co. KG, 1997).