Research on Hardness Detection Method of Polyvinyl Chloride Cable Sheath Material Based on Laser-Induced Breakdown Spectroscopy

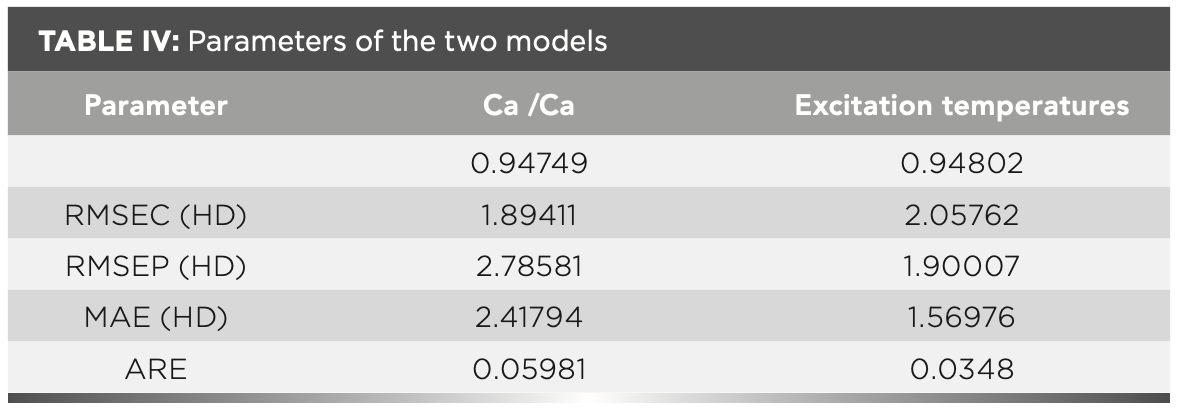
Surface hardness is one of the most important parameters which describes the degree of aging in polyvinyl chloride (PVC) cables. In this work, the hardness of PVC sheathing material was studied using laser-induced breakdown spectroscopy (LIBS). At first, the experimental samples were subjected to different time of aging. Then, the trends of ionic and atomic spectral line intensities of Ca elements in the samples were analyzed with the variation of sample hardness. The relationship between the ratio of intensity in Ca II and Ca I spectral lines and the hardness of samples was analyzed. It was found that there was an obvious linear relationship between the ratio of the intensity at Ca II 422.0071 nm and Ca I 429.8988 nm spectral lines with the hardness of samples. A hardness estimation model was built using the linear relationship between them. The coefficient of determination (R²), root mean square error of calibration (RMSEC), root mean square error of prediction (RMSEP), mean absolute error (MAE), and absolute relative error (ARE) of the model were 0.94749, 1.89411 HD, 2.78581 HD, 2.41794 HD, and 0.05981, respectively. In addition, the relationship between the plasma excitation temperature (T_e) and the hardness of samples was investigated. There was a linear relationship between plasma excitation temperature and sample hardness. And the relationship between the plasma excitation temperature and sample hardness was used to build a shore harness tester (HD) estimation model. The R², RMSEC, RMSEP, MAE and ARE of the model were 0.94802, 2.05762 HD, 1.90007 HD, 1.56976 HD and 0.0348, respectively. By comparing the above two models, it was found that the model for estimating the hardness of the plasma excitation temperature was better performing than the ion-to-atomic line ratio model. It was more suitable for estimating the surface hardness of PVC cables.

Polyvinyl chloride (PVC) polymers are widely used in cable sheathing. However, the cable is used for a long time, and susceptible to environmental factors such as light, high temperature, and humidity. The PVC material has aged, resulting in a decrease in insulation performance. During the aging process, there will be a decrease in the organic content of the surface, an increase in hardness, and a decrease in tensile resistance (1,2). The hardness is an important indicator that measures the degree of aging in PVC sheathing materials. Currently, the hardness of cable sheaths is usually measured using a hardness tester. This inspection method requires close contact with transmission cables, which is dangerous. At the same time, due to space constraints in some cable-laying areas, it is not convenient for maintenance personnel to conduct direct testing. In addition, in-depth testing of the degree for cable surface aging requires thermogravimetric analysis (TGA) and pyrolysis experiments in the laboratory (3). There is some degree of limitations to these cable aging detection methods. Therefore, it is necessary to seek a new detection method of cable aging.

Laser-induced breakdown spectroscopy (LIBS) is a non-contact method of elemental detection. A plasma is generated by focusing a pulsed laser on the sample’s surface for ablation through a lens. The spectral information from the plasma is collected by the lens set. Then, it is analyzed by spectral analysis software and algorithms. LIBS has many distinct advantages, including non-destructive, fast, and in-situ online. Little sample pretreatment is required, and there is minimal damage to the object being tested (4,5). These features of the LIBS are just enough to compensate for the problems that exist in traditional cable hardness testing methods. By measuring the surface hardness of the cable through the LIBS, combined with indicators of the degree of aging such as residual hardness and ratio of residual hardness (RHH) to 100 (6,7), it will potentially bring great convenience to the aging inspection of cables. Currently, the LIBS research in hardness has focused on the following areas. Abdel-Salam and co-authors (8) investigated the hardness of different calcified tissues. They found that the hardness of calcified materials is proportional to the shock wave velocity and ion-to-atom line intensity ratio. Cowpe and associates (9) studied the relationship between sample hardness and plasma properties of bioceramics using LIBS. A linear relationship was found between sample surface hardness and plasma temperature. The hardness method based on plasma excitation temperature measurements was more reproducible than Vickers hardness measurements. Kasem and associates (10) investigated the spectral intensity ratio of calcium ions to atoms in the LIBS spectra. The surface hardness of the excavated bones was estimated to distinguish the different periods. Panya and associates (11) used LIBS to study rock samples of different hardnesses. A linear relationship was found between rock sample hardness and plasma excitation temperature. At present, most scholars are focusing their research on inorganic materials, but there is little research on the surface hardness of organic polymer materials such as PVC.

In this study, the spectra of elemental Ca in PVC cable sheathing samples with different aging times were selected for analysis. At first, the trend of ionic spectral line and atomic spectral line intensity with sample hardness was analyzed. The relationship that exists between the ratio of ionic and atomic spectral line intensities and the hardness of the sample was investigated. The hardness estimation model was established by the ratio of ionic and atomic lines. The relationship between the plasma excitation temperature and the hardness of the sample was then investigated. The hardness estimation model was established using the relationship between plasma excitation temperature and hardness. Finally, the performance of the two hardness prediction models was analyzed in comparison.

Materials and Methods

Samples

The flame-retardant PVC-sheathed flexible cable (ZR-RVV Shanghai Shenghua Cable) used in the experiment is shown in Figure 1. The composition of the PVC cable sheath samples is shown in Table I. First, the cable samples were aged. In the aging process, the plasticizer of samples was evaporated with the increase in temperature (12). This leads to an increase in the surface hardness of the PVC cable sheath. According to the studies of Zuzana and associates, a significant increase in the reaction rate can be achieved by increasing the temperature (activation energy) in the appropriate range. For most samples, the temperature increase of 10 °C can increase the reaction rate by a factor of one to four (13). The maximum operating temperature of the PVC cable samples in the experiment was 70 °C. Rapid thermal aging was simulated using the 105 °C constant temperature heating method. The 15 samples of the same length were intercepted from the same PVC cable. All samples were placed in the constant temperature oven at the same time, and one sample was removed at the same time interval. Samples of rapid thermal aging were placed on a sample table to ventilate and cool down, and hardness was measured after 24 h. Sample hardness was measured by a shore hardness tester (HD). For each sample, the hardness was measured at five different locations. The average of the five measurements was then calculated. Some samples showed large deformations during the heating process, which affected the accuracy of the sample hardness measurement. Therefore, only 10 samples with small errors in hardness measurement were selected. Their spectral information was collected for analysis. During the processing of the data, the average value was calculated for the ten sets of spectral data collected for each sample. Figure 2 shows the relationship between heating time and the hardness of the sample. The hardness of the samples gradually increased with the increase of heating time.

FIGURE 1: ZR-RVV fire retardant cable.

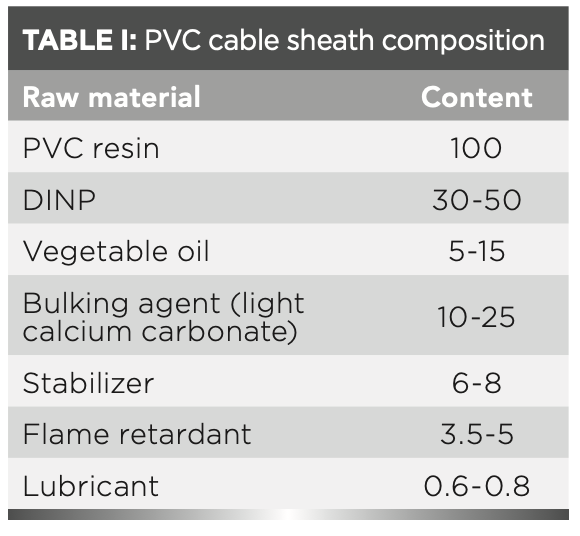
FIGURE 2: Relationship between hardness and heating time of PVC sheath material.

A shore hardness tester was used to measure hardness in the experiment. The principle is to calculate the hardness value based on the depth of the probe inserted into a cable. The depth of probe entry into the cable represents the amount of cable hardness during the measurement. A greater depth indicates a less hard sample. Conversely, it means more hardness. The residual hardness retention rate RRH (equation 1) and residual hardness H’ (equation 2) (6,7) are often used to estimate the degree of aging in cable aging testing. The residual hardness is the difference between 100 and the hardness value of the test sample. The following equation:

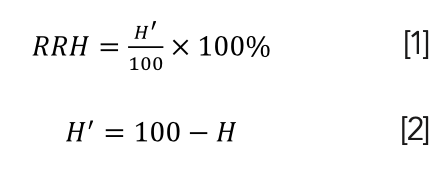
where H’ represents the residual hardness, and H represents the surface hardness of the sample. In the cable aging test, 10%–30% RRH is selected as the cable life failure standard based on the different environments, insulation safety level, and other factors. When the degree of cable aging reaches this standard, it should be promptly stopped and overhauled or replaced.

Experimental Installation

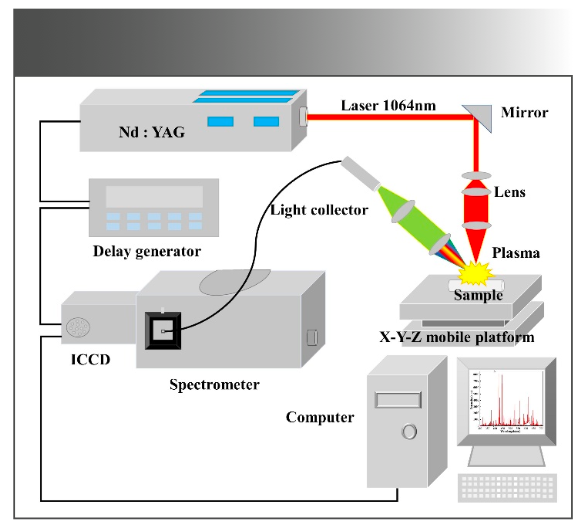
A schematic diagram of the experimental system used in this work is shown in Figure 3. The light source is an Nd: YAG laser (Nimma-400) with a wavelength of 1064 nm, laser energy of 60 mJ, a frequency of 1 Hz, and a beam pattern of TEM₀₀. In an ultra-clean laboratory environment, the pulsed laser beam was focused on the sample surface by a lens placed vertically with a focal length of 150 mm. The laser bombarded the sample to generate plasma. The light emitted from the plasma was focused into an optical fiber by a horizontally placed lens, which was fed into the spectrometer (Andor SR-500i). The light emitted from the plasma passes through the internal light path of the spectrometer, and it shines on the light-sensitive module of the ICCD camera. The spectral information was input to a computer by the ICCD camera. The received data was converted into processable data by the analysis software. A digital time-delay trigger synchronization controlled the timing between the laser and the spectrometer.

FIGURE 3: Experimental Installation.

Analysis Method

The relationship between the plasma excitation temperature and the hardness of the samples was analyzed for different hardness samples (14). The plasma excitation temperature was calculated using the Boltzmann plane method. The plasma in the excited state obeys the Boltzmann distribution.

Laser-induced breakdown of the plasma is a transient process. The plasma produced in the laboratory is non-equilibrium over a large range (for example, non-uniform temperature distribution, etc.), but divided into a sufficiently small range to be considered homogeneous. The plasma is considered to be in local thermodynamic equilibrium (LTE) when it contains a sufficient number of particles within this local range to allow statistical averaging. The atomic or ion-bound states satisfy the Boltzmann distribution. The intensity of the emission spectrum for the plasma transition from the E_i energy level to the E_k energy level satisfies equation 3 (15,16):

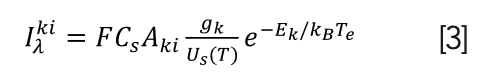
where k and i are the upper and lower energy levels of the spectral line transitions. l^ki is the relative intensity of the measured spectral line. F is the experimental parameter for factors such as optical efficiency and plasma number density of the receiving system. C_s is the concentration of particles transitioned, and A_ki is the transition from the upper energy level to the lower energy level; g_k is the statistical weight, U_s (T) is the partition function, and E_k is the excitation energy of the upper energy level. k_B is the Boltzmann constant (8.617×10^-5 eV/K), and T_e is the plasma excitation temperature.

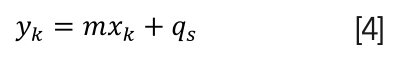
Equation 4 is obtained by taking the logarithm of both sides of equation 3, where y_k=ln(l^ki/_gkA_ki), x_k=E_k, m=-1/k_BT_e, q_s=ln((C_sF)⁄(U_s [T]). The value of x_k on the right side of equation 4 is the horizontal coordinate, and the value of y_k on the left side of the equal sign is the vertical coordinate. The different spectral lines from the same ionization state of the same element were selected for fitting. The plasma excitation temperature was calculated from the slope of the linear fit.

In q_s=ln(C_sF)⁄(U_s[T]), several selected spectral lines are at the same ionization energy level. The lepton particle concentration C_s and the partition function U_s (T) are identical. Thus, q_sis a constant that could be neglected. The plasma excitation temperature can be calculated using equation 4.

The hardness model was established by ion line atomic line ratio and plasma excitation temperature. The performance of the model is usually expressed in terms of parameters such as the coefficient of determination (R²), root mean square error (RMSE), mean absolute error (MAE), and absolute relative error (ARE). Their mathematical expressions are shown in equations 5, 6,7, and 8, respectively.

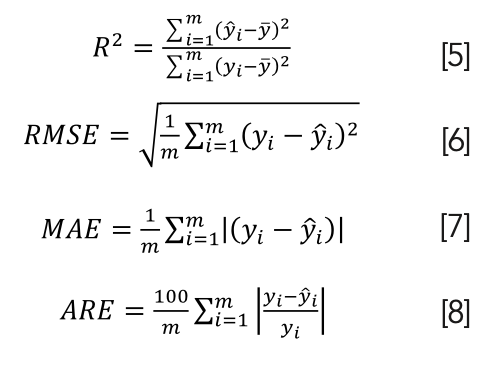
The y_i in the formula represents the true value and the y^_i represents the predicted value, and y^_ represents the average value. The value of R² ranges from [0,1], the closer to 1, the better the regression fit. The higher value of R², the more accurate the model and the more significant the regression effect. The RMSE is used to represent the deviation between the observed and true values. RMSE is in the range of [0, +∞), and the larger the value, the error between the observed value and the true value is larger and the model performance is poorer. MAE reflects the prediction error. It takes values in the range of [0, +∞), and the closer its value is to 0, that indicates the better performance of the model. The value of ARE is in the range [0, +∞). The value of ARE is closer to 0, which means the model performance will be better. On the contrary, the larger the value of ARE indicates the worse the model performance.

Results and Discussion

LIBS Spectral Analysis

The spectrum of PVC cable samples collected after rapid thermal aging treatment was analyzed. The spectra of the samples in the 300–700 nm band after 12 h rapid thermal aging are shown in Figure 4. Based on the National Institute of Standards and Technology and atomic spectral analysis software Spec-Line, it was found that the sample contained mainly Ca, Al, and other metal elements (17). The sample has a large amount of light calcium carbonate filler and the most abundant spectral lines of elemental Ca. Therefore, elemental Ca was chosen as the object of study. The hardness estimation model was established using the relationship between the Ca II to Ca I ratio and the hardness of the sample. Also, the relationship between the plasma excitation temperature of element Ca and the hardness of the sample was studied. The model for hardness estimation was established using the relationship between them.

FIGURE 4: The LIBS spectra of the samples subjected to 12 h rapid thermal aging were collected, and baselines were removed.

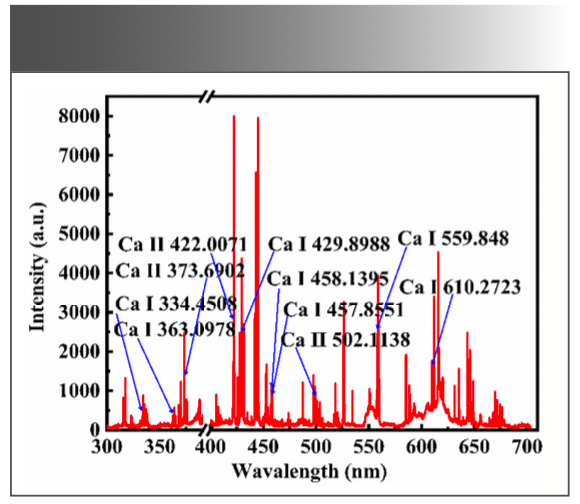
The corresponding spectral information collected was queried through the National Institute of Standards and Technology’s Atomic Spectroscopy Standard Technical Database. The spectral lines of Ca II 373.6902 nm, 422.0071 nm, 502.1138 nm and Ca I 363.0978 nm, 429.8988 nm, 610.2723 nm in the samples were studied. The relationship between the spectral intensity ratios of Ca II and Ca I and the hardness of the samples was analyzed. The relationship between the plasma excitation temperature and the hardness of the samples was investigated by the Ca I 334.4508, 429.8988, 457.8551, 458.1395, 559.848, and 610.2723 nm atomic spectral lines of element Ca. The parameters of Ca elemental spectral lines are shown in Table II.

Hardness Estimation of Ion Spectral Line to Atomic Spectral Line Ratio

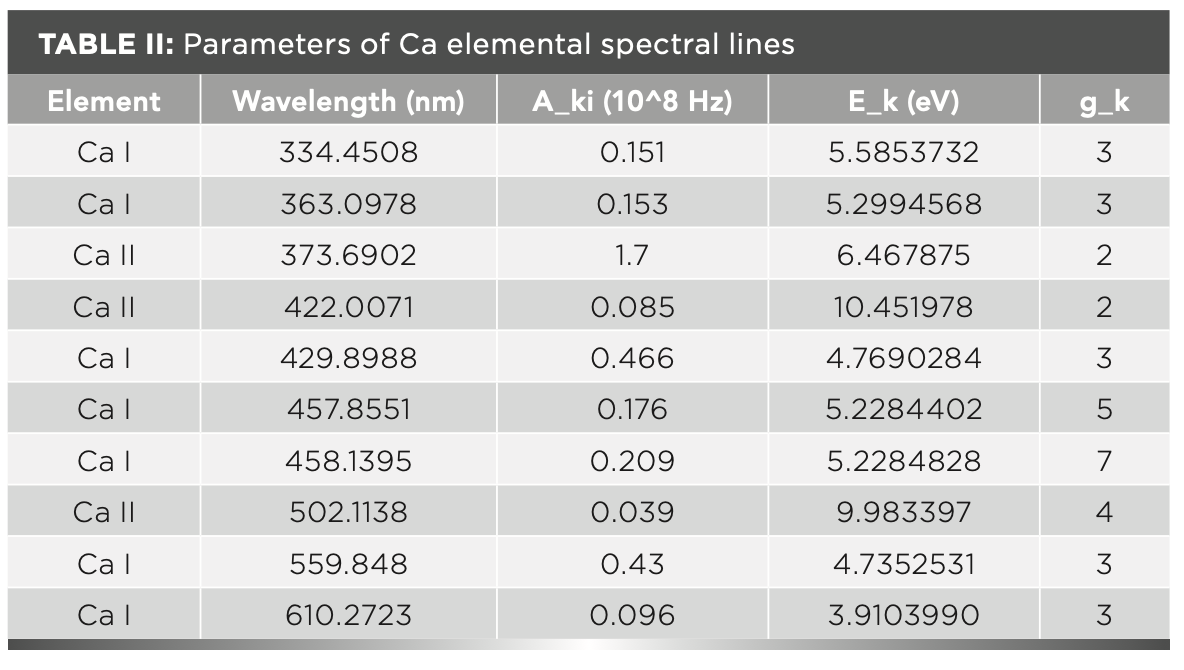
In this study, the relationship between the intensity ratios of Ca II 373.6902 nm, 422.0071 nm, 502.1138 nm and Ca I 363.0978 nm, 429.8988 nm, 610.2723 nm spectral lines of element Ca and the hardness of the samples was investigated. The ion-to-atom spectral line intensity ratio was used to estimate the hardness of the PVC sheath sample. Firstly, the trend of ionic and atomic spectral line intensities for element Ca with increasing sample hardness was investigated. The trend of the ionic and atomic line intensities with increasing sample hardness is shown in Figure 5. It can be seen that the increase in the intensity of the Ca II 422.0071 nm ionic spectral line is the largest in the increasing hardness of the sample. The increase of ionic spectral line intensity at Ca II 373.6902 nm and Ca II 502.1138 nm is greater than the increase of atomic spectral line intensity at Ca I 363.0978 nm. As the hardness of the sample increases, the increase in the intensity of most ionic spectral lines is greater than that of atomic spectral lines. These phenomena may be related to the velocity of the shock wave generated during the plasma excitation. The shock wave velocity becomes larger when the pulsed laser bombards the surface of a hard sample (8,18–20). This leads to faster free electron motion in the plasma. The free electrons moving at high speed collide with other particles, resulting in more collisional ionization. The increase in ionization in the final plasma plume increases the number of ions to a greater extent than the number of atoms. High-speed free electrons collide with other particles, resulting in more collisional ionization. The increase in the degree of ionization of the plasma plume leads to a larger number of ions than atoms. The ion density becomes larger when the laser is bombarded on the surface of a harder sample. This makes the absorption coefficient of laser energy absorbed by the plasma larger. The plasma absorbs more energy in this process and the plasma excitation temperature increases.

FIGURE 5: Variation of ionic and atomic spectral line intensity of element Ca vary with hardness.

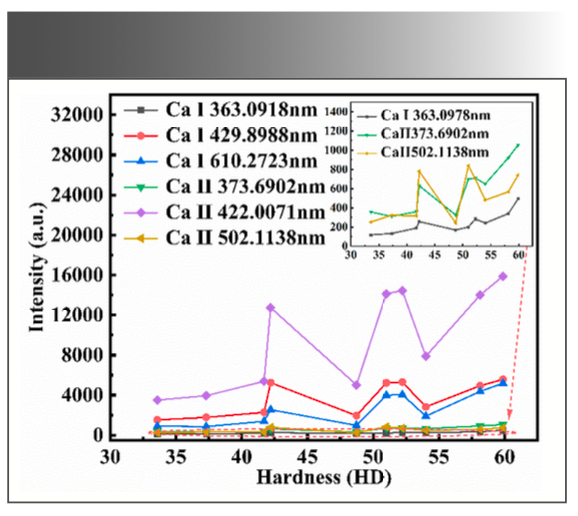
Based on the ratio of ionic spectral lines to atomic spectral line intensities, the relationship between their respective ratios and the hardness of the sample is analyzed. The ratio of the intensity of Ca II 373.6902 nm, 429.8988 nm, 422.0071 nm to Ca I 429.8988 nm, 502.1138 nm, Ca I 429.8988 nm spectral lines in each sample was calculated. By analyzing the relationship between their respective ratios and the sample hardness, a strong linear relationship was found between the spectral intensity ratios of Ca II 422.0071 nm and Ca I 429.8988 nm and the sample hardness. This phenomenon may be due to the fact that as the hardness of the sample increases, the degree of ionization also increases. Finally, the increasing trend of Ca II 422.0071 nm ionic spectral intensity is greater than Ca I 429.8988 nm, and the ratio of their spectral intensity also increases. From these ten samples, seven samples were selected to fit the model and three samples were used for prediction. The criterion for selecting the prediction samples was to remove the first and last samples and randomly select three samples from the middle eight samples as prediction samples. In the seven samples used to fit the model, linear regression analysis was performed using their Ca II 422.0071 nm and Ca I 429.8988 nm spectral line ratios with the sample hardness data. The linear regression model was obtained as y = 39.95464x-55.90103, where y is the sample hardness, and x is the ion-to-atom line ratio. As shown in Figure 6, the predicted hardness and the actual hardness were derived from the linear regression analysis. The R² between the actual and predicted hardness of the model was 0.94749. The sample hardness model’s RMSEC, RMSEP, MAE, and ARE were 1.89411 HD, 2.78581 HD, 2.41794 HD, and 0.05981, respectively. According to the distribution of the training and test data and the parameters of the model. It can be seen that at the early stage of aging, the hardness of the sample changes less, and the predicted hardness of the model has a large deviation from the actual hardness. The deviation of the predicted hardness from the actual hardness becomes smaller as the sample hardness increases. The large fluctuations may be due to the fact that fewer spectral lines were utilized in building the ion line atomic line ratio model, which is susceptible to factors such as self-absorption effects (19,21–23).

FIGURE 6: The relationship between the predicted hardness and the actual hardness of the ion line atomic line ratio model.

Hardness Estimation of Plasma Excitation Temperature

As the sample hardness increased, the laser energy absorbed by the plasma and the plasma excitation temperature simultaneously increased. The relationship between plasma excitation temperature and sample hardness was studied. The plasma excitation temperature was calculated by the Boltzmann plane method. Based on the data in Table II, y_k was calculated from equation 4. A linear fitting was then performed with the values of y_k and x_k to calculate the corresponding slope. The final plasma excitation temperature was calculated from the slope.

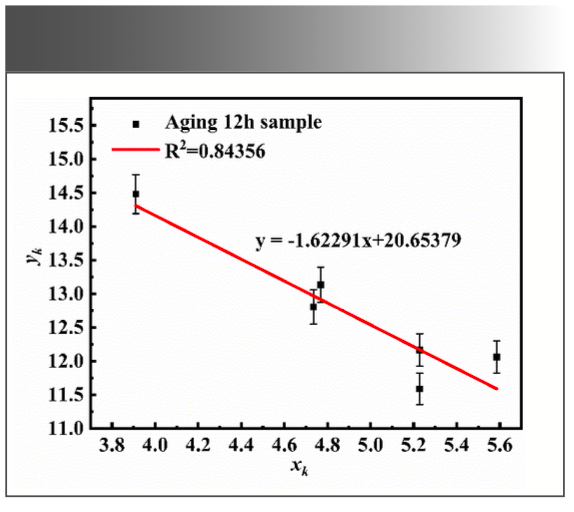
The Boltzmann slope of the spectral data for the aging 12 h sample is shown in Figure 7. The slope diagrams of the Boltzmann plane method were drawn using the Ca I 334.4508 nm, 429.8988 nm, 457.8551 nm, 458.1395 nm, 559.848 nm, and 610.2723 nm atomic spectral lines of element Ca. The plasma excitation temperature was calculated using the slope and equation 4, as shown in Table III.

FIGURE 7: Linear fit of x_k to y_k for Ca.

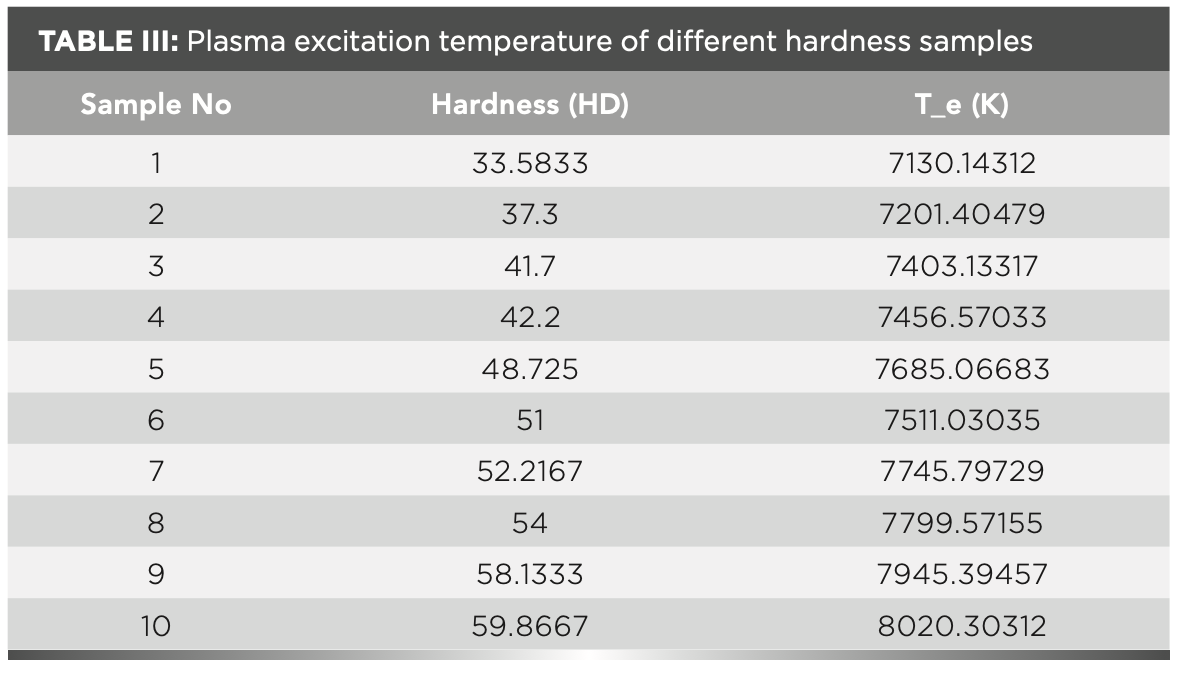
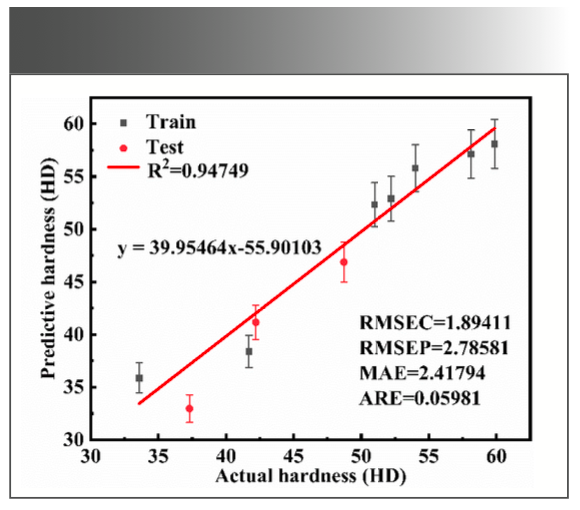
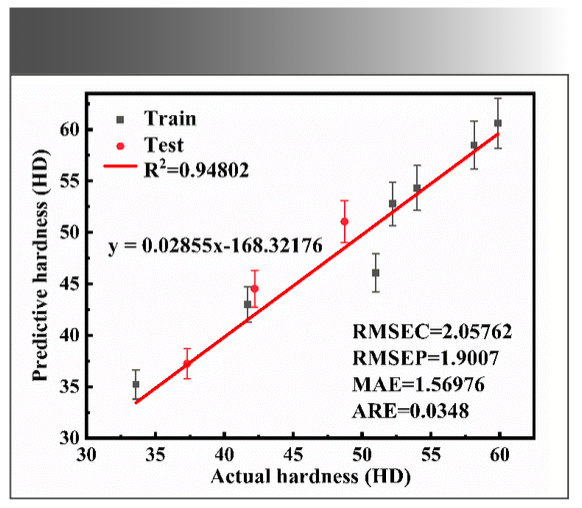
Based on the data in Table III, the relationship between plasma excitation temperature and sample hardness was established. The data from seven samples were used for model training, and data from the remaining three samples were used for prediction. A strong linear relationship was found between the sample hardness and the plasma excitation temperature. The regression analysis resulted in a linear model of y = 0.02855x-168.32176, where y is the sample hardness, and x is the plasma excitation temperature. There was a linear relationship between sample hardness and plasma excitation temperature. This phenomenon may be due to the stronger repulsive force of the shock wave generated by the pulsed laser bombardment on the surface of a hard sample. The chances of collision for various particles in the plasma plume are increased. Under the influence of stronger shock wave repulsion, electron motion is accelerated, and electron collisions with other particles are increased. The plasma absorbs more energy, and the plasma excitation temperature increases (24). The relationship between the predicted hardness and the actual hardness of the samples from the linear regression analysis is shown in Figure 8. The model’s R², RMSEC, RMSEP, MAE, and ARE were 0.94802, 2.05762 HD, 1.90007 HD, 1.56976 HD, and 0.0348, respectively. By analyzing the parameters of the model and the relationship between predicted and actual values, it can be seen that the plasma excitation temperature model as a whole tends to be stable.

FIGURE 8: The relationship between the predicted hardness and the actual hardness of the plasma excitation temperature model.

The performance of the ion-to-atom line ratio model and the plasma excitation temperature model were comprehensively compared. By comparing the parameters of R², RMSEC, RMSEP, MAE, and ARE in Table IV, it can be seen that the performance of the plasma excitation model was better than that of the ion-to-atom line ratio model. The plasma excitation temperature model had test results with less error. The RMSEC values of the plasma excitation temperature model were slightly larger than those of the ion-to-atom line ratio model. It may be due to the effect of the self-absorption effect that occurs during the experiment. Overall, the stability and accuracy of the plasma excitation temperature model were better. During the experiments, it was found that the laser bombardment on the surface of samples with different hardnesses produced different strengths of self-absorption effects.

Conclusions

In this work, the trend of the ionic spectral lines intensity and atomic spectral lines intensity with the hardness of the sample was observed. It was found that as the hardness of the sample increased, the degree of ionization also increased. The relationship between the ratio of Ca II and Ca I spectral line intensities and the hardness of the samples was investigated. It was found that there was a significant linear relationship between the ratio of Ca II and Ca I spectral line intensities and the hardness of the samples. A hardness estimation model was established using the ratio of Ca II and Ca I spectral line intensities. The R², RMSEC, RMSEP, MAE, and ARE of the model were calculated by linear regression analysis as 0.94749, 1.89411 HD, 2.78581 HD, 2.41794 HD, and 0.05981, respectively. The model of ion-to-atom line ratio has a large deviation in the model for samples with small hardness, and the deviation becomes smaller as the hardness increases. The ion-to-atom line ratio model was susceptible to factors such as self-absorption effects due to the small number of spectral lines utilized. The relationship between the plasma excitation temperature and the hardness of the sample was also investigated. It was found that there was a significant linear relationship between plasma excitation temperature and sample hardness. Linear regression analysis was performed on the hardness and plasma excitation temperature of elemental Ca samples. The R², RMSEC, RMSEP, MAE, and ARE of the model were obtained as 0.94802, 2.05762 HD, 1.90007 HD, 1.56976 HD, and 0.0348, respectively. By comparing the performance of the ion-to-atom line ratio model and the plasma excitation temperature model, it was found that the plasma excitation temperature model has a minor error and it is more suitable for predicting the hardness of the sample. In the course of the experiment, different degrees of self-absorption was found, which would influence the experimental results. The future work will focus on studying the effects of self-absorption effects and how to attenuate them. To make the early application of LIBS to the aging detection of PVC cables.

Acknowledgments

The authors are grateful to Yangpeng Xia for his guidance in data analysis, Jiangfei Yang and Xingyu Yue for their help during the experiments.

Declaration of Conflicting Interests

The authors declared no potential conflicts of interest with respect to the research, authorship, and/or publication of this article.

Funding

This paper was supported by National Natural Science Foundation of China (NSFC, No.51374040). Department of Science and Technology of Jilin Province of China (grant No.20200403008SF) and Education Department of Jilin Province of China (grant No. JJKH20210737KJ).

References

1. Wang, Z.; Wei, R. C.; Wang, X. H.; He, J. J.; Wang, J. Pyrolysis and Combustion of Polyvinyl Chloride (PVC) Sheath for New and Aged Cables via Thermogravimetric Analysis-Fourier Transform Infrared (TG-FTIR) and Calorimeter. Materials 2018, 11 (10), 1997. DOI: 10.3390/ma11101997
2. Wang, Q.; Wu, W.; Tang, Y. F.; Bian, J. J.; Zhu, S. W. Thermal Degradation Kinetics of Plasticized Poly (Vinyl Chloride) with Six Different Plasticizers. J. Macromol. Sci. Part B: Phys. 2017, 56 (6), 420–434. DOI: 10.1080/00222348.2017.1316659
3. Mun, S. Y.; Hwang, C. H. Experimental and Numerical Studies on Major Pyrolysis Properties of Flame Retardant PVC Cables Composed of Multiple Materials. Materials 2020, 13 (7), 1712. DOI: 10.3390/ma13071712
4. Klein, S.; Hildenhagen, J.; Dickmann, K.; Stratoudaki, T.; Zafiropulos, V. LIBS-Spectroscopy for Monitoring and Control of the Laser Cleaning Process of Stone and Medieval Glass. J. Cult. Herit. 2000, 1, S287–S292. DOI: 10.1016/S1296-2074(00)00173-4
5. Orcid, J. M.; ElFaham, M. M.; Laserna, J. Dual-Spectroscopy Platform for the Surveillance of Mars Mineralogy Using a Decisions Fusion Architecture on Simultaneous LIBS-Raman Data. Anal. Chem. 2018, 90 (3), 2079–2087. DOI: 10.1021/acs.analchem.7b04124
6. Meng, X.; Wang, Z.; Li, G. Life Assessment of Marine Ethylene Propylene Rubber Power Cables Based on Hardness Retention Rate. Arch. Electr. Eng. 2017, 66 (3). DOI: 10.1515/aee-2017-0035
7. Meng, X. K.; Han, P. J.; Liu, X. Y.; Jin, T. The Aging Degree Analysis of EPR Cable Insulation Based on Hardness Retention Rate Measurement. J. Electr. Electron. Syst. 2018, 7, 2332–0796.1000260. DOI: 10.4172/2332-0796.1000260
8. Abdel-Salam, Z. A.; Galmed, A. H.; Tognoni, E.; Harith, M. A. Estimation of Calcified Tissues Hardness via Calcium and Magnesium Ionic to Atomic Line Intensity Ratio in Laser Induced Breakdown Spectra. Spectroc. Acta Pt. B-Atom. Spectr. 2007, 62 (12), 1343–1347. DOI: 10.1016/j.sab.2007.10.033
9. Cowpe, J. S.; Moorehead, R. D.; Moser, D; et al. Hardness Determination of Bio-Ceramics Using Laser-Induced Breakdown Spectroscopy. Spectroc. Acta Pt. B-Atom. Spectr. 2011, 66 (3–4), 290–294. DOI: 10.1016/j.sab.2011.03.007
10. Kasem, M. A.; Russo, R. E.; Harith, M. A. Influence of Biological Degradation and Environmental Effects on the Interpretation of Archeological Bone Samples with Laser-Induced Breakdown Spectroscopy. J. Anal. At. Spectrom. 2011, 26 (9), 1733-1739. DOI: 10.1039/C1JA10057B
11. Galmed, A. H.; Steenkamp, C.; Ahmed, I.; et al. Using Laser-Induced Breakdown Spectroscopy to Monitor the Surface Hardness of Titanium Samples Bombarded by Carbon Ions. Appl. Phys. B-Lasers Opt. 2018, 124, 1–7. DOI: 10.1007/s00340-018-7093-8
12. Csányi, G. M.; Bal, S.; Tamus, Z. Á. Dielectric Measurement Based Deducted Quantities to Track Repetitive, Short-Term Thermal Aging of Polyvinyl Chloride (PVC) Cable Insulation. Polymers 2020, 12 (12), 2809. DOI: 10.3390/polym12122809
13. Šaršounová, Z. The Inconveniences Related to Accelerated Thermal Ageing of Cables. Transp. Res. Procedia. 2019, 40, 90–95. DOI: 10.1016/j.trpro.2019.07.015
14. Panya panya, S. N.; Galmed, A. H.; Maaza, M.; Mothudi, B. M.; Harit, M. A. Laser-Induced Breakdown Spectroscopy (LIBS) on Geological Materials: Relative Hardness Estimation. Mater. Today Proc. 2021, 36, 600–603. DOI: 10.1016/j.matpr.2020.05.766
15. Wang, X. L.; Hong, X.; Wang, H.; et al. Analysis of the Silicone Polymer Surface Aging Profile with Laser-Induced Breakdown Spectroscopy. J. Phys. D-Appl. Phys. 2017, 50 (41), 415601. DOI: 10.1088/1361-6463/aa87a0
16. Boumans, P. Theory of Spectrochemical Excitation; Springer, 1966.
17. Wang, Z.; Wang, J. An Experimental Study on the Fire Characteristics of New and Aged Building Wires Using a Cone Calorimeter. J. Therm. Anal. Calorim. 2019, 135, 3115–3122. DOI: 10.1007/s10973-018-7626-8
18. Chen, J. Z.; Bai, J. N.; Song, G. J.; et al. Enhancement Effects of Flat-Mirror Reflection on Plasma Radiation. Appl. Optics 2013, 52 (25), 6295–6299. DOI: 10.1364/AO.52.006295
19. Tsuyuki, K.; Miura, S.; Idris, N.; Kurniawan, K. H.; Lie, T. J.; Kagawa, K. Measurement of Concrete Strength Using the Emission Intensity Ratio Between Ca (II) 396.8 nm and Ca (I) 422.6 nm in a Nd: YAG Laser-Induced Plasma. Appl. Spectrosc. 2006, 60 (1), 61–64. DOI: 10.1366/000370206775382668
20. Marpaung, A. M.; Hedwig, R.; Pardede, M.; et al. Shock Wave Plasma Induced by TEA CO2 Laser Bombardment on Glass Samples at High Pressures. Spectroc. Acta Pt. B-Atom. Spectr. 2000, 55 (10), 1591–1599. DOI: 10.1016/S0584-8547(00)00264-0
21. Bredice, F. O.; Rocco, H. O. D.; Sobral, H. M.; Villagrán-Muniz, M.; Palleschi, V. A New Method for Determination of Self-Absorption Coefficients of Emission Lines in Laser-Induced Breakdown Spectroscopy Experiments. Appl. Spectrosc. 2010, 64 (3), 320–323. DOI: 10.1366/000370210790918454
22. Galmed, A. H.; Steenkamp, C. M.; Ahmed, I.; et al. Matrix Effect Impact on Measuring Hardness of Metals Bombarded by Accelerated Ions Using Laser Induced Breakdown Spectroscopy. J. Laser Appl. 2020, 32 (1). DOI: 10.2351/1.5122751
23. Hou, J. J.; Zhang, L.; Zhao, Y.; et al. Mechanisms and Efficient Elimination Approaches of Self-Absorption in LIBS. Plasma Sci. Technol. 2019, 21 (3), 034016. DOI: 10.1088/2058-6272/aaf875
24. Momcilovic, M.; Petrovic, J.; Ciganovic, J.; et al. Laser-Induced Plasma as a Method for the Metallic Materials Hardness Estimation: An Alternative Approach. Plasma Chem. Plasma Process. 2020, 40, 499–510. DOI: 10.1007/s11090-020-10063-5

Xiaomei Lin and Guanyang Wang are with the School of Electrical and Electronic Engineering, Changchun University of Technology, Changchun, China. Jingjun Lin and Yutao Huan are with the School of Mechanical and Electrical Engineering, Changchun University of Technology, Changchun, China. Direct correspondence to Jingjun Lin at 1124270941@qq.com or Yutao Huang at huangyutao@ccut.edu.cn ●