Article
Spectroscopy
Spectroscopy
Spectroscopy Is Applied Quantum Mechanics, Part I: The Need for Quantum Mechanics
Author(s):
In this first part of a multipart series, columnist David Ball reviews the failures of classical mechanics that necessitated the development of a new theory of nature.

Science (n): The process of learning about the natural universe by observation andexperiment.
At least, that's what I teach my students, usually on the first day of class. Science is, ironically, difficult to define precisely, but this is the definition I've developed over the years. If anyone has any suggestions for improvement, let me know. I also teach my students that if theory doesn't agree with nature, there are two choices: change the theory, or change nature. Unfortunately, all attempts to change nature have failed. Our only choice? Change the theory. That, in a nutshell, is why we have quantum mechanics. In this first part of a multipart series, we will review the failures of classical mechanics that necessitated the development of a new theory of nature.
Isaac Newton first published his three laws of motion in 1687, in Principia Mathematica. Although they took some time to be accepted, ultimately it was realized that the three laws did indeed accurately describe the motion of objects. The predictive nature of the three laws was so good that it inspired Pierre-Simon Laplace, the famed French mathematician and astronomer, to famously remark that if we knew the position and velocity of every bit of matter, we could predict the future of the universe.

David W. Ball
However, as the 19th century progressed, certain experiments produced results that could not be explained in terms of the understanding of nature that science held at that time. Here, we will review the main issues.
Spectroscopy
In 1860, Robert Bunsen (of Bunsen burner fame) and Gustav Kirchhoff (of Kirchhoff's laws fame) invented the spectroscope (Figure 1). One of the noteworthy findings, noted almost immediately, was that some samples absorbed only particular colors of light. Bunsen and Kirchhoff quickly concluded that the colors of light absorbed (or emitted, depending upon the temperature of the sample) were specific to the elements in the sample, and less than a year later discovered the elements rubidium and cesium by noting new colors of light not previously associated with any other known element.

Figure 1: One of the earliest models of spectroscope (1).
Of course, there is an obvious question — why? Why do different elements absorb or emit only certain colors of light? Also, what determines which colors of light are absorbed or emitted? Unfortunately, nothing in Newton's laws of motion, or law of gravity, or even Maxwell's equations of electromagnetism (published in 1861) could explain why.
The element hydrogen had a particularly simple spectrum. This was arguably defensible, because it was known even then that hydrogen was the simplest element. However, there was no theoretical explanation for the particular colors that hydrogen did absorb or emit (Figure 2).

Figure 2: Emission spectrum of the element hydrogen. The leftmost line is in the far violet and sometimes is not detectable by the human eye. A major issue in the late 19th century was exactly why these colors, and no others, are emitted by hydrogen.
The simplicity of the spectrum did inspire some investigation, and in 1885, Swiss mathematician Johann Jakob Balmer announced that the wavelengths of the lines of light emitted or absorbed by hydrogen could be predicted by the following formula:
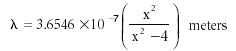
where x was 3, 4, 5, and 6 for the lines going from red to violet, respectively. This formula is more well known in its reciprocal form as
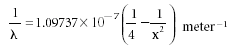
Collectively, these four lines of light are called the Balmer series of hydrogen, in Balmer's honor. Three years later, a Swiss physicist, Johannes Rydberg, generalized Balmer's formula to include light emitted in other regions of the spectrum; his formula is
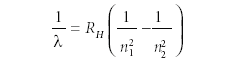
where n1 and n2 are integers such that n1 is less than n2, and RH is a constant now known as the Rydberg constant. This formula predicts the wavelengths of all light, in any region of the spectrum, given off by hydrogen.
Great — we can now predict the spectrum of hydrogen. But the main question still remains: why does this work? What theory of nature can we invoke to explain this? Unfortunately, the understanding of nature at that point in history was insufficient to explain spectra and, no matter how simple, the spectrum of hydrogen.
The Photoelectric Effect
Although it had been observed earlier, the first details of a phenomenon called the photoelectric effect were published by Heinrich Hertz in 1887. The photoelectric effect is simple: when light of a certain wavelength was shined on a metal surface, electrons were emitted. Hertz apparently reported his observations but did not pursue the topic, nor did he attempt any explanation of the phenomenon.
The most detailed observations of the photoelectric effect were made by Philip von Lénárd in 1902. He showed that the energy of the emitted electrons (which he called "cathode rays") was inversely proportional to the wavelength of the light used to promote emission, but independent of the intensity of the light. It also was shown that the intensity of the cathode ray emission was dependent upon the intensity of light used.
This was all acceptable information, but the fundamental question remains — why? Why did these effects appear? One of the more questionable observations had to do with the intensity of the light used. If light were a wave (see below), then the energy of the wave is related to its intensity. This suggests that the more intense the light source, the greater the energy of the emitted cathode rays (electrons). However, it was demonstrated that intensity was not related to the energy of the emitted electrons; rather, the wavelength of the light used determined the energy of the emitted electrons. This did not make sense in terms of the understanding of nature at the time. Once again, the theories of nature at that time were insufficient to explain a measurable phenomenon.
The Nature of Light and Blackbody Radiation
The nature of light is a topic that has been covered recently in this column; readers interested in details can refer to earlier installments (2). The issue of whether light is a particle or a wave supposedly was settled in the early 1800s when English polymath Thomas Young used the so-called double-slit experiment to produce interference fringes, evidence that light was a wave. So the matter rested.
In the mid 1800s, interest in the luminescent properties of blackbodies arose. A blackbody is a perfect absorber of light. Because absorption and emission were recognized as opposite processes, another definition is that a blackbody is a perfect emitter of light as well. Blackbodies emit light when hot, so scientists eventually studied the properties of light emitted from hot blackbodies.
At first, one might think that the emission of a wave of light of any wavelength is equally probable, so a plot of intensity emitted versus wavelength of light ought to be a straight horizontal line (marred by peaks and valleys due to experimental error and the imperfection of real materials). However, experimental measurements showed a variation in the intensity versus wavelength plot. The actual behavior looked something like that shown in Figure 3. The intensity is zero at very small wavelengths, and then increases to some maximum whose wavelength depends upon temperature, and then decreases asymptotically as the wavelength of light increases.
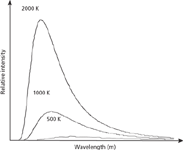
Figure 3: Plots of the intensity of light emitted versus wavelength for a hot blackbody. Although the general curvature is similar, the actual intensity pattern depends upon the temperature of the blackbody.
Why does emission of light from blackbodies follow this behavior? Scientists at the time could not explain the behavior of blackbody radiation (sometimes called cavity radiation) using the theories of the time. There were some observations, however.
The wavelength of maximum intensity λmax is not only temperature-dependent, but it follows a simple mathematical relationship:
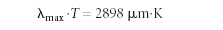
This relationship is called Wien's displacement law, after Wilhelm Wien, the German physicist who first annunciated it in 1893.
The total power radiated per unit area of blackbody, j, is related to the area under the blackbody intensity curve. It was found experimentally by Jožef Stefan and thermodynamically by Ludwig Boltzmann that the total power radiated per unit area is proportional only to the fourth power of the temperature:
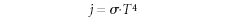
The proportionality constant σ is called the Stefan–Boltzmann constant, and its value is 5.671 × 10–8 W/m2 K4.
These relationships were verifiable experimentally, but the question remains: why do these relationships occur? There were some attempts to derive theoretical explanations for the behavior of blackbody radiation. Wien (of Wien displacement law fame) derived an expression in 1896 that became known as Wien's distribution law (not to be confused with his displacement law). He derived the following expression:
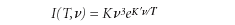
where T is the absolute temperature, ν is the frequency of the light, and K and K' are constants to fit the experimental data. Wien's distribution law was only partially successful, agreeing with experiment on the high-frequency (low-wavelength) end of the blackbody intensity curve.
A different attempt was made by English physicist John William Strutt, 3rd Baron Rayleigh. In 1900, he derived a different expression that was refined in 1905 by fellow British physicist James Jeans. Their function is
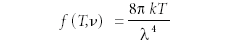
where k is Boltzmann's constant and λ is the wavelength of the light emitted. This function, known as the Rayleigh–Jeans law, also was imperfect: it agreed well on the high-wavelength (low-frequency) side of the experimental blackbody radiation distribution curve.
So what we have at this point is multiple attempts but no satisfactory theoretical understanding of why blackbody radiation behaves the way it does. The theories of nature at that time were insufficient to explain this phenomenon.
Low-Temperature Materials Behavior
In the late 1800s, Dutch physicist Heike Kamerlingh Onnes pioneered the study of materials at temperatures close to absolute zero. (He was, in fact, the first person to liquefy helium, in 1908.) Upon determining the heat capacity C of various materials at various temperatures, it was found that the specific heat capacities approached zero as the temperature approached zero. Furthermore, as one slowly increased the temperature, the heat capacity increased and was proportional to the third power of the absolute temperature:
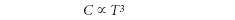
Why? We understand now that having C approach zero must be the case, thanks to the third law of thermodynamics. But why the third-power dependence upon absolute temperature? Scientists couldn't explain why this must be so.
The Resolution: Prelude to Part II
The reason that these phenomena could not be explained was because the physical theories of nature at that time were incomplete. For the first time, scientists were probing matter at the atomic level. What we realize now is that atoms behave slightly differently than bulk matter, and so different physical theories are needed to explain them. (Rest assured that today's science does have adequate theoretical explanations for all of the phenomena presented here!)
To explain these phenomena, scientists needed to understand two things: first, that light acts like a particle in some of its properties; and second, that electrons and other tiny particles of matter act like waves in some of their properties. Until the false dichotomy of "particle or wave" was removed, science couldn't explain these phenomena. Science needed quantum theory and quantum mechanics. Ultimately, if you do spectroscopy, you're doing quantum mechanics.
In the next installment, we will see how these new theories, and the insights that come with them, apply to the phenomena discussed here.
A similar but more detailed version of this story is given in my textbook Physical Chemistry (3). It is my opinion that most textbooks in physical chemistry don't devote sufficient space to the failings of classical mechanics that required the development of quantum mechanics — that was one reason I wrote my own book!
David W. Ball is a professor of chemistry at Cleveland State University in Ohio. Many of his "Baseline" columns have been reprinted in book form by SPIE Press as The Basics of Spectroscopy, available through the SPIE Web Bookstore at www.spie.org. His most recent book, Field Guide to Spectroscopy (published in May 2006), is available from SPIEPress. He can be reached at d.ball@csuohio.edu his website is academic.csuohio.edu/ball.
References
(1) W.J. Rolfe and J.A. Gillet, A Handbook of Natural Philosophy (Woolworth, Ainsworth, & Co., Boston, Massachusetts, 1868).
(2) D.W. Ball, Spectroscopy 21(6), 30–33 (2006).
(3) D.W. Ball, Physical Chemistry (Brooks/Cole Publishing Co., Forest Lodge, California, 2002).
Newsletter
Get essential updates on the latest spectroscopy technologies, regulatory standards, and best practices—subscribe today to Spectroscopy.





