A Full Spectroscopic Study of Pr:YLF Crystals Used in Lasers
A Judd-Ofelt analysis, quantifying the optical intensities of the rare-earth ions’ 4f-4f manifolds, is conducted in detail, based on the absorption spectrum from the visible to infrared spectral range for a Pr:YLF crystal. The values of key parameters, such as radiation lifetime, radiation transition probability, and branching ratio, are obtained accordingly. Additionally, the emission spectra are measured with precaution against reabsorption, and the emission cross sections are calculated using the Füchtbauer-Ladenburg formula, applied frequently in precise emission spectrum analysis, with the radiation lifetime derived from the Judd-Ofelt model. The results obtained regarding the orange and deep red spectral range are higher than those from previous reports, providing further insights into the lasing mechanism at the concerned wavelengths.
Highly-efficient visible lasers have attracted substantial research interest in recent years (1–5). Praseodymium (Pr), thanks to its energy structure (providing a direct down-conversion mechanism pumped by the booming blue diodes coming out recently) has become the most promising laser gain ion in the visible range (6). As for the host material, yttrium lithium fluoride (YLF), a positive uniaxial with tetragonal structure of scheelite type (7), is well-known for its superior physical and optical properties. The Mohs hardness of YLF is 4~5 (8), with a relatively high thermal conductivity (6 W·m-1·K-1 [8]) and expansivity (13×10-6 K-1 for a-axis, and 8×10-6 K-1 for c-axis [8]), facilitating its wide application in high-power lasers (9,10) and the Kerr lens mode-locking technique (11). In particular, Pr-doped YLF (Pr:YLF), as a fluoride crystal, is characterized by its low phonon energy (460 cm-1), compared with its oxide counter- parts ( 550 cm-1 for praseodymium-doped yttrium aluminum perovskite (Pr:YAP) with the formula Pr:AlYO3, leading to a weaker non-radiative multi-phonon relaxation, and thus better lasing performance.
The spectroscopic characteristics of the crystal used in a laser being critical to laser performance, and involve both the absorption and emission spectra, respectively. The former can be derived through a Judd-Ofelt analysis, and the latter by employing the Füchtbauer-Ladenburg formula (14), both of which are analytical calculation procedures. Judd-Ofelt theory, which was proposed independently by B. R. Judd (12) and G. S. Ofelt (13) in 1962, describes the possibilities of radiative emissions in the 4fN configuration, and is extensively adopted to determine the radiative lifetime and branching ratio of each emitting level for trivalent rare-earth-doped laser materials (15–24). On the other hand, the Füchtbauer-Ladenburg formula was derived from the fluorescence analysis correlated with an electronic transition (25), and has gained wide application in the determination of emission cross sections of laser gain media (22–24). To optimize the analytical procedure for characterizing Pr:YLF crystal, various improvements have been made in both theory and experiment. For example, Dunina and associates modified the Judd-Ofelt theory by considering the finite 4f-5d energy (26), Kornienko and colleagues introduced third order perturbation theory into the line intensity calculation (27,28), Goldner and associates adopted a normalized least-squares method in the fitting process to obtain a stable output (29), and Quimby and colleagues used the experimental branching ratios, other than theoretical ones, in deriving emission cross sections (30).
In this paper, the spectroscopic properties of a Pr:YLF laser crystal (31) were studied by analyzing the polarization-dependent absorption and emission spectra, accordingly. Radiation transition probabilities, theoretical branching ratios, and intrinsic radiation lifetimes were obtained from the absorption spectrum analysis. Experimental branching ratios were demonstrated by emission spectrum analysis. In addition, by introducing the intrinsic radiation lifetimes derived from Judd-Ofelt analysis and experimental branching ratios into the Füchtbauer-Ladenburg formula, emission cross sections are also reported.
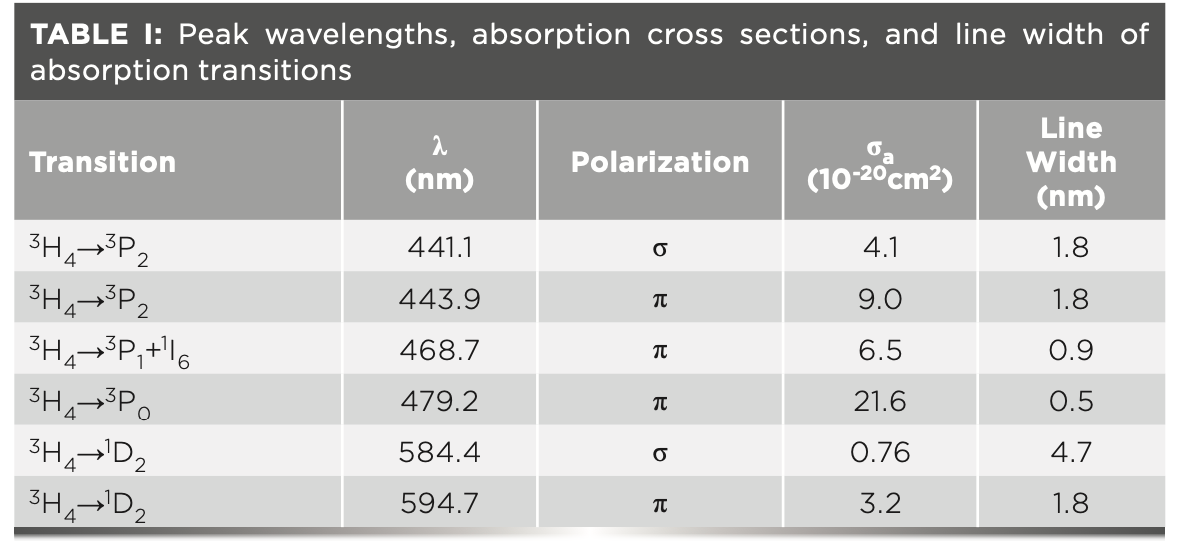
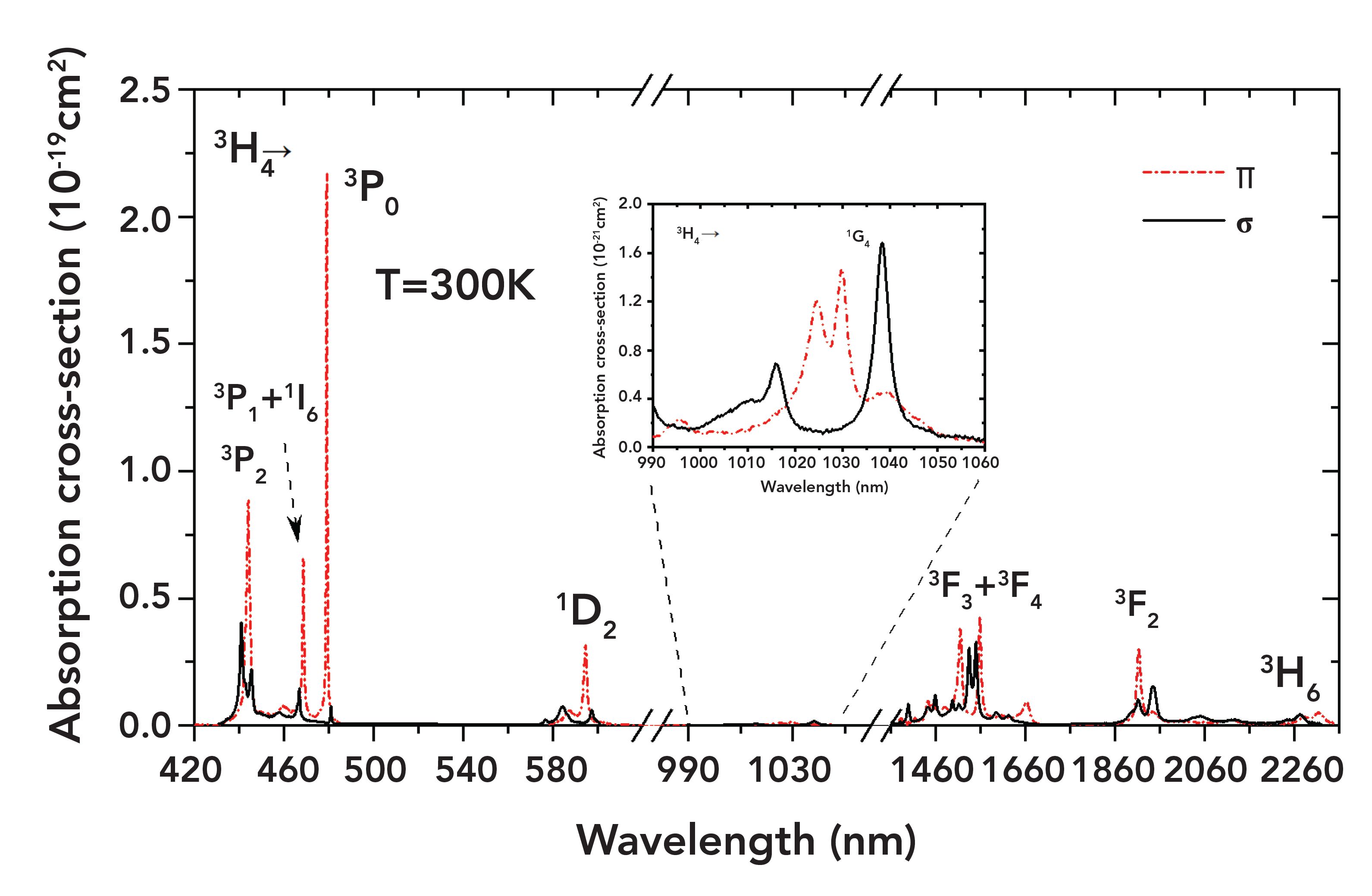
FIGURE 1: Absorption cross section from visible to infrared spectral range.
Experiment and Analysis
Absorption Spectrum
For the absorption spectrum measurement, to reduce the absorption effect of the crystals, a relatively low doping concentration of 0.3 atomic percent (at.%) was adopted. The absorption spectrum was measured using a Lambda 1050 spectrophotometer (PerkinElmer). The data sampling interval was set at 0.05 nm. Polarizing prisms were placed in the sample path and the reference path, to measure absorption spectra in both polarizations.
The absorption coefficients for different wavelengths (a) were calculated from the absorption spectrum using equation 1:
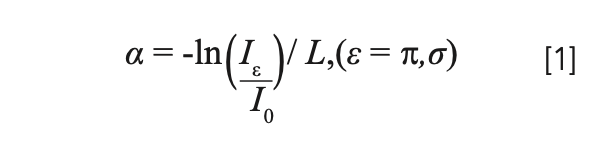
where L is the length of the sample, and Iε and I0 are the light intensities of the sample light path and the reference light path, respectively. The crystal absorption cross sections can then be calculated by equation 2:
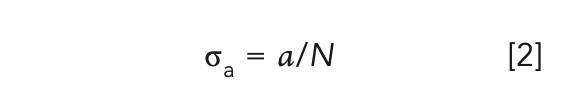
where N = 4.2 × 1019 cm-3 is the lattice concentration of Pr3+ in the crystal. The calculated absorption cross sections are shown in Figure 1.
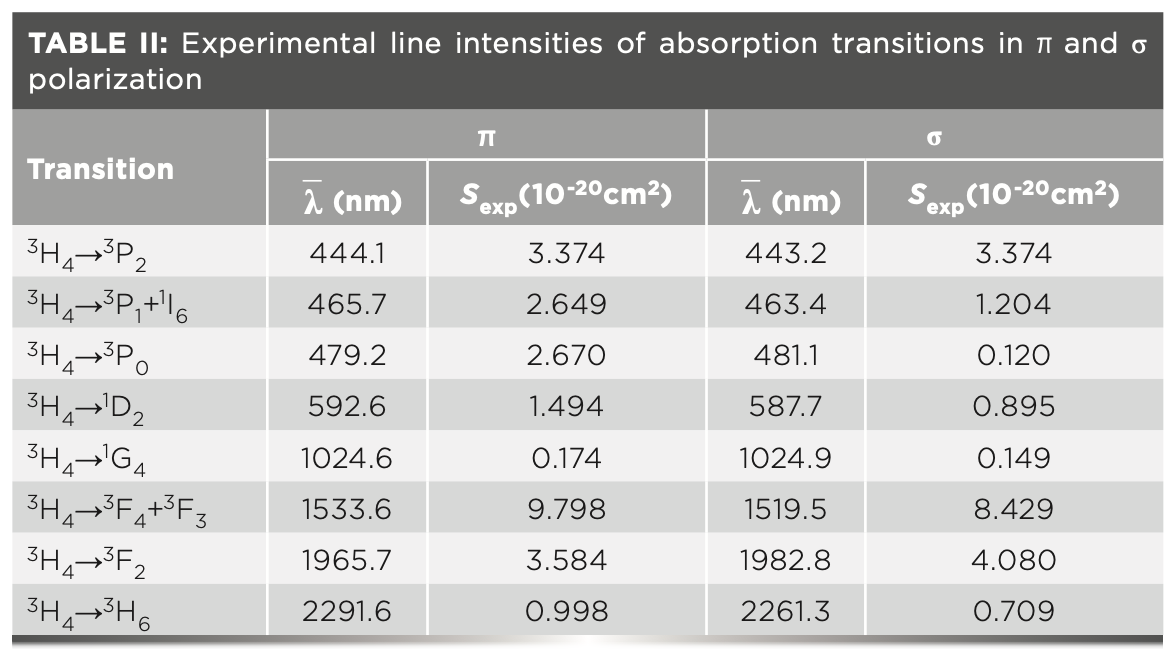
Table I lists the peak wavelengths, absorption cross sections, and line widths of major transitions in the visible spectral range. There are three absorption bands in the blue spectral range, corresponding to transitions 3H4→3PJ (J = 0,1,2). Transition 3H4→3P0, peaking at 479.2 nm, possesses the largest absorption cross section (2.16 × 10-19 cm2) with the narrowest line width (0.5 nm). Transition 3H4→3P2 (443.9 nm) has a relatively large absorption cross section (9.0 × 10-20 cm2) and line width (1.8 nm). In addition, there are four major absorption bands in the infrared spectral region, 3H4→ 1G4 at approximately 1 μm, 3H4→3F3+1F4 at 1.5 μm and 3H4→3F2 at 1.9 μm, and 3H4→3H6 at 2.3 μm, respectively. From the calculation results ranging from the visible to the infrared regime, it can be concluded that the absorption cross sections in π polarization are generally larger than their σ counterparts.
According to the Judd-Ofelt theory (12,13), the experimental line intensity can be expressed as:
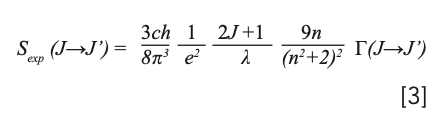
where J and J’ are the total angular momentum quantum numbers of the initial and final energy levels, respectively, c is the speed of light in a vacuum, h is Planck’s constant, n is the refractive index, and Γ(J→J’) is the ›integral absorption cross sections. The values of the polarization-dependent line intensities are listed in Table II, in which λ is the average of measured wavelength weighted by the accordance absorption cross sections.
The mean experimental line intensity is defined as
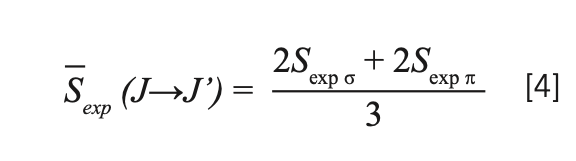
then the intensity parameters Ω2,4,6 can be achieved using the least squares fitting, performed as
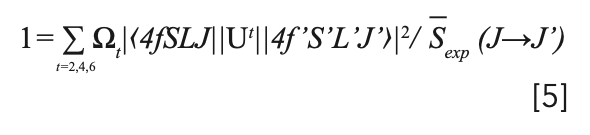
where Ut are doubly reduced matrix elements in accordance with specific rare-earth ions. In our case, we use matrix elements evaluated and given by Weber for Pr3+ in LaF3 (32). For the energy level of Pr3+, the energy of the 4f-5d configuration is very close to that of the 4f configuration. The line intensity from the ground-state level to the highest emitting level is larger than the calculated value, so the hypersensitive transition 3H4→3P2 is always neglected in the fitting procedure (33). The results of the intensity parameters are Ω2=1.2×10-20 cm2, Ω4=6.64×10-20 cm2, and Ω6=5.16×10-20 cm2. Because the line intensity parameters Ω2,4,6 are material dependent, the calculated Ω2,4,6 values are applicable to all transitions of the same material. Considering that the magnetic dipole component for Pr3+ could be neglected (34), the calculated line intensity Scalc is given by the electric dipole line intensity, shown as below:
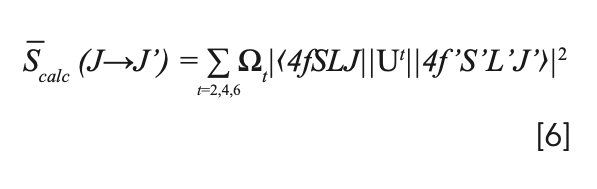
The experimental and calculated values of line intensities, and doubly reduced matrix elements of absorption transitions, are shown in Table III.
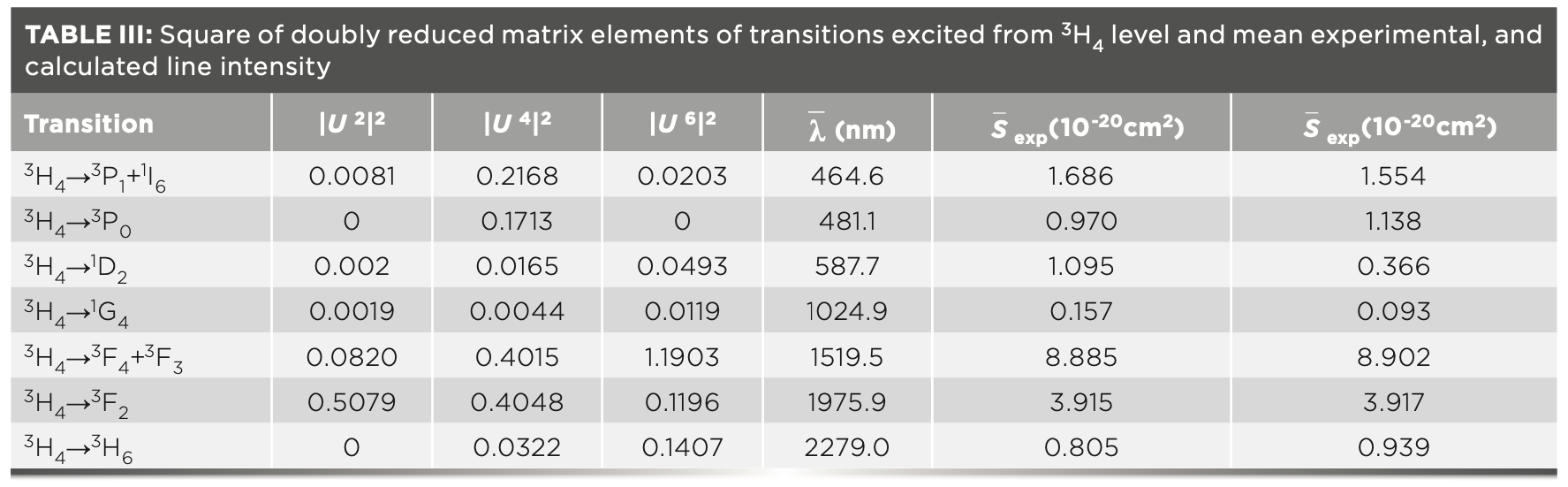
To verify the calculation accuracy, the root-mean-square (RMS) error is calculated, and its expression is given by:
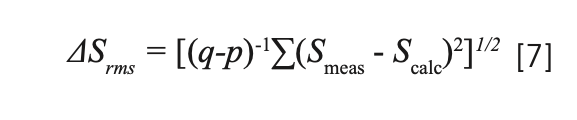
where q is the number of required parameters and p is the number of spectral bands to be analyzed. Combined with our calculation, where p = 7 and q = 3, the calculated ΔSrms= 0.47×10-20 cm2, the experimental line intensity RMS ΔS = 3.78×10-20 cm2, and ΔSrms/ΔS = 12%, which is within the normal scope (5%~25%) (35).
Using Scalc (J→J’), the radiation probability of different transitions A(J→J’) can be obtained by:
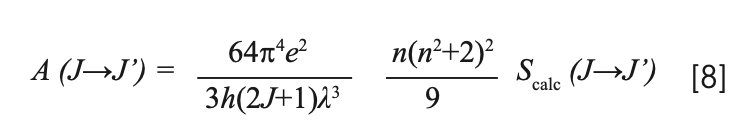
For transitions from the same excited state, the theoretical branching ratios βcalc can be obtained by:
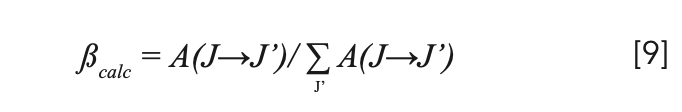
The intrinsic radiation lifetime is defined as the reciprocal of the sum of all radiation transition probabilities from the same energy level, shown as equation 10. The radiation transition probabilities, theoretical branching ratios, and intrinsic radiation lifetimes of 3P0, 3P1, and 1I6 level are listed in Table IV.
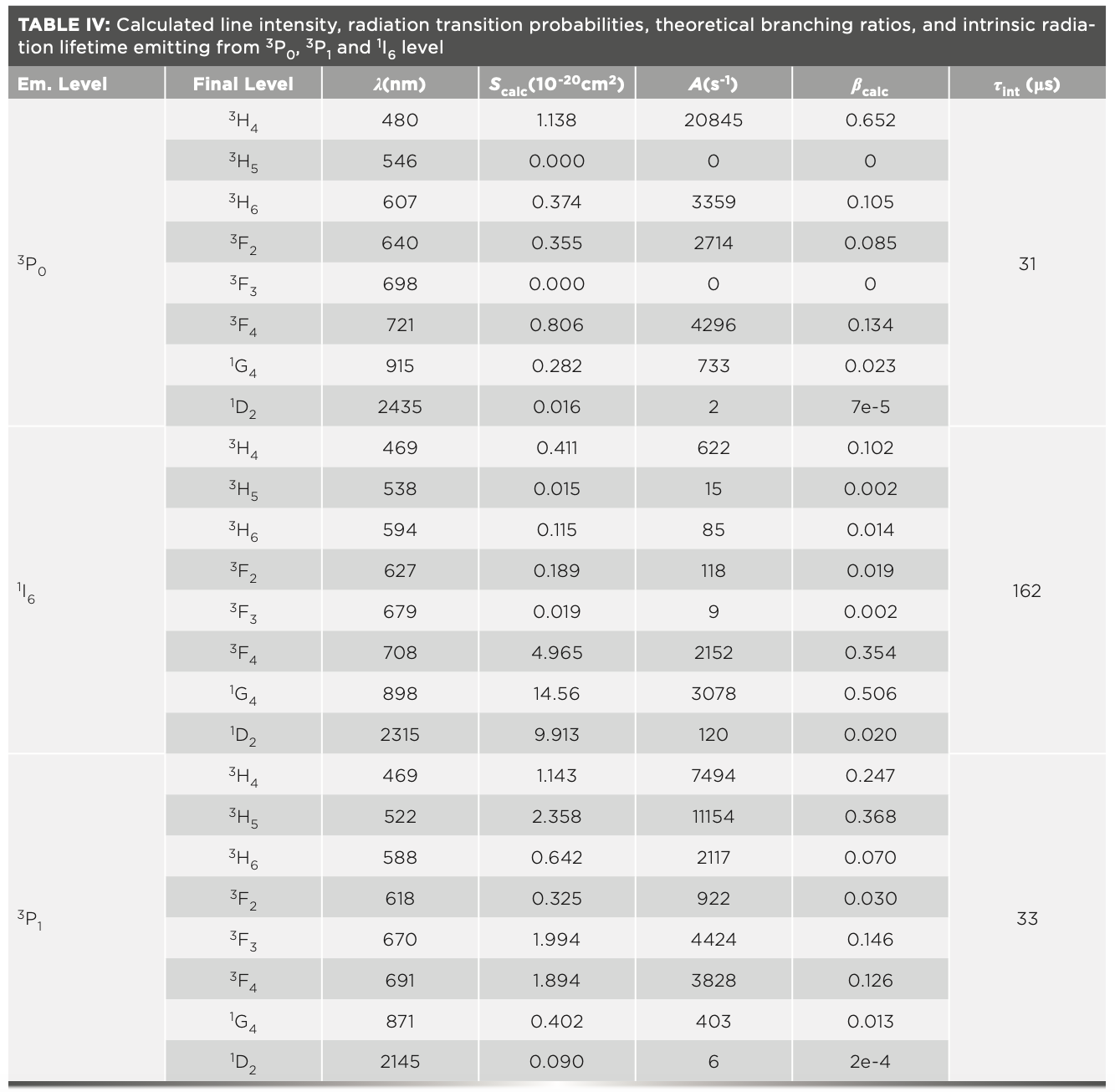
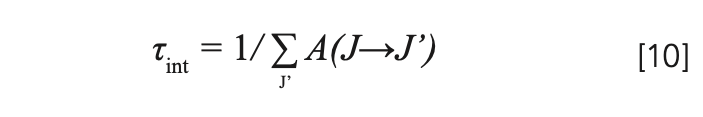
Because electrons residing in 3P0, 3P1, and 1I6 levels obey the Boltzmann distribution, the radiation lifetimes of those levels must be considered simultaneously. The effective radiation lifetime of the upper level is the average of the intrinsic radiation lifetimes of 3P0, 3P1, and 1I6 level, according to the distribution of the number of electrons (36,37), given by
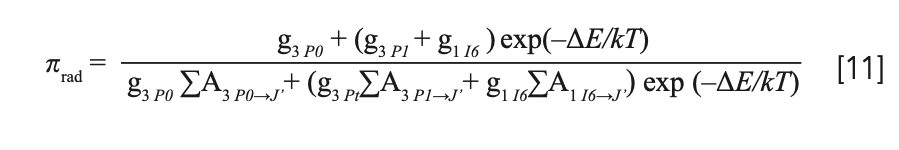
where gk (k = 3P0, 3P1, and 1I6) is the degeneracy of corresponding energy level, being 1, 13, and 3, respectively, and ΔE is the energy difference between the 1I6+3P1 level and the 3P0 level.
By substituting the radiation transition rates listed in Table IV into equation 11, the effective radiation life- time of the upper energy level was calculated to be 49 μs, with a derivation of ±10%, derived from the error of Judd-Ofelt theory. The effective radiation lifetime of the upper level τrad and fluorescence radiation lifetime τflu is related by
where Wnonrad is the non-radiative transition rate, including multi-phonon transition (3PJ →1D2), up-conversion, cross relaxation (3P0→1G4 and 3H4→1G4, 3P0→1D2 and 3H4→3H6 [38,39]), and so on. It could be deduced that τflu decreases with the increasing of Wnonrad, which could be reduced by lowering the doping concentration of rare earth ions.
Emission Spectrum
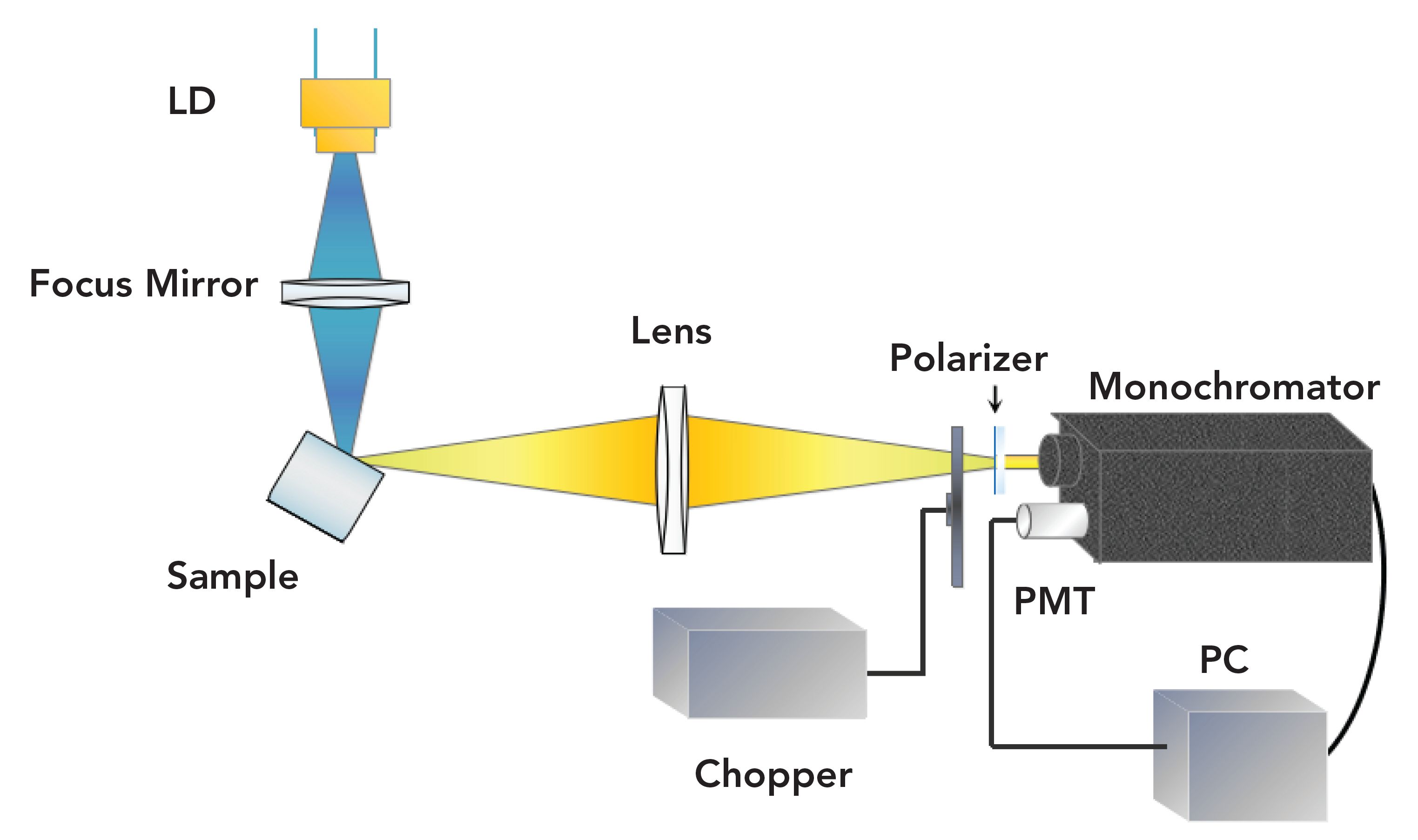
In terms of the emission spectrum, the measurement device is shown in Figure 2. Using an InGaN laser diode (LD) with a wavelength of 444 nm as the pump source, the driving current was set at 100 mA, and the corresponding output power was about 30 mW. The pump light is focused into the sample, whose emitting spectrum was converged into the slit of the monochromator through a lens with large aperture and long focal length. A photo-multiplier (PMT, Hamamatsu R3896) was employed. The phase-locked amplification technique, implemented with a chopper, was utilized to extract the small signal in the noise. A Mercury vapor lamp (color temperature 2900 K) was used for wavelength correction and calculation of spectrometer transfer function. The experiments were conducted at room temperature.
FIGURE 2: Experimental setup of emission spectrum.
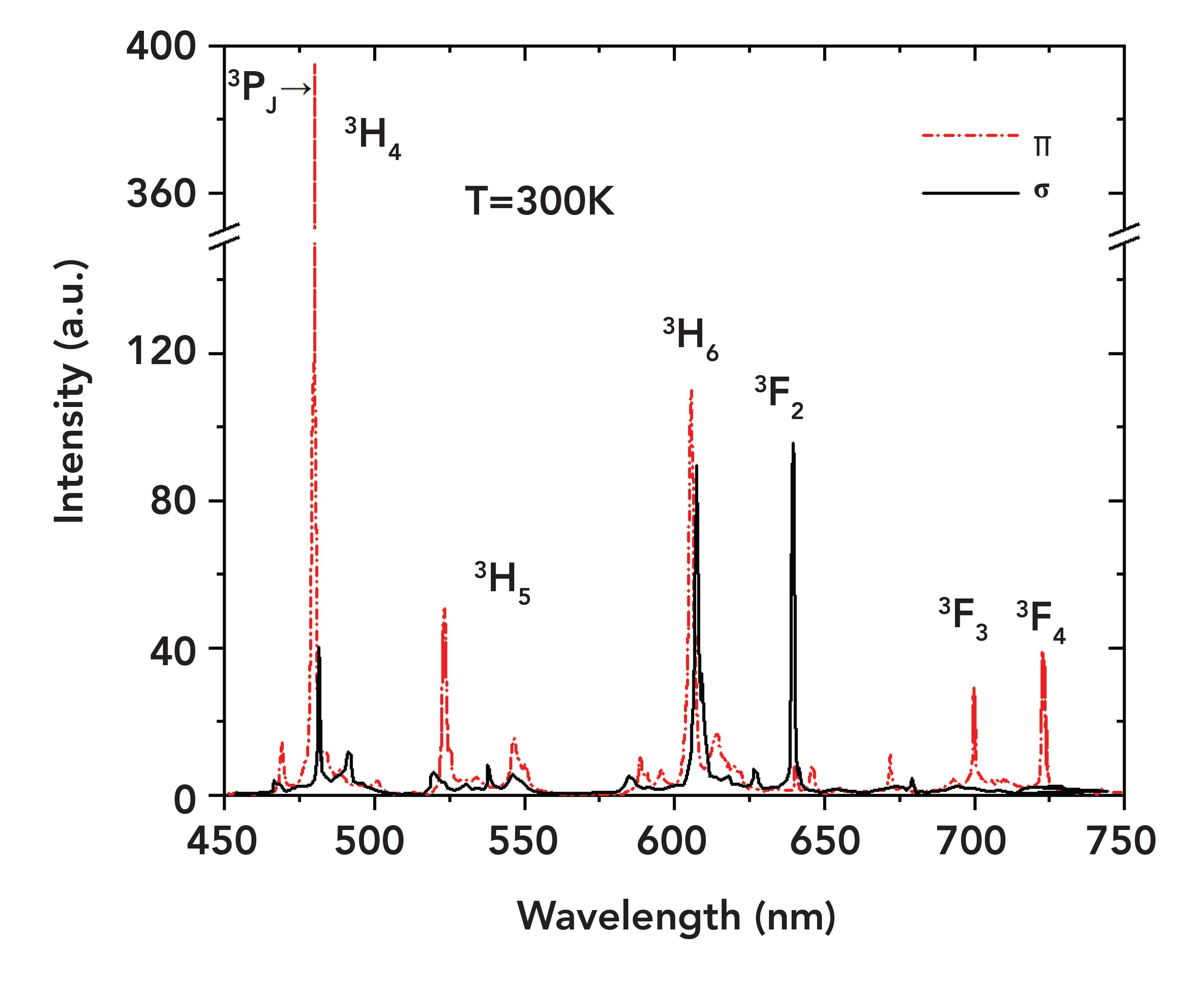
The emission spectrum, showing multiple transitions in the visible range, is shown in Figure 3. The main transitions of 3P0→ 3H4, 3P1→ 3H5, 3P0→ 3H6, 3P0→ 3F2, 3P0→3F3, and 3P0→3F4 correspond to the peak wavelengths of 479.4, 522.6, 607.2, 639.5, 697.7, and 720.7 nm. Blue 3P0→3H4 (479.4 nm), green 3P1→3H5 (522.6 nm), and deep red 3P0→3F3 (697.7 nm) and 3P0→3F4 (720.7 nm) are mainly π-polarized, while red light 3P0→3F2 (639.5 nm) is mainly σ-polarized.
FIGURE 3: Emission spectrum between 450 nm and 750 nm.
A stimulated emission cross section is usually treated as a parameter for evaluating the gain performance of the laser material. The methods for calculating the stimulated emission cross section mainly include the reciprocity method (40), the Füchtbauer-Ladenburg formula (14), and the reverse of the laser performance (41). The first method is applicable to quasi-three-level transitions with clarified ground-state level distribution, the second method is suitable to either quasi-three-level or four- level transitions with a highly accurate emission spectrum, and the third method is strongly dependent to the measurements of laser experiments. Based on the emission measurement results in the 450–750 nm spectral range, the Füchtbauer-Ladenburg formula was employed to calculate the stimulated emission cross section.
For a uniaxial laser crystal with two polarization directions, the Füchtbauer-Ladenburg formula can be written as (42):
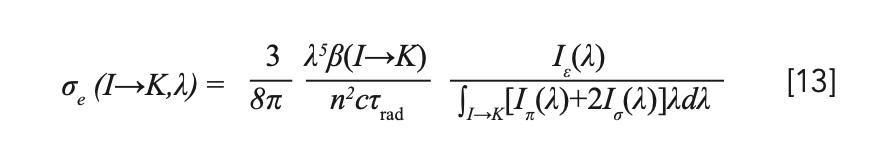
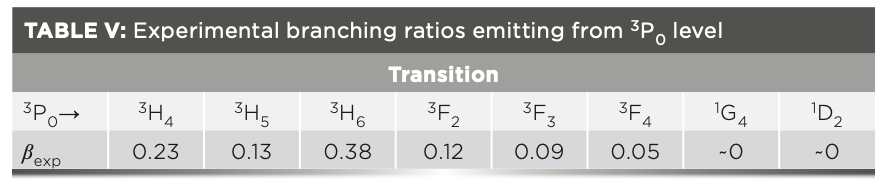
For the branching ratio β in equation 13, experimental value βexp was employed, instead of the theoretical value calculated based on Judd-Ofelt theory, owing to the small energy differences between the 4f and 4f–5d configurations in Pr3+. βexp was expressed by:
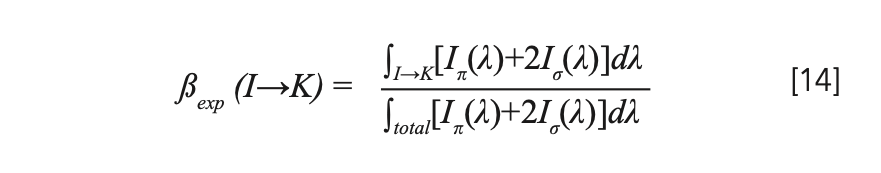
where the denominator is an integral over the entire fluorescence spectrum. The calculation results are shown in Table V.
By substituting equation 14 into equation 13, the numerator of βexp can be eliminated; therefore, equation 13 could be simplified to
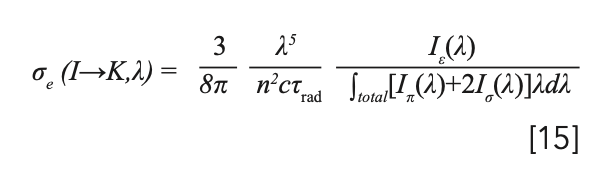
The results are displayed in Figure 4, with the emission cross sections and line widths of the main transitions listed in Table VI.
FIGURE 4: Emission cross sections between 450 and 750 nm.
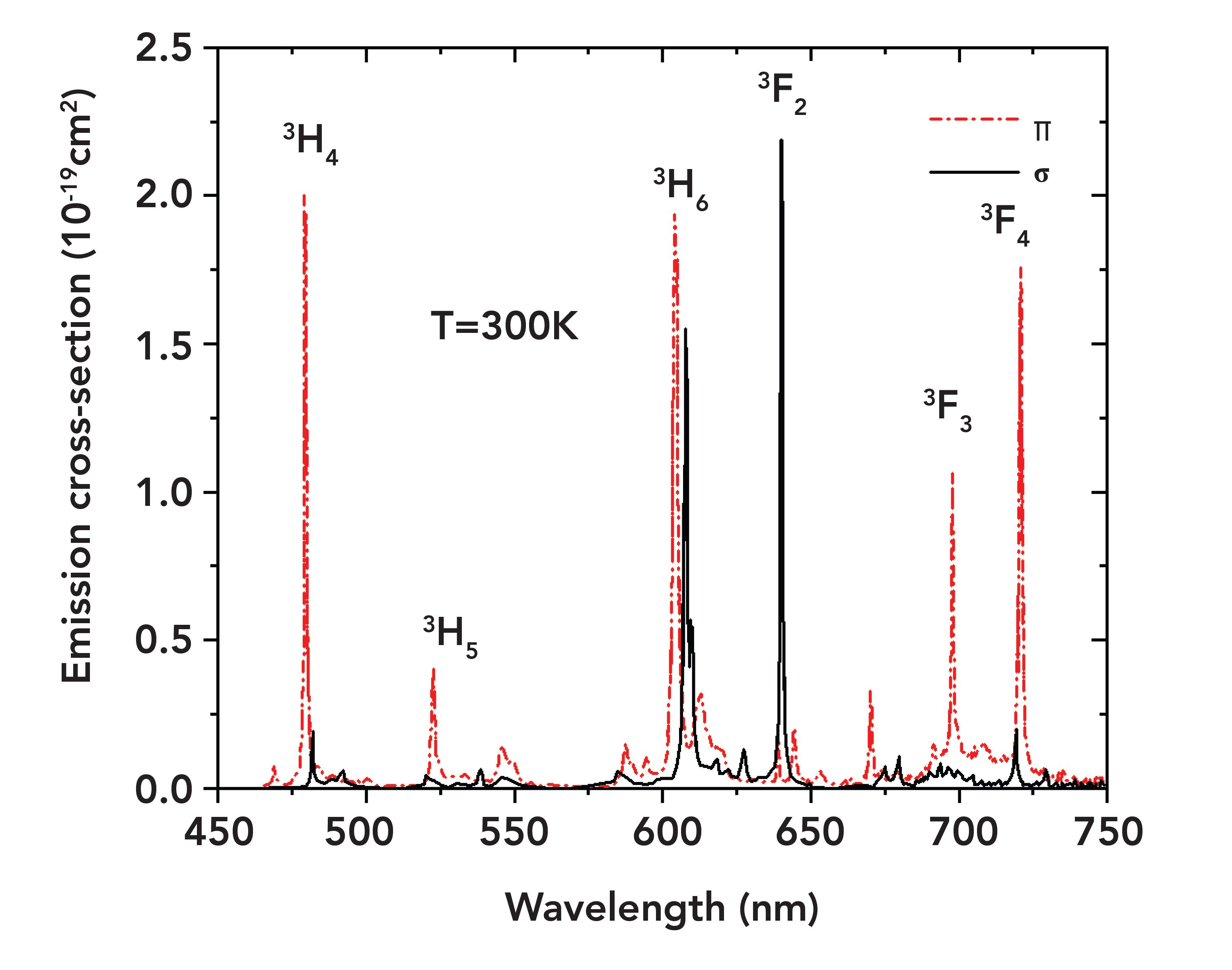
Note that the emission cross sections in π polarization are generally larger than those in σ polarization. The σ-polarized 640 nm transition has the largest emission cross section, reaching 22.3 x 10-20 cm2.
It should be noted that some results different from those of previous reports (43,44) were demonstrated. For the orange transition at 607 nm, a slightly larger value was achieved (1.57×10-19 cm2, compared with 1.4×10-19cm2 [43]), thanks to the precaution to avoid the ground-state reabsorption process 3H4→1D2. In terms of the transitions in the deep red spectral region, the emission cross sections of 698 and 721 nm were 1.07×10-19 cm2 and 1.78×10-19 cm2, about 2x larger than those in (43) (0.5×10-19 cm2 and 0.9×10-19 cm2). Because emission cross sections are proportional to the corresponding laser thresholds, further investigations in the deep red range laser thresholds can be used to verify the accuracy of the emission cross sections.
Conclusion
The spectroscopic properties of a Pr:YLF laser crystal were studied theoretically and experimentally. The polarization-dependent ab- sorption cross sections at room temperature from the visible to infrared spectral range were demonstrated. The maximum absorption of 21.7×10-20 cm2 peaked at 478 nm, with the narrowest line-width of 0.5 nm. In the framework of Judd-Ofelt theory, intensity parameters Ω2,4,6 were obtained when the hypersensitive transition 3H4→3P2 was excluded from the fitting procedure. For the emission spectrum, the experimental branching ratio was introduced to calculate the emission cross section using the Füchtbauer-Ladenburg formula in the visible spectral range. Results different from those seen in previous reports were obtained in both the orange and deep red spectral regions. This investigation provides insight into the spectroscopic analysis of Pr:YLF crystals used in lasers.
Acknowledgments
This work is supported by NSAF (No. U1830123), the National Natural Science Foundation of China (No. 61627802), the Natural Science Foundation of Jiangsu Province (No. BK20180460), and the High-Level Educational Innovation Team Introduction Plan of Jiangsu, China.
References
(1) W.P. Chao, Y. Qi, and W.X. Yang, Appl. Opt. 58, 6733 (2019).
(2) C. Khurmi, S. Thoday, T.M. Monro, G. Chen, and D.G. Lancaster, Opt. Lett. 42, 3339 (2017).
(3) K. Sebastian and J. Bahram, Opt. Lett. 44, 5913 (2019).
(4) S. Kajikawa, M. Yoshida, O. Ishii, M. Yamazaki, and Y. Fujimoto, Opt. Commun. 424, 13 (2018).
(5) A. V. V. Nampoothiri, F. B. A. Aghbolagh, B. Debord, F. Gerome, F. Benabid, and W. Rudolph, Appl. Opt. 56, 9592 (2017).
(6) M. Fibrich, J. Sulc, and H. Jelínková, Proc. SPIE 9342, 93421X (2015).
(7) K. Ogasawara and S. Watanabe, Advances in Quantum Chemistry (Academic Press, Amsterdam, Holland, 2008).
(8) A.A. Kaminskii, H.J. Eichler, B. Liu, and P. Meindl, Phys. Status Solidi A 138, K45 (1993).
(9) H.K. Nie, H.P. Xia, B.N. Shi, J.X. Hu, B.T. Zhang, K.J. Yang, and J.L. He, Opt. Lett. 43, 6109 (2018).
(10) B. Xu, Z. Liu, H. Xu, Z. Cai, C. Zeng, S. Huang, Y. Yan, F. Wang, P. Camy, J. L. Doualan, A. Braud, and R. Moncorgé, Opt. Commun. 305, 96 (2013).
(11) S. Ruan, J.M. Sutherland, P.M. W. French, J.R. Taylor, and B.H.T. Chai, Opt. Lett. 20, 1041 (1995).
(12) B.R. Judd, Phys. Rev. 127, 750 (1962).
(13) G.S. Ofelt, J. Chem. Phys. 37, 511 (1962).
(14) W.B. Fowler and D.L. Dexter, Phys. Rev. 128, 2154 (1962).
(15) S.W. Long, D.C. Ma, Y.Z. Zhu, S.P. Lin, and B. Wang, Opt. Mater. Exp. 6, 3354 (2016).
(16) S. Buckley, Spectroscopy 35(1), 16–21 (2020).
(17) D.K. Bradshaw, Spectroscopy 35(2), 53–56 (2020).
(18) H. Zhao, Y. Zhao, X.X. Si, J. Li, Y.X. Duan, W. Jiang, C.B. Liu, J.H. You, Z.J. Li, and Q.P. Shen, Spectroscopy 35(2), 41–52 (2020).
(19) V. Sudesh, J.A. Piper, E.M. Goldys, and R. S. Seymour, Opt. Soc. Am. B 15, 239 (1998).
(20) S. Balaji, A.D. Sontakke, R. Sen, and A. Kalyandurg, Opt. Mater. Exp. 1, 138 (2011).
(21) G.A.S. Flizikowski, V.S. Zanuto, L.A.O. Nunes, M.L. Baesso, L.C. Malacarne, and N.G.C. Astrath, J. Alloy. Compd. 780, 705 (2019).
(22) M. Olivier, J.L. Doualan, V. Nazabal, P. Camy, and J.L. Adam, J. Opt. Soc. Am. B 30, 2032 (2013).
(23) V. G. Truong, A. M. Jurdyc, B. Jacquier, B. S. Ham, A. Q. Le Quang, J. Leperson, V. Nazabal, and J. L. Adam, J. Opt. Soc. Am. B 23, 2588 (2006).
(24) M.S. Liao, L. L. Hu, Y.Z. Fang, S.Q. Xu, and L.Y. Zhang, J. Opt. Am. B 24, 1498 (2007).
(25) W. F. Krupke, IEEE J. Quantum Electron. 10, 450 (1974).
(26) E. Dunina, A. Kornienko, and L. Fomicheva, Central Eur. J. Phys. 6, 407 (2008).
(27) A.A. Kornienko, A.A. Kaminskii, and E.B. Dunina, Phys. Status Solidi B. 157, 261 (1990).
(28) A A. Kornienko, A.A. Kaminskii, and E.B. Dunina, Phys. Status Solidi B 157, 267 (1990).
(29) P. Goldner and F. Auzel, Appl. Phys. 79, 7972 (1996).
(30) R.S. Quimby and W.J. Miniscalco, Appl. Phys. 75, 613 (1994).
(31) W. Koechner, Solid-State Laser Engineering (Springer Verlag, New York, New York, 6th Ed., 2005).
(32) M.J. Weber, J. Chem. Phys. 48, 4774 (1968).
(33) Y. Wang, J. Li, Z. You, Z. Zhu, and C. Tu, J. Alloy. Compd. 502, 184 (2010).
(34) L.R. Moorthy, A. Radhapathy, M. Jaysimhadri, T.S. Rao, D.V.R. Murthy, and S.A. Saleem, Phys. B 352, 210 (2004).
(35) L A. Riseberg and M.J. Weber, Prog. Opt. 14, 89 (1977).
(36) M. Eyal, E. Greenberg, R. Reisfeid, and N. Spector, Chem. Phys. Lett. 117, 108 (1985).
(37) S. Khiari, M. Velazquez, R. Moncorgé, J. L. Doualan, P. Camy, A. Ferrier, and M. Diaf, J. Alloy. Compd. 451, 128 (2008).
(38) G. Özen, O. Forte, and B. Di Bartolo, J. Appl. Phys. 97, 013510 (2005).
(39) J. Hegarty, D. L. Huber, and W. M. Yen, Phys. Rev. B. 25, 5638 (1982).
(40) D.E. McCumber. Phys. Rev. 136, A954 (1964).
(41) E. Sani, A. Toncelli, M. Tonelli, A. Agnesi, A. Guandalini, and G. Reali, IEEE J. Quantum. Elect. 39, 971 (2003).
(42) A. Rapaport, O. Moteau, M. Bass, and L.A. Boatner, J. Opt. Soc. Am. B 16, 911 (1999).
(43) T. Gün, P. Metz, and G. Huber, Opt. Lett. 36, 1002 (2011).
(44) G. Huber, A. Richter ,and E. Heumann, Proc. SPIE 6451, 645102 (2007)
Xiang Geng, Li Li, Chen Qian, and Saiyu Luo are with the School of Electronic and Optical Engineering, at Nanjing University of Science and Technology, in Nanjing, The People’s Republic of China. Direct correspondence to: 905645238@qq.com
Tracking Molecular Transport in Chromatographic Particles with Single-Molecule Fluorescence Imaging
May 18th 2012An interview with Justin Cooper, winner of a 2011 FACSS Innovation Award. Part of a new podcast series presented in collaboration with the Federation of Analytical Chemistry and Spectroscopy Societies (FACSS), in connection with SciX 2012 ? the Great Scientific Exchange, the North American conference (39th Annual) of FACSS.
