Analytical Vibrational Spectroscopy - NIR, IR, and Raman

Molecular vibrations can be exquisitely sensitive to molecular structure, and as such, measurements detecting them have gained favor for analytical purposes. Easy-to-use instrumentation to detect molecular vibrations is well developed in the mid-infrared (IR), near-IR (NIR), and visible (Raman) regions of the spectrum. The instrumentation has been optimized for each spectral range, but the physical processes producing the spectra are all different. Deciding which technique will be the measurement of choice will depend on the sample format, the required sample environment, the available database, and which process provides the most relevant information to answer the required question. In this installment, I will attempt to guide you through the maze of choices and describe what is being measured, how it is being measured, and what the advantages and disadvantages of these techniques are.

What is being measured? Vibrations can be detected by direct absorption of light into fundamental levels, by absorption into overtones and combinations of those levels, and by scattering off those levels with lasers. The first process is called mid-infrared (IR) absorption, named as such because the vibrational energies are in the mid-IR part of the electromagnetic spectrum. While at Cornell University (Ithaca, New York), William W. Coblentz measured IR absorption spectra of a large number of organic compounds from which he was able to catalog group characteristic bands. Even though he was not the first to measure mid-IR spectra, he is credited with developing the application of IR spectroscopy for structural diagnostics (1). The second process is near-infrared (NIR) absorption, named as such because the overtones and combinations of CH, NH, and OH vibrations and their angle-deformations occur in the near-IR part of the electromagnetic spectrum. According to Jerry Workman (2), the development of NIR spectroscopy for analytical purposes goes back to about 1960 when spectra–structure correlations for a number of functional groups were documented (3). The scattering process is called the Raman effect because C.V. Raman was the first to report the phenomenon (4,5) that had actually been predicted about 5 years earlier by Smekal (6). In fact, Raman received the Nobel Prize for this work in 1928.

By its nature, a scattering experiment is quite different from any absorption experiment. In an absorption experiment, one is always ratioing the spectrum of transmitted or reflected light to the spectrum of the light source; thus the signal intensity is absolute and the spectrum should be invariable from instrument to instrument, unless there are effects of spectral resolution. On the other hand, it is very difficult to normalize the scattering intensity to absolute units; one can correct for the laser intensity, but that is only the first of the phenomena that affect the spectral intensity. One important effect is that of the laser wavelength; everything else being equal, a shorter-wavelength laser will produce a stronger signal than a longer-wavelength laser because of the physics of the scattering phenomenon itself. In addition, if the sample is colored (has an electromagnetic absorption in the vicinity of the laser wavelength), the signal will be enhanced and the relative intensities will change drastically. Another factor affecting the signal is the volume of excitation (laser-irradiated volume). Then the optics used to image the laser-irradiated volume on the entrance slit will determine the angular aperture from which the light is collected, as well as the size of the image on the slit, which will, in turn, determine how much light is detected. And if the sample is turbid or opaque, the size of the illuminated volume will be affected. To conclude this topic, one can see that it is not easy to produce a Raman spectrum representing absolute scattering intensity, thereby making the application of the technique less straightforward than the two absorption phenomena.

There also is the issue of the polarity of the photons. Who among us remembers group theory? The point is that a photon is a shorthand name for a bundle of energy described by coupled oscillating electric and magnetic fields that move through space. The smallest unit of energy (the energy of a photon or light quantum) is proportional to the reciprocal of the wavelength (λ), called the wavenumber.

The interaction between the photons and the molecules is by the coupling with the photon's electric field, which of course is a polar quantity. That means that for a molecular vibration to absorb radiation, not only must the energy of the vibration match the photon energy (v), but the vibration has to produce an electric dipole. That is why polar groups like carbonyls are strong IR absorbers. In the case of Raman scattering, there are two photons, so the molecular vibration must have even character. (Two odd photons make "even" character and will couple with a symmetric-type vibration.) That is why a vibration like a C=C double bond is strongly Raman active. The implication of this is that IR and Raman spectroscopies are complementary — vibrations that appear in one do not appear in the other if there is a center of symmetry; or, when there is no center of symmetry, a vibration tends to be strong in one effect and weak in the other.

So what about NIR absorption? Again we have an absorption process, but each photon makes a transition to more than one vibration so that the energy range is high enough to reach the NIR range of the spectrum. The observed spectra are attributed to overtones of CH, NH, and OH stretches and combinations of the stretches and angle deformations; the region between 700 and 1600 nm is typically assigned to overtones (14,300–6250 cm^-1), and the region between 1600 and 2500 nm to combinations (6250–4000 cm^-1). Because there is only one photon, the symmetry of the sum of the vibrations has to be compatible with the odd character of the electric field. That would imply, for instance, that a combination of a symmetric and asymmetric XH vibrations would occur in the "overtone" region. It should be noted that the longer-wavelength detectors that are sensitive to the combination region are noisier and more expensive (in the case of the multichannel arrays this is quite significant) than the shorter-wavelength detectors that can measure overtones. An important point that should be made is that because of the overall weakness of the absorptions in the NIR, relatively thick materials can be measured, even in transmission.

Instrumentation

The biggest factor in the definition of the instrumentation for each measurement is what is compatible with the wavelength range. For instance, the NIR region is still compatible with glass optics, which means that fiber optics can be used. However, the detectors used in this region of the spectrum are different from those used in the visible and tend to be based on materials like InGaAs (to 1.7 μm) and extended InGaAs (to 2.5 μm), but other materials such as InSb, Ge, and Pb salts have also been used. The light can be analyzed into a spectrum by using either a grating-based dispersive spectrometer or a Fourier-transform (FT) interferometer. When the dispersive instrument is equipped with a multichannel detector, such as an InGaAs array, all wavelengths are detected simultaneously. Such a dispersive instrument with a multichannel detector will benefit from the multiplex or Fellget's advantage, which was originally defined on FT systems.

For mid-IR measurements, FT instruments are now used almost exclusively. Before the 1960s, IR measurements were made with prism-based dispersive systems equipped with single-channel detectors. The development of digital control and then computers of manageable size provided much of the computing power necessary to engineer FT-IR instruments compatible with industrial and university laboratories. The conversion from dispersive to interferometer-based systems provided powerful new capabilities. Compared to a spectrometer with a light-limiting entrance slit, the entrance aperture of an interferometer accepts essentially all of the available energy (Jacquinot advantage). During the acquisition of the interferogram, the detector is "seeing" all of the wavelengths simultaneously so every point in the transformed spectrum reflects the same state (or combination of states) of the sample (Fellget's advantage). To accurately perform the conversion from the interferogram to a spectrum, the instruments use a laser to search for the zero-crossing of the interferogram; this provides superb wavelength tracking, so the instruments are much less susceptible to environmentally caused drift (Connes' advantage). Because of the rapid evolution of FT-IR instruments, their usage has become universal — thousands and even tens of thousands of instruments have been delivered with ever more sampling accessories. A corollary of this is the assembly of rather large IR data bases that have enormous benefits for identification of unknowns.

As will be discussed later, NIR was developed largely for commercially interesting products because of several technical advantages, not the least of which is the use of glass optics. NIR instruments are easier to construct than mid-IR instruments. They can be small (grating-based) spectrometers or small interferometers, either one with a single-channel detector, and they can also be constructed with a multichannel detector (MCD) on a grating-based spectrograph. The downside of the use of NIR spectra is the lower information content in the spectra that is exhibited in broad overlapping bands superimposed on a large scattering background which has to be dealt with in data treatment (this aspect will be discussed in the next section). However, the limitation of low information content has been overcome by the extremely high signal-to-noise in the data that enables sophisticated multivariate analysis to extract composition information from the data sets.

Raman instruments come in essentially two designs — dispersive and Fourier transform. In fact, the earliest instruments built during the 1930s and 1940s were prism-based dispersive systems. In the 1960s grating-based instruments became the rule, with the Toronto mercury lamp source getting replaced by gas lasers that became available. But the universal implementation of Raman equipment was hindered by fluorescence, which was experienced in almost all industrial materials. In 1986, Bruce Chase at Dupont, in collaboration with Tomas Hirschfeld, experimented with Raman detection on the emission port of an FT-IR instrument, using a Nd:YAG laser emitting at 1064 nm (7). The motivation for this effort was that the laser photons have energy low enough that they are unable to excite fluorescence, at least in organic systems. FT-Raman systems were commercialized first as accessories to FT-IR instruments, and then as stand-alone products.

FT-Raman systems became quite popular and reinvigorated interest in Raman spectroscopy by the analytical community. The instruments were continuously improved until they came close to hitting the photon shot-noise limit. During this period, dispersive Raman instrumentation also reinvented itself. Large double monochromators with water-cooled lasers and single-channel detectors were replaced by compact spectrographs with air-cooled lasers and multichannel detectors. Spectral acquisition times for equivalent spectral resolution and signal-to-noise dropped by two orders of magnitude. Red lasers eliminated much of the fluorescence interference seen with green argon laser excitations. In addition, the microscope as a sampling accessory, which had been introduced much earlier in 1972, provided ease of sampling and added fluorescence rejection. Today, these instruments are being used with lasers between 1064 nm and 244 nm for a wide range of applications.

An important advantage of Raman spectroscopy is that the spectrum can include frequencies between 5 cm^-1 and 400 cm^-1 — a region that is inaccessible to routine IR systems. This provides information on metal oxide vibrations and phonon modes in molecular crystals. Metal oxides are of importance to the paint industry, to catalysis, to conservation of cultural heritage, to additives in textiles and paper, and so forth. Molecular phonons are of high importance in the pharmaceutical industry where the crystalline form of the active pharmaceutical ingredient (API) can determine bioavailability.

Vibrational spectroscopy is valuable not only because of the information that it can potentially provide. Sampling is often done in a noncontact mode, sometimes in controlled, isolated environments (such as reactors), and the measurement is nondestructive. Furthermore, in the visible and NIR region of the spectrum, light can penetrate many containers made of glass or plastic so a measurement can be made without breaching the container integrity. On top of this, visible and NIR light can be transmitted via fiber optics to and from a container making remote sampling a reality.

The serious reader can obtain more information on these types of instrumentation in Volume I of Handbook of Vibrational Spectroscopy, edited by John Chalmers and Peter Griffiths (8).

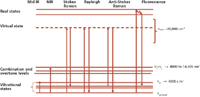
Figure 1: Jablonski diagram illustrating mid-IR absorption, NIR absorption, Raman processes, Rayleigh scattering, and fluorescence.

Spectral Characteristics

To enable the reader to have a feeling for the differences in spectral characteristics of IR, Raman, and NIR spectra, the following figures have been included. Figure 2 shows Raman and IR (corrected attenuated total reflection [ATR]) spectra from the same materials. (Keep in mind that these spectra were not generated from the same samples. The Raman spectra came from our spectral collection, and the FT-IR spectra were generously provided by Pauline Leary of Smiths Detection, Danbury, Connecticut.) The spectra can be overlaid along the x-axis because both are detecting fundamental vibrations. Clearly the spectra are different and the analysis of these differences is beyond what I am hoping to do in this column. The point is to note that the spectral characteristics are different, as well as the sampling possibilities. These are the factors that would determine the choice of the technique for a particular situation.

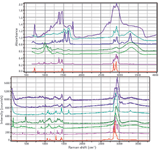
Figure 2: FT-IR (top) and Raman (bottom) spectra of (from top to bottom on each plot) tendon, wool, nylon, starch, cotton, polypropylene, and polyethylene. The FT-IR spectra were generously supplied by Pauline Leary of Smiths Detection.

Figure 3 shows NIR spectra generously supplied by Linda Kidder and Gerald Sando of Malvern Instruments (Columbia, Maryland). Note that most of these spectra span the region between 1400 to 2400 nm which corresponds to 7143 to 4167 cm^-1, or a spectral range of 2976 cm^-1, and can be compared to the IR or Raman region that is about 3500 cm^-1. Because of the limited number of spectral features and the widths of the bands, the spectra are considered to be less information-rich. But because the signal-to-noise ratio of the NIR spectra is so high, and sampling can be done in situ, for industrial applications the spectra are quite useful, especially when taking advantage of electronic data processing, which will be discussed in the next section of this article.

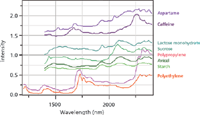
Figure 3: NIR spectra (from top to bottom) of aspartame, caffeine, lactose monohydrate, sucrose, polypropylene, Avicel, starch, and polyethylene. These spectra were kindly provided by Linda Kidder and Gerald Sando of Malvern Instruments.

Data Handling

Because of the almost universal use of IR absorption measurements in analytical laboratories for more than 30 years, the spectroscopy software for these measurements was developed the earliest. It goes without saying that the interferograms need to be converted to absorbance from transmission or reflectance (diffuse, specular, or ATR). These algorithms are now well-developed. Routines for smoothing, taking derivatives, fitting and subtracting baselines, scaling two spectra, subtracting one spectrum from another, band-fitting, and so forth are available in most commercial products.

Raman also requires Fourier transformation if the spectrum is acquired on an FT instrument, but that only applies to FT-Raman instruments that are equipped with 1064-nm Nd:YAG lasers. Dispersive Raman instruments provide spectra directly, although there are extensive algorithms behind the user interface to convert the data into wavenumber space from the CCD pixels. The same treatment functions that are used for IR are applied to Raman spectra — smoothing, derivatization, baseline treatment, spectral scaling and subtraction, band-fitting, and so forth. The Raman spectra, however, tend to be significantly noisier than the FT-IR spectra, and there are often much more intense baselines relative to the spectral bands, so smoothing and baseline correction have to be done with care.

Often spectra are acquired to identify material for a variety of reasons — manufacturing contaminants, incoming product identification, seized illicit drugs, forensics matching, and so on. Identification can be done by spectral matching with a database of spectra of a library of materials that represents the universe of possibilities. Because FT-IR has been a mature measurement technique for so long, the available libraries are quite large and can be easily located. More than half of the compounds are organic in nature. Raman libraries have appeared more recently and are not as extensive, but the situation is improving as time goes on.

NIR spectroscopy was developed largely for commercially interesting products such as foodstuffs, polymers, and pharmaceuticals, in which the optical quality of the sample cannot be controlled. What this means is that there is always a significant broad background under the absorption bands that is a result of light scattering and varies with the particle size. Data treatment of these spectra is absolutely essential to derive any useful information. The following discussion is a description of how these spectra are often treated.

The baseline of NIR spectra can be approximated by a linear background. Performing the first derivative on such spectra will convert a linear baseline to a constant offset in the derivatized spectrum. However, performing a second derivative to the spectrum will eliminate the baseline altogether, and in addition, will enable easy identification of peak positions — a positive peak in the original spectrum appears as a negative peak in the second derivative spectrum. In this format, spectral features have much better definition and intensities can be used to "predict" concentrations. For example, in agricultural products, one might want to know the protein or fat content of a food product such as milk, meat, or cheese. In a pharmaceutical tablet, one might want to know the relative concentrations of the API and the various excipients. Figure 4 illustrates how performing the second derivative defines peaks that are almost not discernable in the original spectra. This, of course, can only be performed effectively because of the extremely high signal-to-noise ratio of the spectra.

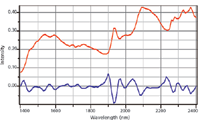
Figure 4: NIR spectrum of lactose monohydrate - original spectrum (top) vs. second derivative of original spectrum (bottom), multiplied by a factor of 10 for comparison purposes. These spectra were kindly provided by Linda Kidder and Gerald Sando of Malvern Instruments.

Summary

Vibrational spectroscopies (FT-IR, Raman, and NIR) are being used for numerous analytical applications. Each application can be best managed by a selected technique, or in some cases a combination of techniques. If remote measurements, or measurements inside a container are required, then the choice would be NIR or Raman. If identification of an unknown is required, the choice would first be IR and then Raman. If a sample is very highly fluorescent, Raman, even with contemporary solutions to avoid much fluorescence, might not be the best choice, so one could use FT-IR. An aqueous solution would be difficult for IR analysis, unless the use of ATR sampling is possible, but because the Raman signal of water is weak, it provides an ideal solvent for Raman studies of the solute. If you have a problem to solve, and think that one of the analytical vibrational spectroscopies will work, it would be best to consider these effects, and perhaps consult with someone experienced in the field.

Acknowledgment

To give credit where it is due, I want to say that the idea for this column came from one of my colleagues, who pointed out to me that there is confusion among people in need of one of these techniques and they are unable to make an informed decision about what to use because of a lack of knowledge.

Fran Adar is the Worldwide Raman Applications Manager for Horiba Jobin Yvon (Edison, New Jersey). She can be reached by e-mail at fran.adar@horiba.com.

Fran Adar

References

(1) N. Sheppard, "The Historical Development of Experimental Techniques" Handbook of Vibrational Spectroscopy, Volume 1, J.M. Chalmers and P.R. Griffiths, Eds. (John Wiley & Sons, Hoboken, New Jersey, 2002).

(2) J. Workman, An Introduction to Near Infrared Spectroscopy, www.spectroscopynow.com.

(3) R.F. Goddu and D.A. Delker, Anal. Chem. 32, 140–141 (1960).

(4) C.V. Raman, Nature 121, 501 (1928).

(5) C.V. Raman, Ind. J. Physics 2, 387 (1928).

(6) A. Smekal, Naturwissenschafter 11, 873 (1923).

(7) T. Hirschfeld and D.B. Chase, Appl. Spectrosc. 40(2), 133–134 (1986).

(8) Sections: "Instrumentation for Mid- and Far-infrared Spectroscopy," "Instrumentation for Near-infrared Spectroscopy," and "Instrumentation for Raman Spectroscopy" in Handbook of Vibrational Spectroscopy, Volume 1, John M. Chalmers and Peter R. Griffiths, Eds. (John Wiley & Sons, Hoboken, New Jersey, 2002).