The Correlation Method for the Determination of Spectroscopically Active Vibrational Modes in Crystals

Page 17–22

The application of vibrational selection rules is usually taught with respect to the vibrational motions of individual molecules. However, many of the materials studied by infrared or Raman spectroscopy are solids and even single crystals. Furthermore, some materials such as covalent or ionic solids have no molecular species associated with them. Consequently, there is a need to understand the application of group theory for the determination of spectroscopically active vibrational modes of crystals. The correlation method is ideal for that purpose.

The determination of spectroscopically active vibrational modes (Raman or infrared [IR]) is generally taught through the use of individual molecules in conjunction with group theory (1). Most spectroscopists have been taught at some time about the identification of point groups for a molecule and the use of character tables in conjunction with selection rules for the determination of Raman and IR active vibrational modes of molecules. That works fine when dealing with gases or liquids. However, very often we deal with solid-state materials for which there may be no molecular species-for example, TiO₂, Si, C (graphene or diamond), or CaCO₃. How then should we go about determining the Raman- or IR-active vibrational modes of such materials? This is where factor group analysis comes into play. If instead of a molecule, we consider a unit cell and the atoms located at specific sites therein, the factor group consists of those symmetry elements and corresponding symmetry operations that when applied to the unit cell leave it indistinguishable from its original position. I think you can see how the factor groups of the 32 crystal classes correspond to the isomorphic point groups. Consequently, the same character tables of point groups used to determine the vibrational modes of molecules are also used to determine the Raman- and IR-active lattice vibrational modes of crystals (2,3).

The procedures for determining the Raman- and IR-active modes of crystals were first published many decades ago (4–7). Subsequently, several helpful books were published on these methods teaching spectroscopists to apply selection rules and factor group analysis to determine the Raman- and IR-active vibrational modes of crystals whose space groups were known along with the number of formula units per unit cell (8–10). I highly recommend the books by Ferraro and Ziomek (9) and Fateley and colleagues (10). The Fateley book works through many examples through which the authors teach the correlation method established by Halford and Hornig. I have used one example and the style of teaching the correlation method from the Fateley book for this installment.

Space-Group Identification and Crystallographic Information

The first step in determining the spectroscopically active vibrational modes of a crystal is to identify the Bravais space cell. Many publications and crystallographic tables will list the number of molecules or formula units per crystallographic unit cell (Z). However, the correlation method requires knowledge of the number of molecules or formula units in the primitive or Bravais cell (Z^B), which is equal to Z divided by the number of lattice points (LP) determined by the designation of the space group (11). Examples of crystals with different numbers of lattice points are shown in Table I.

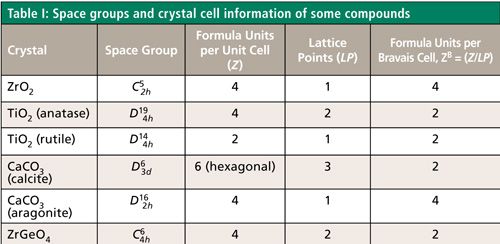
We will use TiO₂ in the anatase crystal form as our first example for determining the vibrational modes. This crystal form belongs to space group 
D¹⁹_4h(I_41/amd) with four formula units and two lattice points per crystallographic unit cell. Therefore, there are two formula units per Bravais cell (Z^B = 2). Having identified the space group of a crystalline compound, the next step is to identify the site symmetry of equivalent atoms in the Bravais cell. The site symmetry for equivalent atoms is a subgroup of the factor group describing the Bravais cell. The site symmetries for all of the space groups have been identified and tabulated (12), and that for D¹⁹_4h is shown in Table II.

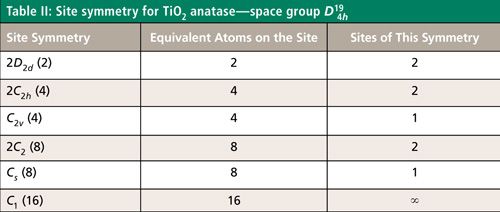
We know from Table I that anatase TiO₂ has two formula units per Bravais cell (Z^B = 2), two Ti atoms, and four O atoms in the Bravais cell. From Table II, we see that the only site that accommodates two equivalent atoms is D_2d, which then must be the site symmetry of the two Ti atoms. Regarding the O atoms, the C_2h and C_2v sites both accommodate four equivalent atoms, and we must therefore choose between these two site symmetries. To choose correctly between our two options we must consult crystallographic tables to identify the proper Wyckoff site and consequently the site symmetry of the O atoms. We don’t have the space here to explain the procedure for using the tables listing Wyckoff sites, so we simply state that the site position of the O atom in anatase TiO₂ corresponds to the site symmetry C_2v. Those readers interested in the procedure of using the crystallographic tables to identify Wyckoff sites should consult chapter 2 of the book by Fateley and colleagues (9).

The Correlation Method-Site Symmetry to Factor Group

To make the correlation from site species to factor group species and the determination of the lattice vibrational modes, we need to understand the relationship between the translational and rotational degrees of freedom and site and factor group symmetry species. The number of translations in the site species γ is defined as t^γ and the number of rotations for the site species is R^γ. The degrees of freedom can take on the values of 0, 1, 2, and 3 depending upon the degeneracy and symmetry species; the values are taken directly from the x, y, and z column of the character table. The degrees of vibrational freedom for a given site species γ is given by the symbol f^γ and is related to the number of translations in that same species by the following equation:

f^γ = t^γ ∙ n [1]

where n is the number of equivalent atoms in the formula unit. Likewise, the degrees of rotational freedom f^γ_R is given by

f^γ_R = R^γ ∙ n [2]

The contribution of degrees of freedom by each site species γ to a factor group species ζ is given by the symbol a_γ, and its relationship to the vibrational degrees of freedom is given by the following expression:

f^γ = a_γΣC_ζ [3]

where C_ζ is the degeneracy of the factor group species ζ. The values of C_ζ are equal to the degeneracies of the symmetry species; that is, 1 for the A and B species, 2 for the doubly degenerate E species, and 3 for the triply degenerate T species.

Spectroscopists often learn group theory for the purpose of determining the spectroscopically active vibrational modes of a molecule. Similarly, our goal here is to determine the irreducible representation of the symmetry species of crystal lattice vibrations. To do that, we need to correlate the site symmetry species of the equivalent sets of atoms to the factor group of the crystal. The irreducible representation of an equivalent set of atoms is given by

Γ_{eqiv atoms} =Σa_ζ ∙ ζ [4]

where a_ζ equals the number of lattice vibrations of species ζ of the crystal factor group. Summing all of the irreducible representations of the equivalent atoms yields the total irreducible representation of the crystal.

Γ_crystal = Γ_{equiv atoms} 1 + Γ_{equiv atoms} 2 + …  [5]

The irreducible representation of the crystal contains within it the so-called optical and acoustical vibrations or phonons. Therefore, we will have to subtract the irreducible representation of the acoustical phonons from the irreducible representation of the crystal to achieve our final goal of determining the irreducible representation of the optical lattice vibrations:

Γ_vib = Γ_crystal - Γ_acoustic [6]

To determine Γ_crystal we need to correlate the site symmetries of the equivalent atoms in the lattice to the factor group of the crystal. We have identified in the previous section the site symmetries of the Ti and O atoms in the TiO₂ anatase crystal. Next, we will treat the displacements of each atom on those sites, correlate the site species to the species of the factor group, and identify the lattice vibrations of the crystal. The procedure for determining the lattice vibrations is to work with one element in the chemical formula at a time. The site symmetry of the Ti atoms is D_2d and so we turn to the D_2d character table shown in Table III to identify those symmetry species associated with displacements along x, y, and z axes. The D_2d site species that involve vibrations along the x, y, and z directions are B₂ and E. Our next step is to correlate the site species to the factor group species from which we can determine the symmetry species of spectroscopically active vibrational modes.

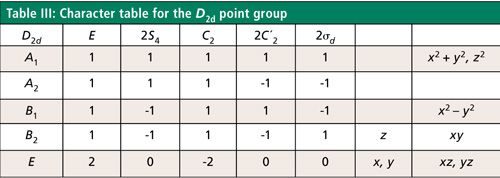
Factor Group Analysis of Anatase TiO₂
We begin our factor group analysis by considering the Ti atoms on site D_2d. We know from Table II that there are two equivalent Ti atoms (n = 2) on site D_2d and from Table III that the symmetry species of translation are B₂ and E. Thus, the vibrational degrees of freedom of the two equivalent Ti atoms are 2 for B₂ and 4 for E. For clarity, these results and their relationship are summarized in Table IV. Next, we want to correlate the site group species of the atom to the corresponding factor group species of the crystal, which is D_4h for anatase TiO₂ as indicated in Table I. To do this it is necessary to consult published correlation tables that can be found in several of the references cited here (9,10,13). A complete correlation of the D_2d site group species to the D_4h factor group species is shown in Figure 1. However, we see from Table IV that the only the B₂ and E site species have nonzero vibrational degrees of freedom. Therefore, we need only consider the correlation of those two site group species to the factor group species when determining the vibrations of the Ti atoms in the anatase TiO₂ crystal.

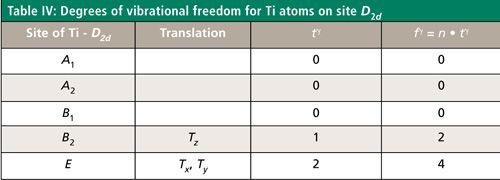
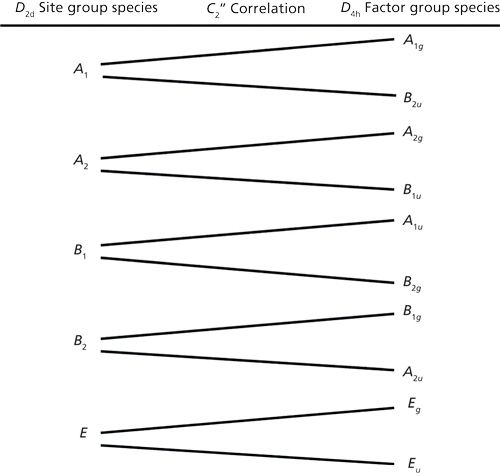
Figure 1: Correlation of the D_2d site group species to the D_4h factor group species.

Figure 2: Correlation of the site group species D_2d to the D_4h factor group species for the determination of the vibrational modes involving the Ti atoms in the anatase TiO₂ crystal lattice.

Figure 2 shows the correlation of the site group species D_2d to the D_4h factor group species for the determination of the vibrational modes involving the Ti atoms in the anatase TiO₂ crystal lattice. Examine carefully the values shown in the last three columns of this figure and you will see that a_ζ is equal to the sum of the number of the site species contributing to the number of factor group species:

a_ζ = Σ_γa_γ [7]

We have several ways of checking the accuracy of Figure 2 and confirming our work. The total degrees of freedom of our equivalent set of two Ti atoms is 3n = 6. The sum of the degrees of vibrational freedom (column 1, f^γ) equals 6. Also, the sum of the number of factor group species times the degeneracy of the species (Σ_ζa_ζC_ζ) equals 6; that is the sum of the products in columns a_ζ and C_ζ equals 6. So our check of the degrees of vibrational freedom of the Ti atoms confirms our work and correctness of the values in Figure 2.

Next, we generate the irreducible representation of the symmetry species associated with the vibrations of the Ti atoms. Note that in Figure 2 the a_ζ value for each D_4hfactor group species is 1. Therefore, our D_4h factor group contribution from the Ti atoms to the anatase TiO₂ crystal is

Γ_Ti = B_1g + A_2u + E_g + E_u [8]

Now that we have determined the factor group species associated with the vibration of Ti atoms, we must complete our work by performing the same procedure for the O atoms. Referring back to Table II and the discussion regarding the selection of site symmetries, we see that the site group symmetry of the O atoms in anatase TiO₂ is C_2v. Therefore, our correlation table should relate the site group species C_2v to the D_4h factor group species for the determination of the vibrational modes involving the O atoms in the anatase TiO₂ crystal lattice. The procedure for the correlation of the O atom site group species to the factor group species is the same as that for the Ti atoms and we show the results in Figure 3. Note that for the O atoms not all of the a_ζ values are nonzero and that for the doubly degenerate E species the value is 2. The a_ζ values provide the corresponding coefficients for the individual factor group species forming the irreducible representation of the O atoms.

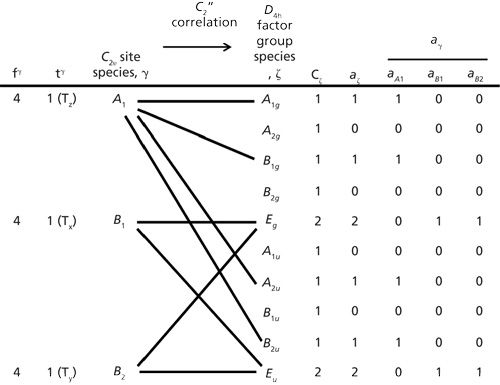
Figure 3: Correlation of the site group species C_2v to the D_4h factor group species for the determination of the vibrational modes involving the O atoms in the anatase TiO₂ crystal lattice.

We should check our work as we did previously for the Ti atoms. The total degrees of freedom of our equivalent set of four O atoms is 3n = 12. The sum of the degrees of vibrational freedom (column 1, f^γ) equals 12. Also, the sum of the number of factor group species times the degeneracy of the species (Σ_ζa_ζC_ζ) equals 12; that is the sum of the products in columns a_ζ and C_ζ equals 12. So our check of the degrees of vibrational freedom of the O atoms confirms our work and the correctness of the values in Figure 3.

Next, we generate the irreducible representation of the symmetry species associated with the vibrations of the O atoms. Note that in Figure 3 not all of the a_ζ values corresponding to the individual D_4h factor group species are nonzero. Therefore, our D_4h factor group contribution from the O atoms to the anatase TiO₂ crystal is constructed from only those species with nonzero values in Figure 3.

Γ_O = A_1g+ B_1g + 2E_g + A_2u + B_2u + 2E_u  [9]

Recall from equation 5 that the sum of all of the irreducible representations of the equivalent atoms yields the total irreducible representation of the crystal.

Γ_{TiO2(anatase)} = Γ_Ti + Γ_O = A_1g + 2A_2u + 2B_1g + B_2u + 3E_g + 3E_u  [10]

However, we are not quite done yet. The irreducible representation of the TiO₂ anatase crystal contains within it the so-called optical and acoustical vibrations or phonons. Therefore, we still have to subtract the irreducible representation of the acoustical phonons from equation 10 to achieve our final goal of determining the irreducible representation of the optical lattice vibrations. The acoustical modes have the same character as the translations in the factor group’s character table; here D_4h. Consulting the D_4h character table shown in Table V we find that the X and Y translations correspond to the E_u species and the Z translation corresponds to the A_2u species.

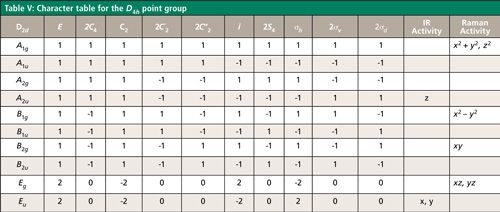
Therefore,

Γ_acoust = A_2u + E_u   [11]

Subtraction of the acoustical phonons from Γ_{TiO2(anatase)} will, according to equation 6, will give us the irreducible representation of the optical lattice vibrations of anatase TiO₂.

Γ_vib = A_1g + 2A_2u + 2B_1g + B_2u + 3E_g + 3E_u  - (A_2u + E_u ) = A_1g + A_2u + 2B_1g + B_2u + 3E_g + 2E_u  [12]

and

Γ_vib = A_1g (Raman) + A_2u  (IR) + 2B_1g (Raman) + B_2u (inactive) + 3E_g (Raman) + 2E_u (IR) [13]

Therefore, a Raman spectrum of anatase TiO₂ should consist of one A_1g, two B_1g, and three E_g bands, whereas the IR absorption spectrum should consist of one A_2u and two E_u bands. The B_2u vibrational mode is silent.

It is important to realize that the exercise that we have completed has provided us with the irreducible representation of the vibrational modes of only the anatase form of TiO₂; that is, the representation does not apply to the other crystalline forms such as rutile or brookite. For example, here is the irreducible representation of the vibrational modes of rutile TiO₂.

Γ_{TiO2 rutile} = A_1g (Raman) + A_2g (inactive) + A_2u (IR) + B_1g  (Raman) + B_1u (inactive) + B_2g (Raman) + E_g (Raman) + 3 E_u (IR) [14]

A comparison of the anatase and rutile irreducible representations in Table VI reveals that the Raman and IR spectra should allow us to differentiate them by the number of bands and their symmetries.

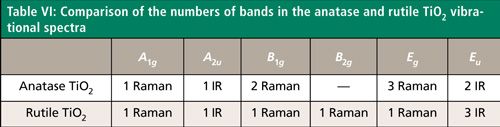
The work that we have just done has hopefully demonstrated to you the importance and distinction between the determination of spectroscopically active vibrational modes of crystalline solids and the more familiar treatment of isolated molecules. The spectroscopist must appreciate the dependence of IR absorption and Raman scattering on the material’s crystal structure. Furthermore, a working knowledge of the selection rules and the correlation method allows the vibrational spectroscopist to use IR absorption and Raman spectroscopy to differentiate crystal forms in a fashion complementary to X-ray diffraction.

Conclusions

The correlation method for the determination of spectroscopically active vibrational modes in crystals has been presented. The correlation of the site symmetry of equivalent atoms in the chemical formula to the factor group of the crystal allows one to build the irreducible representation of the vibrational modes of the crystal one element at a time. The method has within itself checks on the correctness of the determinations based upon the degrees of freedom of the equivalent atoms and the vibrational degrees of freedom. The spectroscopist can use vibrational spectroscopy in conjunction with the correlation method to differentiate different crystalline forms of the same chemical compound.

References

• D. Tuschel, Spectroscopy29, 14–23 (2014).

• J.R. Ferraro, Appl. Spec.29, 418–421 (1975).

• D.L. Roussequ, R.P. Bauman, and S.P.S. Porto, J. Raman Spec. 253–290 (1981).