The Fundamentals of Total Reflection X-ray Fluorescence

Total reflection X-ray fluorescence (TXRF)spectroscopy is one of the most impressive analytical techniques, and provides spectral signatures of materials that can be used to unravel their elemental composition. Within a few seconds, and with minute sample amounts and limited sample preparation, a first characterization of qualitative character can be obtained, where previouslythorough and quantitative information would require a full experimental design approach. TXRF extends the capabilities of XRF down to the ultratrace elemental range, with detection limits reaching the femtogram levels. However, given that TXRF is a highly sensitive technique, variations between the samples or the analysis conditions may cause very slight spectral differences that may be difficult to distinguish or identify. Therefore, and despite its apparent versatility and ease of use, many issues remain in TXRF analysis, and its application requires knowledge and care. A network (1) was set up for training and expanding the user community, as well as tackling the challenges of the technique. Here, we provide a tutorial on the techniques and key steps that any user, and particularly new users, should follow.

Know the Fundamentals of the Technique

TXRF is a surface sensitive multielement microanalysis technique, based on the principle that a collimated X-ray beam is totally reflected when striking a flat, highly polished, and reflective surface material at an incident angle below the critical angle of the substrate (2–5). The fundamentals of TXRF are based on Fresnel formalism and on the complex index of refraction for X-rays (n =1 -δ - iβ, with δ the decrement of the refractive index, and β the absorption term). Considering absorption negligible, the critical angle αcrit (deg.), can be calculated as follows:

[1]

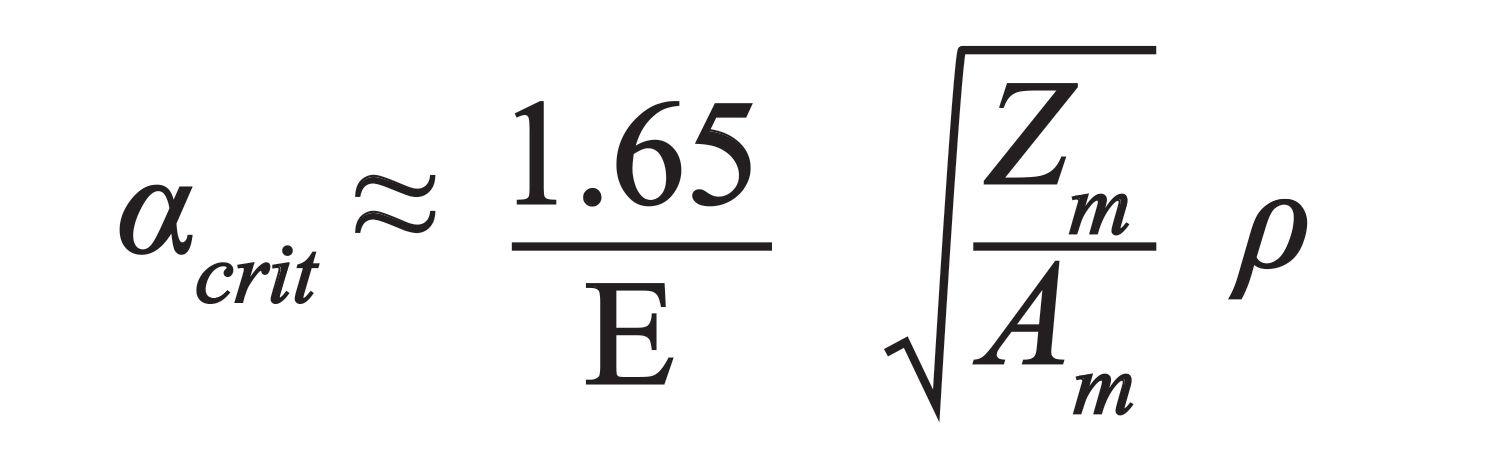
where E refers to incoming photon energy (keV), Zm is the atomic number, Am is the atomic weight (g/mol), and ƿ is the substrate density (g/cm3) of the substrate.

A complex triangular volume of X-ray interferences dominated by waves is created by the superposition of the incoming and refracted beam, the intensity of which varies according to the distance above the surface (Figure 1). This standing-wave field is composed of an alternated node and antinode pattern whose period is a function of the incident angle and photon energy. The period (∝E/sinα) is typically only several tens of nanometers, while the height of the standing-wave triangular volume can reach several micrometers. For an ideal 100% reflective surface, and angles below the critical angle, the intensity of the standing-wave lies alternatively between zero (nodes) and four times (antinodes) the intensity of the incident photon energy. But, even for highly reflective materials, some photons penetrate the substrate, the intensity of which varies radically with the angle of incidence. The penetration depth is defined as the depth at which the intensity of the primary radiation is reduced to 1/e (about 37%), and its value remains low (almost constant) below the critical angle. Working below αcrit thus favors low scattered contributions to the background from the substrate, which, in turn, directly improves the low detection limits and high sensitivity and detection power of TXRF.

Figure 1: TXRF instrumental schematic and angular dependency of the fluorescence emission for three sample types.

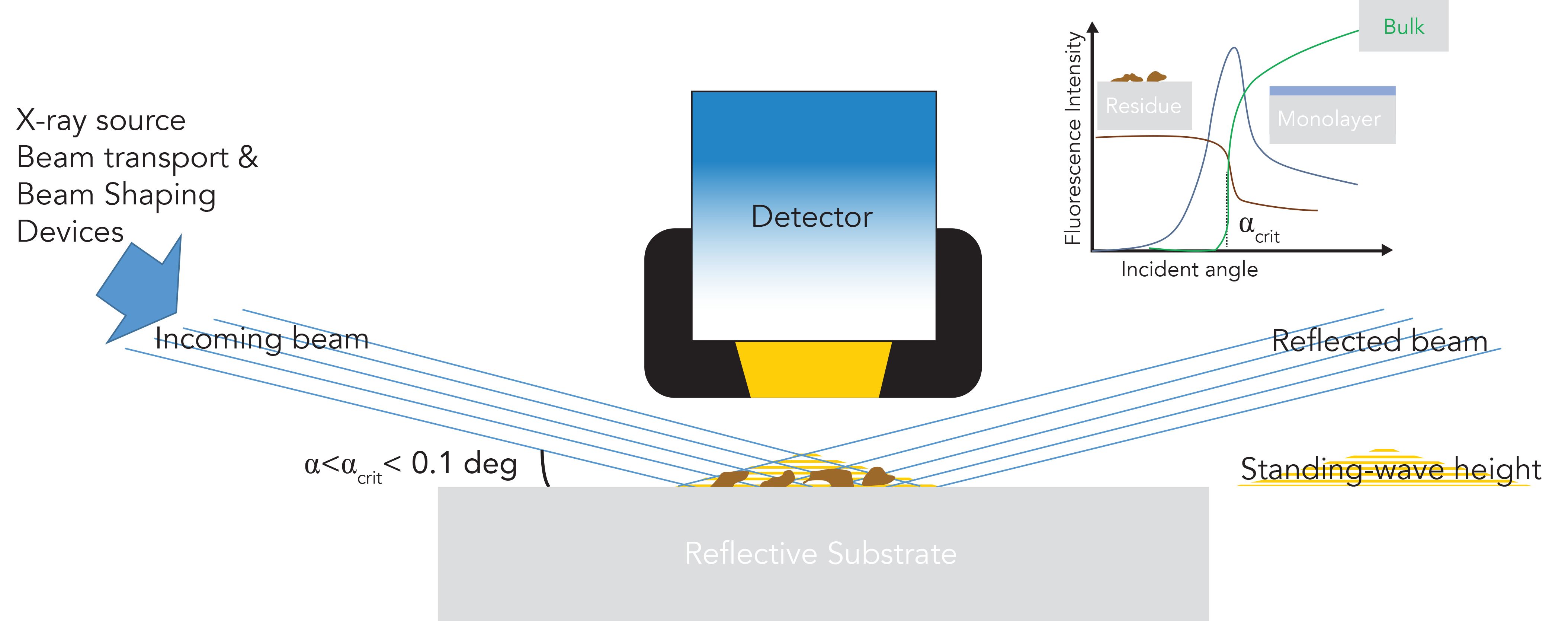
Any thin sample placed on the substrate within the standing-wave field will be irradiated by both the incident and the reflected beam as the incident radiation experiences total reflection at the substrate interface. A thin solid residue (from a droplet, or powder, a few 100 nm thick) will be subjected to a great number of standing waves, and its fluorescence emission intensity on average will be doubled, whereas a thin monolayer or layered structure (<100 nm thick) will experience standing waves arising above and within its structure, and the fluorescence intensity will vary, according to the layer position within nodes and antinodes. Indeed, the intensity of the fluorescence radiation emitted by the elements present on or at the substrate surface is directly proportional to the intensity of the standing-wave field, and, thus, of the primary photon energy intensity.

Do:

Calculate your critical angle, and check the penetration depth for your experimental conditions.

Know Your Instrument, and Check its Performance

A number of instruments are available to the TXRF community, ranging from different types of commercial TXRF spectrometers (such as benchtop or portable), to custom-made instruments in laboratories or synchrotron facilities (4,8). The main components are the X-ray source, the beam guidance (for example, monochromator, apertures, filters), the reflector sample stage (with or without angle scan option), and the energy dispersive X-ray fluorescence detector (for example, silicon drift diode and germanium). All these parts can be carefully selected to optimize the instrumental conditions of analysis, according to the nature of the sample and
characterization needs.

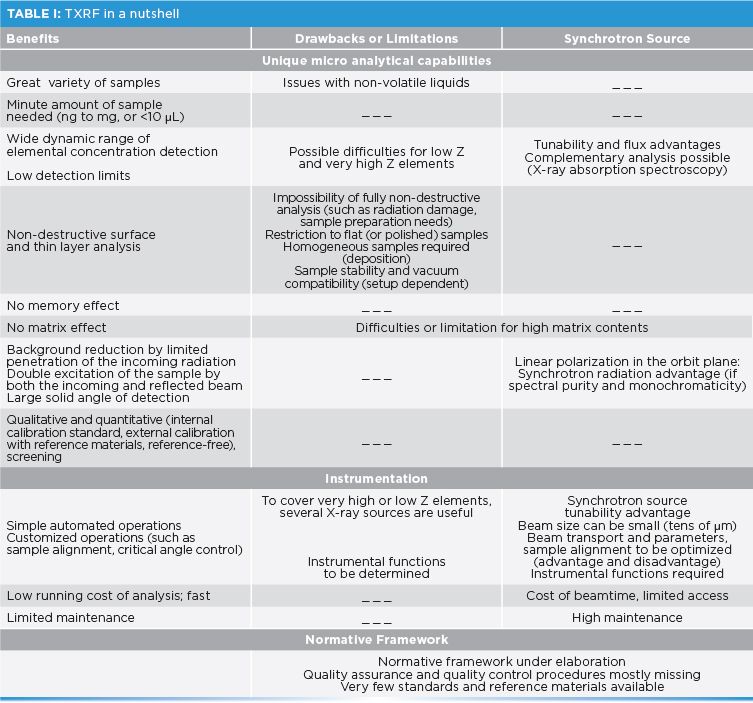
The X-ray source choice is crucial to ensure successful excitation of the elements of interest. Indeed, the efficiency of the excitation decreases for elements whose absorption edges are away from the primary photon energy. Laboratory X-ray sources like molybdenum (17.4 keV) are favored, but if lower Z elements are to be assessed, chromium (5.4 keV) or a plasma source should be considered. To ensure low background, a high level of monochromaticity, spectral purity, low divergence, and flux are required. Additionally, the beam needs to be shaped in size, ideally to illuminate a 1 mm2 area (for example, 1 mm by 40 μm) on the sample. Using a synchrotron as the X-ray source presents flux, a tunable energy range (0.078–90 keV), spectral purity, collimation, and linear polarization, thus providing excitation and detection advantages (Table I). Lastly, the detector, placed at 90 o above the sample surface, must have its solid angle maximized for effective collection of the emitted radiation, by getting close to the sample (a few mm or less) or using a large sensor area (30 to 100 mm2). The type or size of detector and detector windows will determine the spectral resolution obtained from the data, and the sensitivity to the light elements.

Do:

• Check the stability of your X-ray source flux, size, position, and
  spectral characteristics.
• Check the repeatability of the substrate positioning for the critical
  angle considered.
• If adjustable, properly select your critical angle with respect to the angular scan shape obtained. This also ensures that your sample complies with TXRF conditions. If not adjustable, use a reflector substrate that best matches your beam incoming angle.
• Check the response function of your detector for the energy employed, and your detector stability.
• If not fixed, check the rotation axis for your source–substrate–detector system.
• Use blank and certified reference materials to perform quality control of your setup.
• Establish or follow standard operating procedures.

Know and Prepare Your Sample

A key aspect for reliable elemental analysis is knowing the sample under investigation. A plethora of samples can be investigated with TXRF, coming from many different fields of research (Table II). As a consequence, the sample can present itself under various physical and chemical states, most of them allowing for elemental content determination either directly (such as liquids or fine powders) or after some sample preparation steps (4–7,8) to transfer or optimize them into liquid form. Many procedures, including grinding, suspension, dilution, digestion, and preconcentration,
have been proposed.

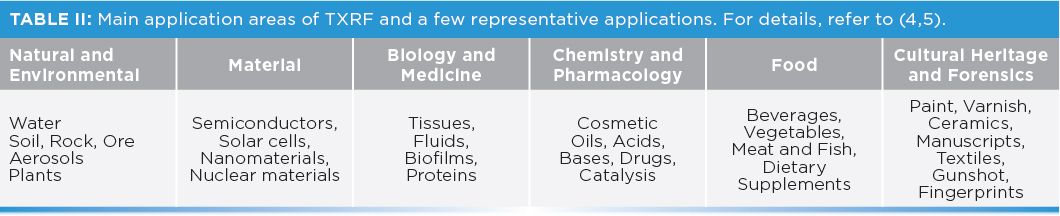
Do:

• Carefully select your reflector substrate. Use a flat (<ʎ/20), smooth (<1 nm roughness), pure, and surface-clean material. Quartz reflectors allow for the best results.
• Use only reagents of ultrahigh purity grade and that will not modify the elemental composition of your sample.
• Carefully select your internal calibration standard. It should not present any spectral interference at any position of the emission spectra, its emission lines should frame the elemental range of analysis, and its concentration should lie within the average sample concentration range. Also, add it prior to any preparation steps.
• Evaluate the total sample mass deposition placed onto the reflector. It should not exceed the microgram level, and is highly matrix-dependent.
• Ensure that the final deposit of sample or residue is homogeneous, thin (a few 100 nm thick), and entirely contained in the surface so as to be illuminated by the incoming beam.
• Always at least duplicate your sample.
• Thoroughly check the recovery rates obtained for the elements
  of interest.
• For each type of sample, optimize and revise the sample preparation procedures.

Define Your Experimental Procedures

Building a consistent system via standard operating procedures (9) and experimental design ensures the quality and reliability of the results obtained.

Do:

• Select carefully your excitation energy.
• Run blank or certified reference materials (Figure 2) to check your instrument, and eventually derive the instrumental parameters, if not determined.

Figure 2: TXRF spectrum of a multielement reference material (ICP L-IV, 3 mg/L) on a quartz reflector; E = 12 keV at the Elettra–XRF beamline, 60 s integration time, αTXRF: 0.19.

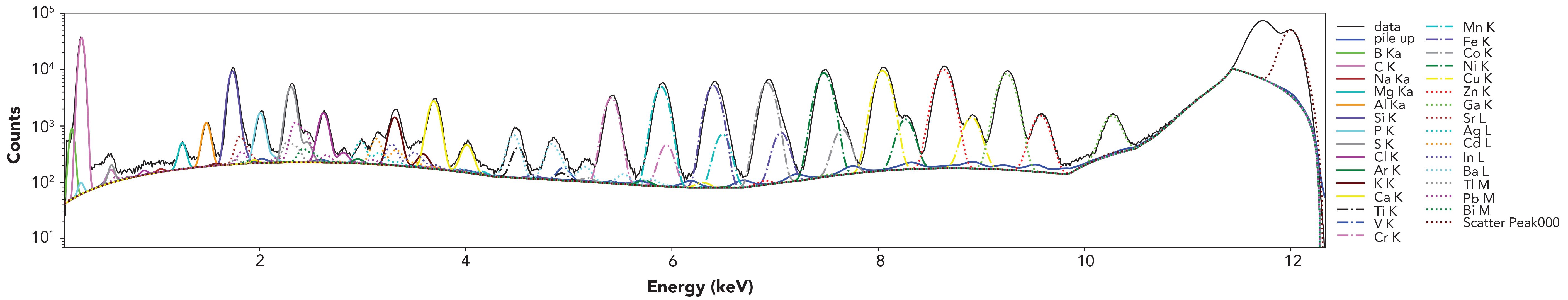
• Optimize the counting time to improve the signal-to-noise ratio (100 s to 1 h, depending on the elemental concentrations targeted), and maintain a low detector dead time.
• For assessing light elements (Z < 20), work under vacuum (this is also efficient to improve the background and the detection in general), with low excitation photon energy, and use a germanium detector, or an silicon drift detector (SDD) without windows, or with thin polymer windows.
• Repeat your measurements.
• Calculate your total budget uncertainty (comprising sample preparation, and instrumental and data analysis).
• Critically assess your data, and compare them with other techniques, taking into account the analytics’ inherent strengths and drawbacks.
  

Know the Quantification Procedures

Calibration of the spectrometer is a prerequisite to any quantitative analysis, and provides relative sensitivity values (S) of all the elements targeted versus a reference element for specific measurement conditions. It uses multi-element reference materials of various concentrations to build a linear relationship of S to atomic number Z, by measuring the net intensity or area (N) of an elemental peak and its concentration (C). These sensitivities can be retrieved by calculations using fundamental data tables, if all instrumental parameters are perfectly known.

The quantification procedure can be undertaken in one of three ways. The first is the use of an internal calibration standard of known concentration (Cis); the unknown elemental concentrations Cx are then calculated as:

[2]

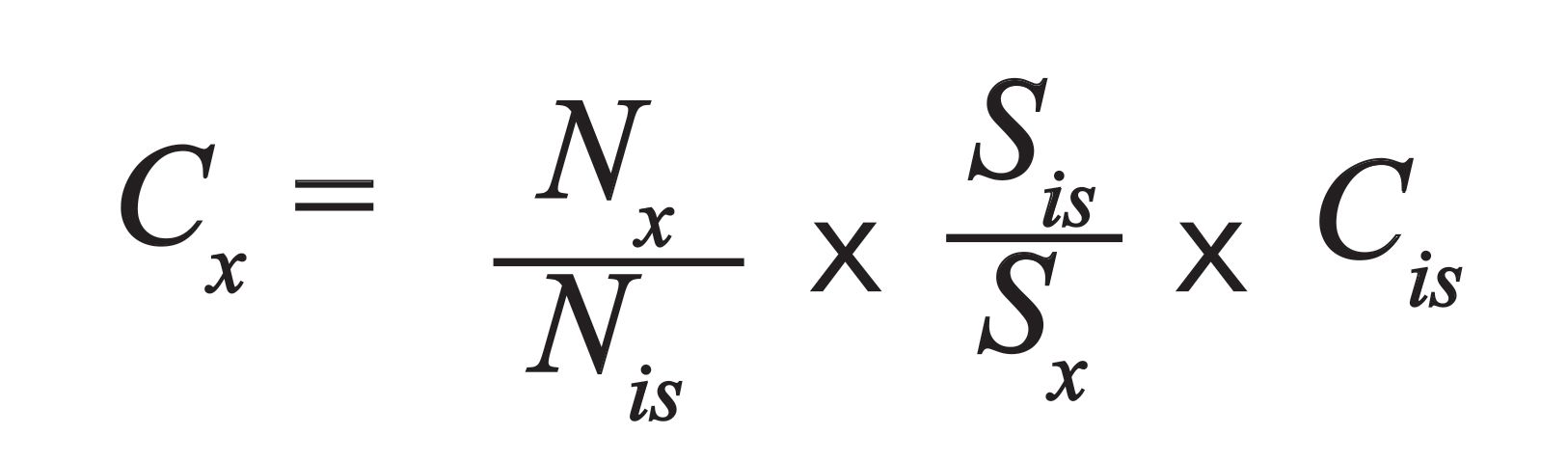
The second is the use of external calibration with reference materials close to the sample itself, and with graduated concentrations of the unknown elements. The third is reference-free quantification, which requires perfect knowledge of the instrumental set up parameters (such as source and detectors), and the use of the fundamental
parameters approach.

Do:

• Build your quantification strategy carefully.
• Supervise your peak deconvolution and fitting algorithms procedure.
• Assess the various elemental detection limits of your setup with respect to minimum mass deposition, which is background dependent. According to IUPAC rules:

[3]

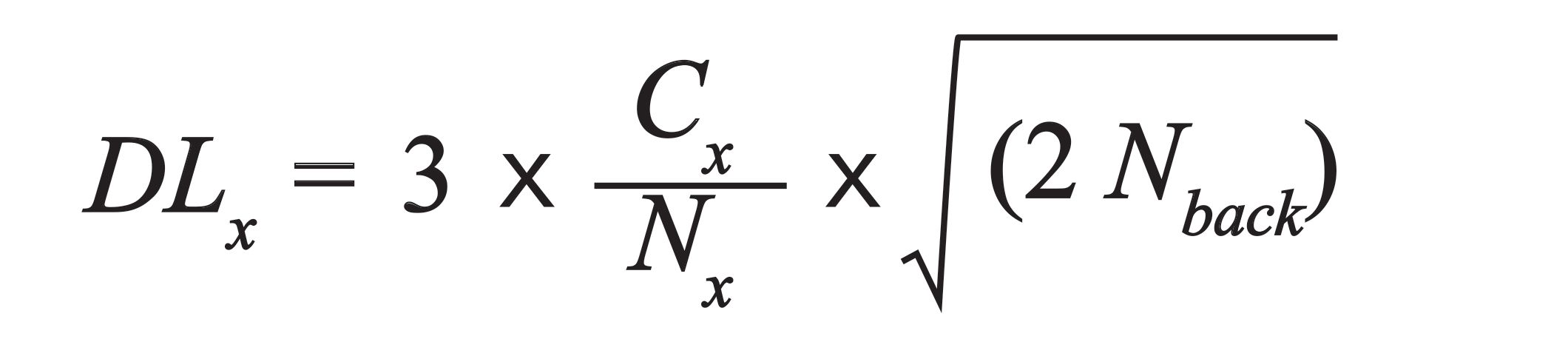
where Nback is the net background intensity or area below the element’s net area of interest.

References

1. www.enforcetxrf.eu
2. Y. Yoneda and T. Horiuchi, Rev. Sci. Instrum. 42(7), 1069–1070 (1971).
3. P. Wobrauschek and H. Aiginger, Anal. Chem. 47(6), 852–855 (1975).
4. R. Klockenkamper and A. Von Bohlen, Total-Reflection X-ray Fluorescence Analysis and Related Methods (Wiley and Sons, Hoboken, New Jersey, 2nd Edition, 2015).
5. M. Schmeling, Phys. Sci. Rev. 4(7), 20170161-23 (2019).
6. I. De La Calle, N. Cabaleiro, V. Romero, I. Lavilla, and C. Bendicho, Spectrochim. Acta B 90, 23–54 (2013).
7. E. Margui, I. Queralt, R. Van Grieken, in Encyclopedia of Analytical Chemistry, R.A. Meyers, Ed. (John Wiley & Sons, Ltd., Chichester, United Kingdom, 2016), pp. 1–26.
8. J. Lubeck, B. Beckhoff, R. Fliegauf, I. Holfelder, P. Hoenicke, M. Mueller, B. Pollakowski, F. Reinhardt, and J. Weser, Rev. Sci. Instrum. 84(4), 045106 1–7 (2013).
9. L. Borgese, R. Dalipi, A. Riboldi, f. Bilo, A. Zacco, S. Federici, M. Bettinelli, E. Bontempi, and L.E. Depero, Talanta 181, 165–171 (2018).

Diane Eichert is with Elettra–Sincrotrone Trieste in Trieste, Italy. Direct correspondence to diane.eichert@elettra.eu